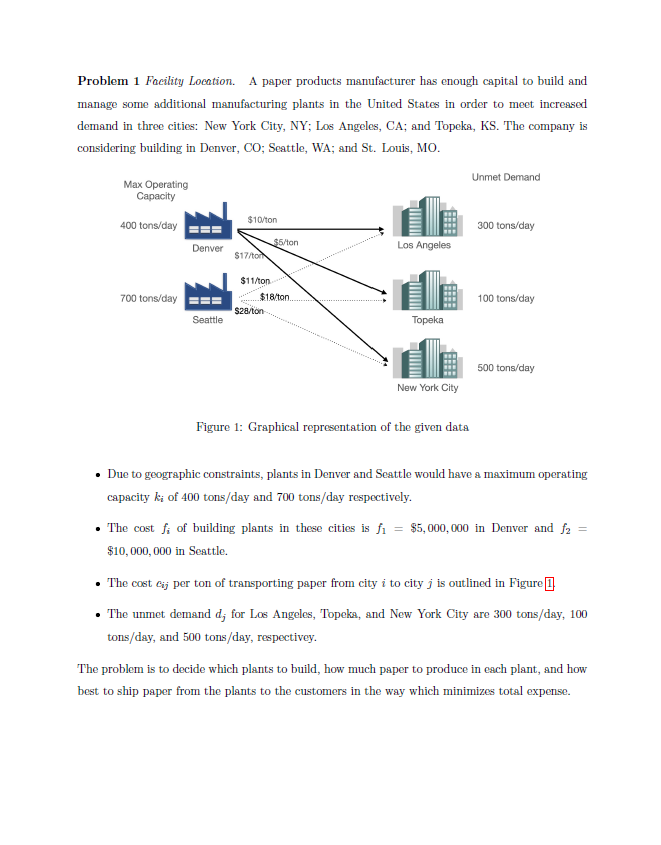




Problem 1 Facility Location. A paper products manufacturer has enough capital to build and manage some additional manufacturing plants in the United States in order to meet increased demand in three cities: New York City, NY; Los Angeles, CA; and Topeka, KS. The company is considering building in Denver, CO; Seattle, WA; and St. Louis, MO. Figure 1: Graphical representation of the given data - Due to geographic constraints, plants in Denver and Seattle would have a maximum operating capacity ki of 400 tons/day and 700 tons/day respectively. - The cost fi of building plants in these cities is f1=$5,000,000 in Denver and f2= $10,000,000 in Seattle. - The cost cij per ton of transporting paper from city i to city j is outlined in Figure 1 . - The unmet demand dj for Los Angeles, Topeks, and New York City are 300 tons/day, 100 tons/day, and 500 tons/day, respectivey. The problem is to decide which plants to build, how much paper to produce in each plant, and how best to ship paper from the plants to the customers in the way which minimizes total expense. (a) Model this problem as an Integer Program. Let wi represent the binary choice of whether or not to build plant i and xij represent the amount of paper to be sent from plant i to customer j for i{1,2} and j{1,2,3}. For this example of the problem, it would be safe to assume that both plants need to be built since nelther can meet all the demand on its own. Please do not make this assumption. Doing so will remove the integer variables which we want to include. (b) Ignoring any integer constraints, derive the dual of the IP you found in part (a). Hint: remember that a binary constraint decomposes into 0,1, and Integer. (c) The first step of solving an Integer Program is to solve its Continuous Relaxation. This is the linear program formed by simply ignoring the integer constraints in the original IP. Solve the IP from part (a), its continuous relaxation, and its dual in Excel. Report the optimal objective function values in your written submission and upload the excel sheets in a workbook under the name YourSurname_Final_solver.xlsx. (d) Recall that the dual of a minimization LP is a lower bound on said LP. That is, there does not exist a solution to the primal with OFV less than the optimal OFV of the dual. Even if we cannot (with our current knowledge) prove that the excel solution to our IP is optimal, we certainly know that there is no integer-feasible solution to the primal with OFV better than the optimal dual solution we have found. If P is the OFV of the best known integer-feasible solution and D is the OFV of the optimal dual solution, we can define the Integrality Gap to be PPD If this gap falls to zero then we know (by the weak LP duality theorem) that P is the integeroptimal solution. All techniques for solving IPs aim to iteratively reduce this gap until it is within some acceptable threshold. Report the integrality gap of the solutions to the facility location problem that you found in part (c)











