Question: Figure shows a uniform beam subject to a linearly increasing distributed load. The equation for the resulting elastic curve is w. (-X* + 21X

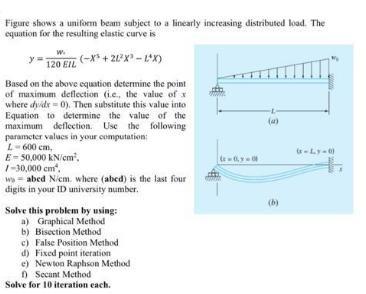
Figure shows a uniform beam subject to a linearly increasing distributed load.

Figure shows a uniform beam subject to a linearly increasing distributed load. The equation for the resulting elastic curve is w. (-X* + 21X - L*X) 120 EIL Based on the above equation determine the point of maximum deflection (i.e., the value of x where dyidr = 0). Then substitute this value into Equation to determine the value of the maximum deflection. Use the following parameter values in your computation: L- 600 cm, E- 50,000 KN/cm. 1-30,000 cm. w = abed Niem. where (abed) is the last four digits in your ID university number. tey (b) Solve this problem by using: a) Graphical Method b) Bisection Method c) False Position Method d) Fixed point iteration e) Newton Raphson Method n Secunt Method Solve for 10 iteration cach.

Step by Step Solution

★★★★★

3.45 Rating (158 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

To solve this problem we need to use the deflection equation given for the beam y fracw120 E I L lef... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Civil Engineering Questions!

Figure a, shows a uniform beam subject to a linearly increasing distributed load. The equation for the resulting elastic curve is (see Figure b) Use bisection to determine the point of maximum...

Figure P2.26 a shows a uniform beam subject to a linearly increasing distributed load. As depicted in Fig. P2.26 b , deflection y (m) can be computed with where E = the modulus of elasticity and I =...

Figure shows a uniform disk that can rotate around its center like a merry-go-round. The disk has a radius of 2.00 cm and a mass of 20.0 grams and is initially at rest. Starting at time t = 0, two...

We are to write a script using the Bisection Method using MatLab where there may be functions within the script. 8.18 Figure P8.18a shows a uniform beam subject to a linearly increasing distributed...

Solve by using MATLAB and bisection method only! Figure P5.13a shows a uniform beam subject to a linearly increasing distributed load. The equation for the resulting elastic curve is wo y = 120EIL x3...

How are governments' vanilla fixed-coupon bonds' and inflation-linked bonds' (ILB) yields typically quoted? Select one: a. Vanilla fixed coupon yields are quoted as nominal, while ILB's are quoted as...

In matlab Do problem 5.13 (same number for second and third edition) in the text. while th P, (t where ramete P,(t) a The p u.min 10,00 use ( 5.16 char tron the elec erei equ FIGURE P5.13 5.13 Figure...

Figure P5.3 shows a pinned-fixed beam subject to a uniform load. The equation for the resulting deflections is Develop a MATLAB script that (a) plots the function, dyldx versus x (with appropriate...

Below is my code Error that i see while running the Tutotial_main.m file Error using diff Difference order N must be a positive integer scalar. Error in Newton_Raphson_tutorial (line 35) f_prime0 =...

I need to know how to do the sections under Master It! and Solution Ross, Westerfield, Jaffe, and Jordan's Spreadsheet Master Corporate Finance, 11th edition by Brad Jordan and Joe Smolira Version...

What measures can you use for the following discrete frequencydistribution? Class Frequency1 Class Fequency7 0-5 6-10 11-15 8 16-20 21-25 26-30 31-35 36-40 4

Phillip Florrick is responsible for the warehouse operation for a local discount department store chain. The warehouse has only one unloading dock that is currently operated by a single three- person...

Question 5 7 of 8 5 Participating policies are issued by which type of insurance company? A . Authorized B . Surplus lines C . Mutual D . Stock

A large study used records from Canadas national health care system to compare the effectiveness of two ways to treat prostate disease. The two treatments are traditional surgery and a new method...

The Environmental Protection Agency (EPA) has determined that safe drinking water should contain at most 1.3 mg/liter of copper, on average. A water supply company is testing water from a new source...

Consider the problem of determining whether a Turing machine M on an input w ever attempts to move its head left at any point during its computation on w. Formulate this problem as a language and...

Consider the problem of determining whether a Turing machine M on an input w ever attempts to move its head left when its head is on the left most tape cell. Formulate this problem as a language and...

In a 2018 study reported in The Lancet, a randomized, double-blind controlled experiment was conducted to determine the effect of the drug upadacitinib on patients with active rheumatoid arthritis....

Analyse the relevant legislation (acts and regulations) for each of the following areas, including the full title of the legislation. Identify full name of Legislation and/or subordinate legislation,...

write the matlab code for 2 & 3 quickly ? 2. Fol llowing figure shows a uniform beam subject to a linearly increasing distributed load. The equation for the resulting elastic curve is: y-wall (f...

write the matlab code? 2. Fol llowing figure shows a uniform beam subject to a linearly increasing distributed load. The equation for the resulting elastic curve is: y-wall (f +213x3- L4x) 120EIL 0...

write the matlab code for 2 and 3 2. Fol llowing figure shows a uniform beam subject to a linearly increasing distributed load. The equation for the resulting elastic curve is: y-wall (f +213x3- L4x)...

(25 Points) In the figure below, figure (a) shows a uniform beam subject to a linearly increasing distributed load which starts a 0 at the left end and increases to Wo on the right end. As depicted...

Figure below (a) shows a uniform beam subject to a linearly increasing distributed load. As depicted in Fig. (b), deflection y (m) can be computed with y-120E1 Where E the modulus of elasticity and I...

Solve by matlab and submitte m-file The figure given below shows a uniform beam subject to a linearly increasing distributed load. The equation for the resulting elastic curve is as follows: EI (1x =...