Problem 1;
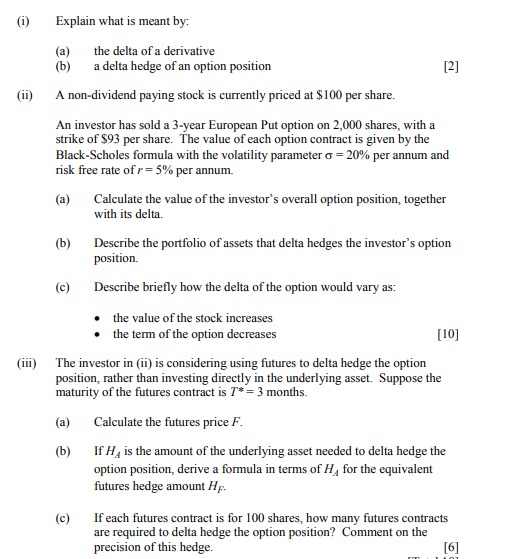
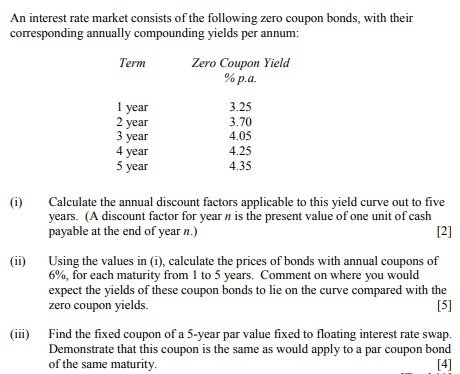
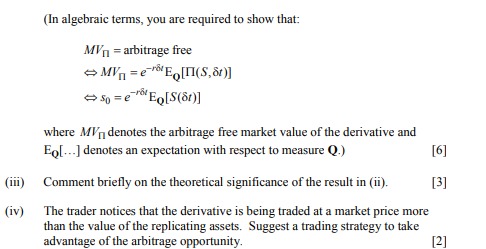
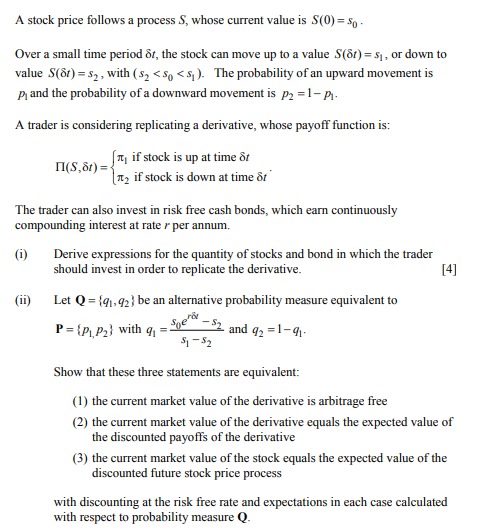
(i) Explain what is meant by: (a) the delta of a derivative (b) a delta hedge of an option position [2] (ii) A non-dividend paying stock is currently priced at $100 per share. An investor has sold a 3-year European Put option on 2,000 shares, with a strike of $93 per share. The value of each option contract is given by the Black-Scholes formula with the volatility parameter o = 20% per annum and risk free rate of r = 5% per annum. (a) Calculate the value of the investor's overall option position, together with its delta. (b) Describe the portfolio of assets that delta hedges the investor's option position. (c) Describe briefly how the delta of the option would vary as: the value of the stock increases . the term of the option decreases [10] (iii) The investor in (ii) is considering using futures to delta hedge the option position, rather than investing directly in the underlying asset. Suppose the maturity of the futures contract is 7* = 3 months. (a) Calculate the futures price F. (b) If Hy is the amount of the underlying asset needed to delta hedge the option position, derive a formula in terms of Hy for the equivalent futures hedge amount HF. (c) If each futures contract is for 100 shares, how many futures contracts are required to delta hedge the option position? Comment on the precision of this hedge. [6]An interest rate market consists of the following zero coupon bonds, with their corresponding annually compounding yields per annum: Term Zero Coupon Yield % p.a. 1 year 3.25 2 year 3.70 3 year 4.05 4 year 4.25 5 year 4.35 (i) Calculate the annual discount factors applicable to this yield curve out to five years. (A discount factor for year n is the present value of one unit of cash payable at the end of year n.) [2] (ii) Using the values in (i), calculate the prices of bonds with annual coupons of 6%, for each maturity from 1 to 5 years. Comment on where you would expect the yields of these coupon bonds to lie on the curve compared with the zero coupon yields. [5] (iii) Find the fixed coupon of a 5-year par value fixed to floating interest rate swap. Demonstrate that this coupon is the same as would apply to a par coupon bond of the same maturity. [4](In algebraic terms, you are required to show that: MYn = arbitrage free MV="E[II(S, 80)] 50= 2Eo[S(80)] where MI denotes the arbitrage free market value of the derivative and Eo[...] denotes an expectation with respect to measure Q.) [6] (iii) Comment briefly on the theoretical significance of the result in (ii). [3] (iv) The trader notices that the derivative is being traded at a market price more than the value of the replicating assets. Suggest a trading strategy to take advantage of the arbitrage opportunity.A stock price follows a process S, whose current value is S(0) =$ . Over a small time period of, the stock can move up to a value S(8) = $, or down to value S(87) = $2, with ($2