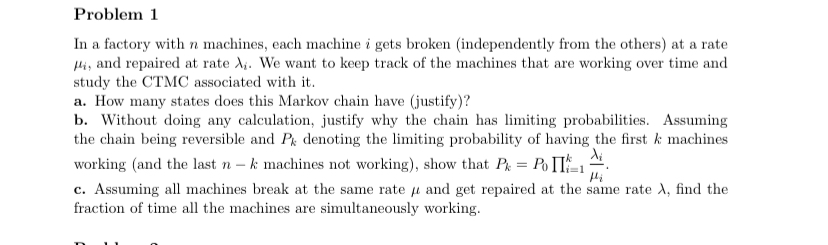
Problem 1 In a factory with n machines, each machine i gets broken (independently from the others) at a rate Hi, and repaired at rate A. We want to keep track of the machines that are working over time and study the CTMC associated with it. a. How many states does this Markov chain have (justify)? b. Without doing any calculation, justify why the chain has limiting probabilities. Assuming the chain being reversible and Pk denoting the limiting probability of having the first k machines working (and the last n - k machines not working), show that P = Po c. Assuming all machines break at the same rate and get repaired at the same rate A, find the fraction of time all the machines are simultaneously working. Q: A factory consists of N identical machines, each operated by an employee. When a machine breaks down, its operator immediately begins to repair it. Each machine independently breaks down at rate and each repair independently takes Exp(1) time. 1. How many states are needed to describe the corresponding CTMC? Because all the machines are identical, we can simply model, the # machine working State nn working machines. describe the CTMC. Over time to N+1 states and S = {0,..., N3 # of machines rate at which. 2. What kind of CTMC is it? that may break 1. machine breaks. 3 # of broken machines if n are working Birth death process Mn = n. M . (N-n) A + 3. After, the factory has been in operation for several years, an inspector visits. Determine the probability that the inspector finds at least one broken machine. Step 1: Find limiting probabilities. Po, P, Step 2 Ans. 1-PN. = We are looking for at least one broken machine ..PN. rate at which I machine is required PN=Probability that they are all working. Po= Probability that they are Detailed balence equations: all broken. 90,1 Po = 91,0. P .Po = M.P. P. = Po M 91.2 Pl = 421P2. 2.P = P P MM Po Pn = An- Mn : Po =rn: Po M (N-(n-1)) (N-(n-2)) N! n! (N-n)! (N-1). Nn 21 Mn = (N) (A)" P.n = N rn = Mn = M (^)(^)^ Po = Pn = + n-in-1) Po Po = ((^) 1-2 pm (1-2 (") (4)^) R - (2 (^) (#)") P-) n=o Note: (A) n = in MN-n 17+MIN TA+MIN MN N-n = ( A+M +M P. I-P Plug in above: N 1 = (1+1). Po 2 (N) P^ 11-P)" P(Bin IN, P) = n ) Po = 7+