Problem 1.

Problem 2.

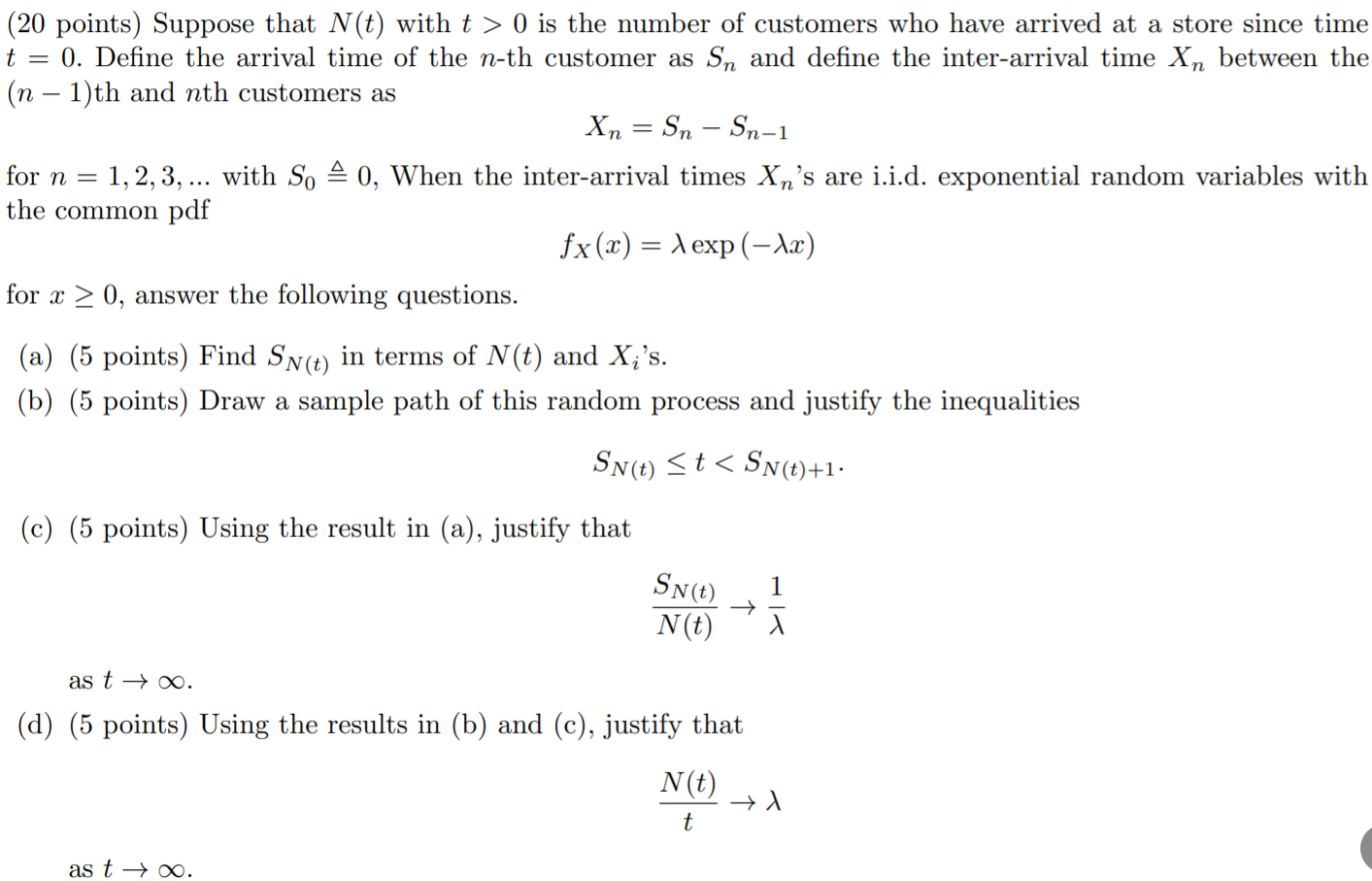
Problem 3.

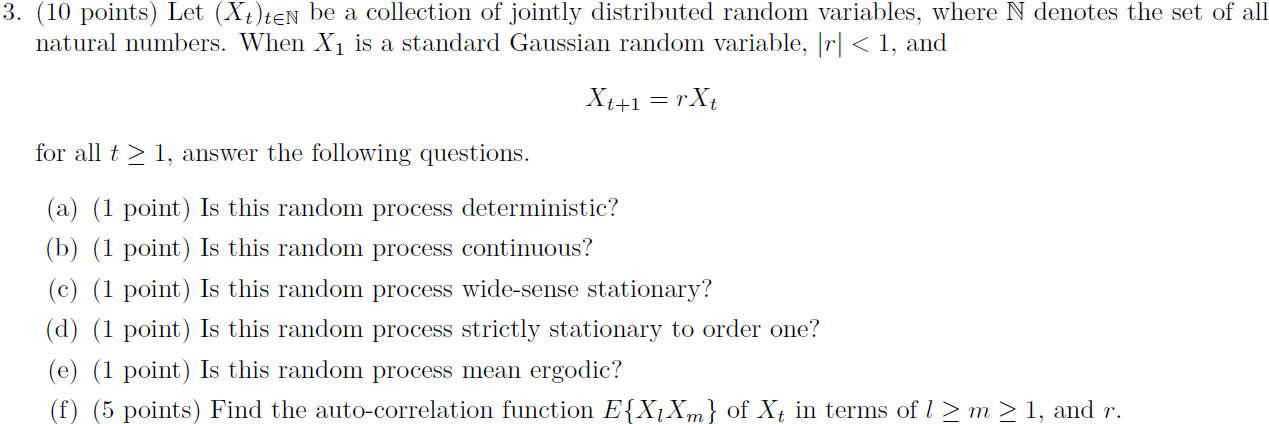
Problem 4.

1. (10 points) Let X; for i =1,2,..., n be independent and identically distributed random variables with mean u > 0 and standard deviation o > 0. When we define Mn and Zn as , M, n and Zn 211(X; u) no respectively, answer the following questions. (a) (8 points) Find the mean and variance of Mn, respectively. Also find the mean and variance of Zn, respectively. (b) (2 points) Find limn + Mn. Also find limn too Zn. (20 points) Suppose that N(t) with t > 0 is the number of customers who have arrived at a store since time t = 0. Define the arrival time of the n-th customer as Sn and define the inter-arrival time Xn between the (n 1)th and nth customers as Xn = Sn Sn-1 for n = 1, 2, 3, ... with S. 40, When the inter-arrival times Xn's are i.i.d. exponential random variables with the common pdf fx(x) = lexp (- x) for x > 0, answer the following questions. = (a) (5 points) Find Sn(t) in terms of N(t) and X;'s. (b) (5 points) Draw a sample path of this random process and justify the inequalities Sn(t) 1, answer the following questions. (a) (1 point) Is this random process deterministic? (b) (1 point) Is this random process continuous? (C) (1 point) Is this random process wide-sense stationary? (d) (1 point) Is this random process strictly stationary to order one? (e) (1 point) Is this random process mean ergodic? (f) (5 points) Find the auto-correlation function E{X/Xm} of Xt in terms of 1 > m > 1, and r. 4. (10 points) Let X(t) be a Poisson random process with Pr(X(t) = n) = -14 (At)n =e n! for 1 > 0,t> 0, and n = 0,1,2, .... Answer the following questions. (a) (8 points) Find the mean and variance of X (1), respectively. (b) (2 points) Find the conditional mean and variance of (X(t+1) X(t)) for t > 1, respectively, given the event {X(1) = 3}. 1. (10 points) Let X; for i =1,2,..., n be independent and identically distributed random variables with mean u > 0 and standard deviation o > 0. When we define Mn and Zn as , M, n and Zn 211(X; u) no respectively, answer the following questions. (a) (8 points) Find the mean and variance of Mn, respectively. Also find the mean and variance of Zn, respectively. (b) (2 points) Find limn + Mn. Also find limn too Zn. (20 points) Suppose that N(t) with t > 0 is the number of customers who have arrived at a store since time t = 0. Define the arrival time of the n-th customer as Sn and define the inter-arrival time Xn between the (n 1)th and nth customers as Xn = Sn Sn-1 for n = 1, 2, 3, ... with S. 40, When the inter-arrival times Xn's are i.i.d. exponential random variables with the common pdf fx(x) = lexp (- x) for x > 0, answer the following questions. = (a) (5 points) Find Sn(t) in terms of N(t) and X;'s. (b) (5 points) Draw a sample path of this random process and justify the inequalities Sn(t) 1, answer the following questions. (a) (1 point) Is this random process deterministic? (b) (1 point) Is this random process continuous? (C) (1 point) Is this random process wide-sense stationary? (d) (1 point) Is this random process strictly stationary to order one? (e) (1 point) Is this random process mean ergodic? (f) (5 points) Find the auto-correlation function E{X/Xm} of Xt in terms of 1 > m > 1, and r. 4. (10 points) Let X(t) be a Poisson random process with Pr(X(t) = n) = -14 (At)n =e n! for 1 > 0,t> 0, and n = 0,1,2, .... Answer the following questions. (a) (8 points) Find the mean and variance of X (1), respectively. (b) (2 points) Find the conditional mean and variance of (X(t+1) X(t)) for t > 1, respectively, given the event {X(1) = 3}