
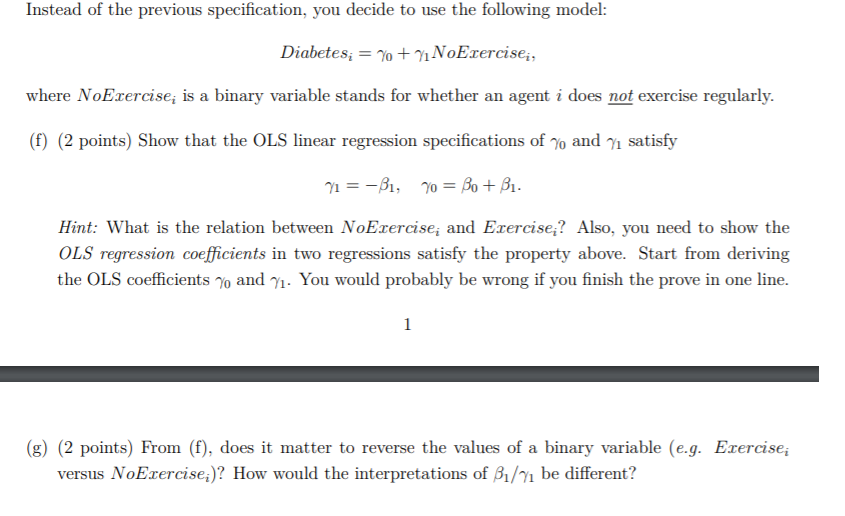
Problem 1 Suppose you are studying the correlation between diabetes and exercise. You have a data set describing whether individuals in the sample have diabetes and whether they exercise regularly. You summarize the variables: whether an observation is diagnosed to have diabetes and whether the observation works out at least 3 times a week, 45 Exercise No Exercise With Diabetes 5 Without Diabetes 500 950 where each cell the number of observations by whether they have diabetes and whether they exercise regularly. (For example: there are 5 individuals with diabetes that exercise regularly.) You also propose a population regression model: Diabetes; = Bo + B2 Exercisei, where Diabetes; is a binary variable stands for whether an agent i has diabetes (Diabetes; = 1 if i has diabetes and Diabetes; = 0 otherwise) and Exercise; is a binary variable stands for whether an agent i exercises regularly. (a) (4 points) Derive the OLS population parameters of Bo and Bi. Be sure to start from the minimization problem. The Bo and B. you find should be functions of Diabetes; and Exercise;. (b) (1 point) Can you find the actual values of B, and B.? Why or why not? (c) (4 points) Find the OLS estimators for Bo and B1. Be sure to start from the minimization problem. The estimators you derive should be a function of your observations. (d) (2 points) Using the table above, calculate the estimates of Bo and Bi. (e) (2 points) Interpret the estimates of Bo and B. you find in (d). Instead of the previous specification, you decide to use the following model: Diabetes; = % +71 NoEcercisei, where NoExercise; is a binary variable stands for whether an agent i does not exercise regularly. (f) (2 points) Show that the OLS linear regression specifications of 7 and 71 satisfy 71 = -B1, 70 = Bo + Bi. Hint: What is the relation between NoExercise; and Exercise;? Also, you need to show the OLS regression coefficients in two regressions satisfy the property above. Start from deriving the OLS coefficients 7 and 71. You would probably be wrong if you finish the prove in one line. 1 (g) (2 points) From (f), does it matter to reverse the values of a binary variable (e.g. Erercise; versus NoEcercise;)? How would the interpretations of B1/71 be different? Problem 1 Suppose you are studying the correlation between diabetes and exercise. You have a data set describing whether individuals in the sample have diabetes and whether they exercise regularly. You summarize the variables: whether an observation is diagnosed to have diabetes and whether the observation works out at least 3 times a week, 45 Exercise No Exercise With Diabetes 5 Without Diabetes 500 950 where each cell the number of observations by whether they have diabetes and whether they exercise regularly. (For example: there are 5 individuals with diabetes that exercise regularly.) You also propose a population regression model: Diabetes; = Bo + B2 Exercisei, where Diabetes; is a binary variable stands for whether an agent i has diabetes (Diabetes; = 1 if i has diabetes and Diabetes; = 0 otherwise) and Exercise; is a binary variable stands for whether an agent i exercises regularly. (a) (4 points) Derive the OLS population parameters of Bo and Bi. Be sure to start from the minimization problem. The Bo and B. you find should be functions of Diabetes; and Exercise;. (b) (1 point) Can you find the actual values of B, and B.? Why or why not? (c) (4 points) Find the OLS estimators for Bo and B1. Be sure to start from the minimization problem. The estimators you derive should be a function of your observations. (d) (2 points) Using the table above, calculate the estimates of Bo and Bi. (e) (2 points) Interpret the estimates of Bo and B. you find in (d). Instead of the previous specification, you decide to use the following model: Diabetes; = % +71 NoEcercisei, where NoExercise; is a binary variable stands for whether an agent i does not exercise regularly. (f) (2 points) Show that the OLS linear regression specifications of 7 and 71 satisfy 71 = -B1, 70 = Bo + Bi. Hint: What is the relation between NoExercise; and Exercise;? Also, you need to show the OLS regression coefficients in two regressions satisfy the property above. Start from deriving the OLS coefficients 7 and 71. You would probably be wrong if you finish the prove in one line. 1 (g) (2 points) From (f), does it matter to reverse the values of a binary variable (e.g. Erercise; versus NoEcercise;)? How would the interpretations of B1/71 be different








