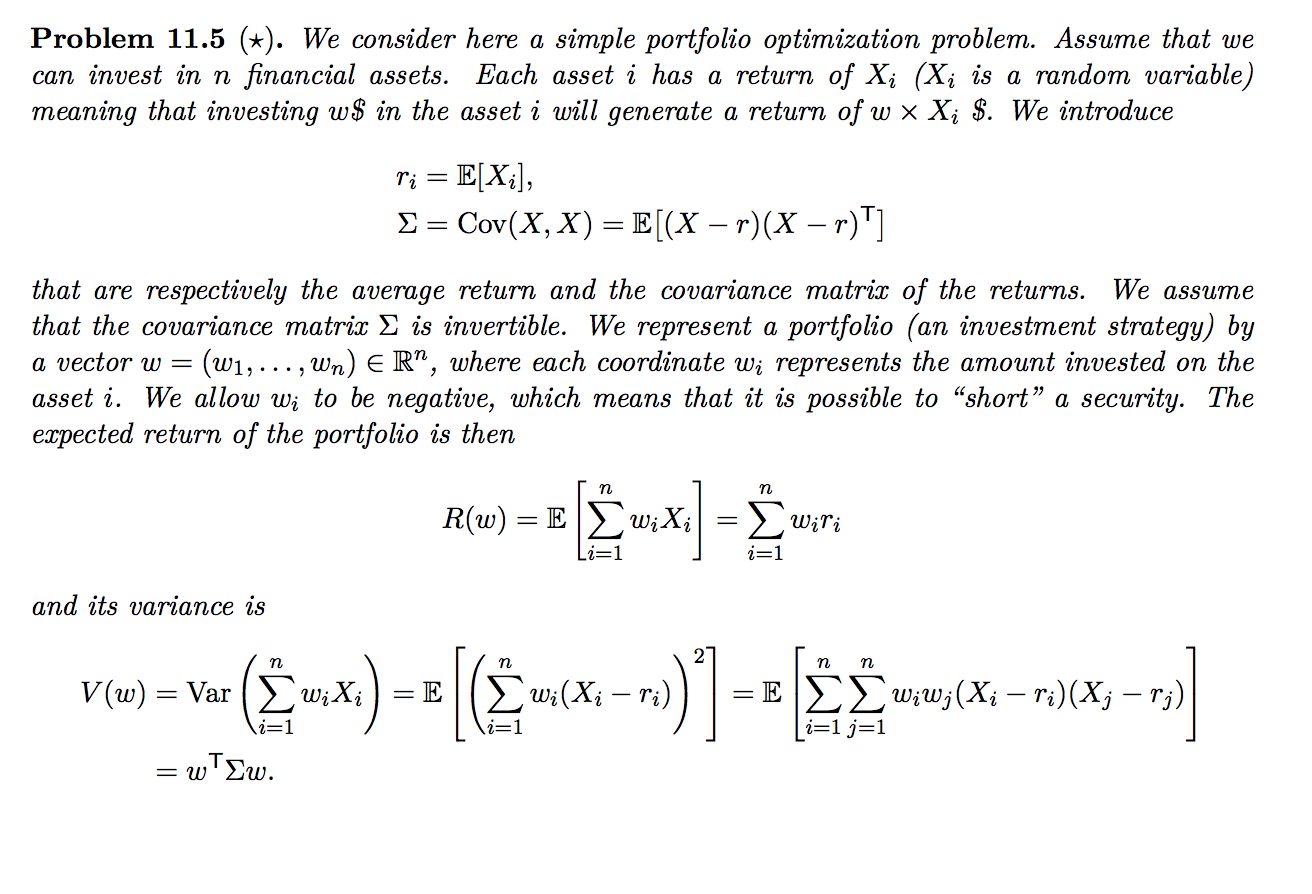
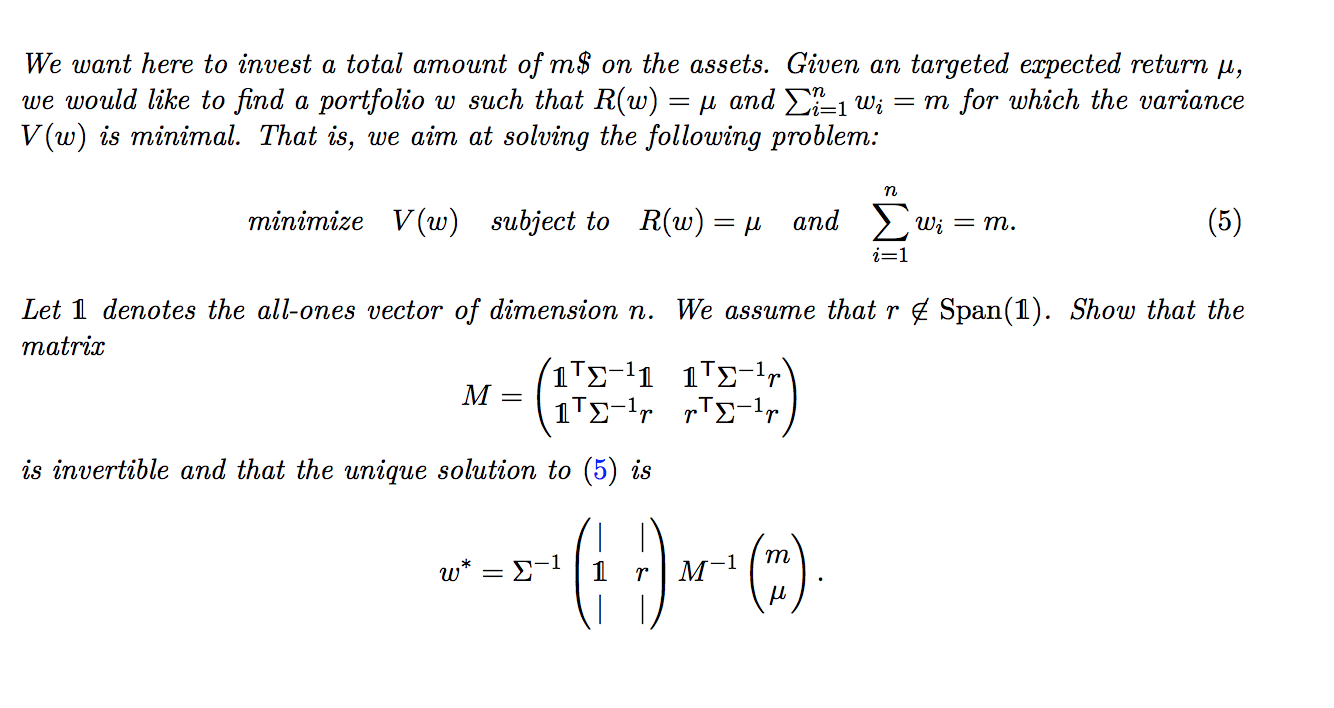
Problem 11.5 (*). We consider here a simple portfolio optimization problem. Assume that we can invest in n financial assets. Each asset i has a return of Xi (X; is a random variable) meaning that investing w$ in the asset i will generate a return of w x X; $. We introduce = E[X;], E = Cov(X, X) = E[(X r)(X r)"] that are respectively the average return and the covariance matri of the returns. We assume that the covariance matrix E is invertible. We represent a portfolio (an investment strategy) by a vector w = (w1,..., wn) E R", where each coordinate w; represents the amount invested on the asset i. We allow wi to be negative, which means that it is possible to short" a security. The expected return of the portfolio is then = E> w;X; Wiri Li=1 i=1 and its variance is vie) = Ver (Emx) = = (mcx, - n)] = = EE- ~)]-| : ; w, (X; -) (X-T) |i=1 j=1 i=1 w". We want here to invest a total amount of m$ on the assets. Given an targeted expected return , we would like to find a portfolio w such that R(w) = and E1 w; = m for which the variance V(w) is minimal. That is, we aim at solving the following problem: minimize V(w) subject to R(w) = (5) and > uWi = m. i= Let 1 denotes the all-ones vector of dimension n. We assume thatr Span(1). Show that the atrix (1-1 1 11) M = 1-r r-1r) is invertible and that the unique solution to (5) is (()- w. 1 r| M-1 Problem 11.5 (*). We consider here a simple portfolio optimization problem. Assume that we can invest in n financial assets. Each asset i has a return of Xi (X; is a random variable) meaning that investing w$ in the asset i will generate a return of w x X; $. We introduce = E[X;], E = Cov(X, X) = E[(X r)(X r)"] that are respectively the average return and the covariance matri of the returns. We assume that the covariance matrix E is invertible. We represent a portfolio (an investment strategy) by a vector w = (w1,..., wn) E R", where each coordinate w; represents the amount invested on the asset i. We allow wi to be negative, which means that it is possible to short" a security. The expected return of the portfolio is then = E> w;X; Wiri Li=1 i=1 and its variance is vie) = Ver (Emx) = = (mcx, - n)] = = EE- ~)]-| : ; w, (X; -) (X-T) |i=1 j=1 i=1 w". We want here to invest a total amount of m$ on the assets. Given an targeted expected return , we would like to find a portfolio w such that R(w) = and E1 w; = m for which the variance V(w) is minimal. That is, we aim at solving the following problem: minimize V(w) subject to R(w) = (5) and > uWi = m. i= Let 1 denotes the all-ones vector of dimension n. We assume thatr Span(1). Show that the atrix (1-1 1 11) M = 1-r r-1r) is invertible and that the unique solution to (5) is (()- w. 1 r| M-1








