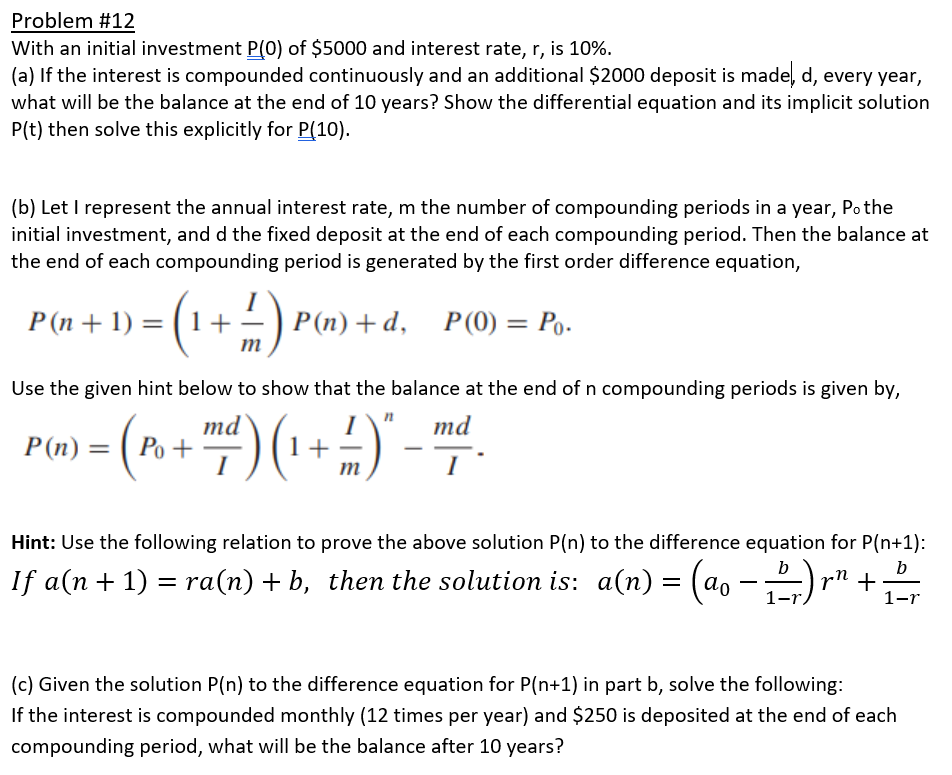
Problem #12 With an initial investment Plo) of $5000 and interest rate, r, is 10%. (a) If the interest is compounded continuously and an additional $2000 deposit is madel , d, every year, what will be the balance at the end of 10 years? Show the differential equation and its implicit solution P(t) then solve this explicitly for P(10). (b) Let I represent the annual interest rate, m the number of compounding periods in a year, Po the initial investment, and d the fixed deposit at the end of each compounding period. Then the balance at the end of each compounding period is generated by the first order difference equation, P(n + 1) = (1+ ( m P(n) + d, P(0) = P. Use the given hint below to show that the balance at the end of n compounding periods is given by, md md P(n) = Po + m 1 (1+) m) PC ma) (1+ "- Hint: Use the following relation to prove the above solution P(n) to the difference equation for P(n+1): b b + 1-r If a(n + 1) = ra(n) +b, then the solution is: a(n) = (ao - :) rn + L- (c) Given the solution P(n) to the difference equation for P(n+1) in part b, solve the following: If the interest is compounded monthly (12 times per year) and $250 is deposited at the end of each compounding period, what will be the balance after 10 years? Problem #12 With an initial investment Plo) of $5000 and interest rate, r, is 10%. (a) If the interest is compounded continuously and an additional $2000 deposit is madel , d, every year, what will be the balance at the end of 10 years? Show the differential equation and its implicit solution P(t) then solve this explicitly for P(10). (b) Let I represent the annual interest rate, m the number of compounding periods in a year, Po the initial investment, and d the fixed deposit at the end of each compounding period. Then the balance at the end of each compounding period is generated by the first order difference equation, P(n + 1) = (1+ ( m P(n) + d, P(0) = P. Use the given hint below to show that the balance at the end of n compounding periods is given by, md md P(n) = Po + m 1 (1+) m) PC ma) (1+ "- Hint: Use the following relation to prove the above solution P(n) to the difference equation for P(n+1): b b + 1-r If a(n + 1) = ra(n) +b, then the solution is: a(n) = (ao - :) rn + L- (c) Given the solution P(n) to the difference equation for P(n+1) in part b, solve the following: If the interest is compounded monthly (12 times per year) and $250 is deposited at the end of each compounding period, what will be the balance after 10 years







