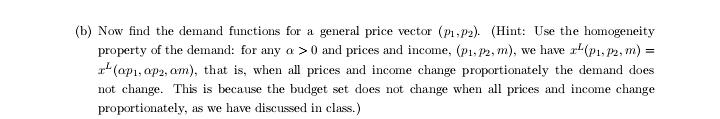
Problem 2. Intertemporal choice Mainy Landin has an income of 200.000 kr this year and she expects an income of 110.000 kr next year. She can borrow and lend money at an interest rate of 10%. Consumption goods cost 1 kr and there is no inflation. a. What is the present value of Mainy's endowment? b. What is the future value of Mainy's endowment? c. Suppose that Mainy has the utility function U = cic. Write down Mainy's marginal rate of substitution. d. Set this slope equal to the slope of the budget line and solve for the consumption in period 1 and 2. Will she borrow or save in the first period. e. = d, but the interest rate is 20%. Will Mainy be better or worse off? Problem 1. Uncertainty Jonas Thern maximises expected utility: Jonas's friend Stefan Schwarz has offered to bet him 10.000 kr on the outcome of the toss of a coin. If the coin comes up head, Jonas must pay Stefan 10.000 kr, and if the coin comes up tails, Stefan must pay Jonas 10.000 kr. If Jonas doesn't accept the bet, he will have 100.000 kr with certainty. Let Event 1 be "coin comes up heads". a. What is Jonas's utility if he accepts the bet and if he decides not to bet? Does Jonas take the bet? b. Answer the question in a, if the bet is 100.000 kr. c. Answer the question if Jonas must pay Stefan 100.000 kr if he coin comes up head, but if the coin comes up tails Stefan must pay Jonas 500.000 kr. d. Klas Ingesson would also like to gamble with Jonas. He is very intelligent and realises the nature of Jonas' preferences. He offers him a bet that Jonas will take. Klas says: "If you loose you will give me 10.000 kr. If you win, I will give you ......? Problem 2. Uncertainty Gabriel likes to gamble and his preferences are represented by the expected utility function U = mic," + nyCz Gabriel has not worked out very well, he only have 1.000 kr. Thomas shuffled a deck of cards and offered to bet Gabriel 200 kr that he would not cut a spade from the deck. a. Show that Gabriel refuses the bet. b. Would Gabriel accept the bet if they would bet 1.000 kr instead of 200 kr? c. Sketch one of Gabriel's indifference curves (let Event 2 be the event that a card drawn from a fair deck of cards is a spade) d. On the same graph, sketch the indifference curve when the gamble is that he would not cut a black card from the deck. Problem 3. Uncertainty Consider an individual with an income of 100. She has the option of participating in a lottery where she can win 30 with a probability of 0.5, and loose 30 with a probability of 0.5. Would she participate if she is risk averse? What id she is a risk lover? Explain(c) Since Alex got his job in Toronto, the Canadian dollar (the currency in which Alex is paid his salary) has been becoming increasingly more valuable relative to the U.S. dollar. Because Alex visits the U.S. quite often, it is as easy for him to shop for clothes in either country. Lately, he has been doing more and more of his clothes shopping in the U.S. The price tags have not changed in either country, and the clothes Alex buys are the same kind in both countries. For Alex, what kind of good are clothes?(b) In his grad student days, Alex hardly ever bought himself a lemonade, let alone buying lemonade for others. Now, when he comes back to visit Boston, it's quite possible to see him buy entire rounds of lemonades, even for strangers. The price of lemonade in Boston has not changed, nor have Alex's preferences. For Alex, what kind of good is lemonade?1. Ann is deciding whether to bet on a tennis match. A friend offers to give her 20 dollars if the lower ranked player wins, while she has to pay him 12 dollars otherwise. The utility that she derives from a (positive or negative) cash transfer of r dollars is determined by the following utility function, u(r) = (16 +x) 1/2. Ann believes that the probability of the lower ranked player winning the match is p. (a) Find the expected value of this lottery. For what values of p is the expected value positive?" 5 marks) (b) Find Ann's expected utility when betting on the match. For what values of p would she accept the bet? (6 marks) (c) Find Ann's certainty equivalent for this lottery when p = 3/4. (6 marks) 2. Consider the following Bayesian game played by two players 1 and 2. Two states are possible, A and B. Suppose that player 2 knows state, while player 1 deems both states equally likely. Payoffs in each state respectively satisfy 1\\2 8 P 1\\2 8 P State A: 1, 1 0,2 State B: 0, 2 1, 1 . p 0,0 2,0 p 2,0 0,0 Player 1 is the row player, and his payoff is the first to appear in each entry. Player 2 is the column player and his payoff is the second to appear in each entry. (a) What is the set of possible strategies for either player in this game? (7 marks) (b) Find a pure strategy Bayes Nash equilibrium of the game. (10 marks) 3. Suppose that two friends have split 5 indivisible cookies according to the following protocol. Player 1 gets to divide the cookies between two dishes and Player 2 gets to choose which of the two dishes to consume (while the remaining dish is consumed by Player 1). First, assume that all cookies are alike, so that both players value every cookie equally, and care only about consuming more cookies. (a) Set up this problem as an extensive form game. [4 marks] (b) Find a Subgame Perfect equilibrium of this game. Write the behavioral strategy for both players, and check that no deviation is profitable. [6 marks] (c) Does the game possess any Nash equilibrium that is not Subgame Perfect? [7 marks](b) Now find the demand functions for a general price vector (p1,p2). (Hint: Use the homogeneity property of the demand: for any o > 0 and prices and income, (p1, pz, m), we have r(pi, p, m) = r" (opi, ops, am), that is, when all prices and income change proportionately the demand does not change. This is because the budget set does not change when all prices and income change proportionately, as we have discussed in class.)