Question: Problem 2. Let p be a prime number. (a) (5 pts) Let f(T) be a polynomial modulo p of degree 2 or 3. Prove

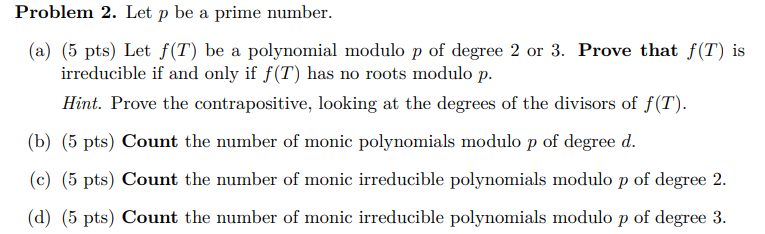
Problem 2. Let p be a prime number. (a) (5 pts) Let f(T) be a polynomial modulo p of degree 2 or 3. Prove that f(T) is irreducible if and only if f(T) has no roots modulo p. Hint. Prove the contrapositive, looking at the degrees of the divisors of f(T). (b) (5 pts) Count the number of monic polynomials modulo p of degree d. (c) (5 pts) Count the number of monic irreducible polynomials modulo p of degree 2. (d) (5 pts) Count the number of monic irreducible polynomials modulo p of degree 3.

Step by Step Solution

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

The synthesis of compound C was achieved by using compounds A and B as the sources of all carbon atoms. Suggest a synthetic sequence involving no more than three steps by which A and B may be...

The crate C has a weight of 150 lb and rests on the truck elevator for which the coefficient of static friction is s = 0.4. Determine the largest initial angular acceleration a, starting from rest,...

Solve Prob. 834 using Castiglianos theorem. Data from Prob. 834 Determine the slope and displacement at point B. Assume the support at A is a pin and C is a roller. Take E = 200 GPa, I = 150(10 6 )...

Shirley Processing Incorporated (SPI) makes adhesive tape. The following information on the physical flow of units and costs for month of March Shirley Processing, Incorporated (SPI) makes adhesive...

4 1. Let F be a finite field. Prove that there are infinitely many irreducible polynomials in F [x]. 2. Let p be a prime number. Prove that f (x) = xp1 + xp2 + . . . + x + 1 is irreducible in Q[x]....

(a) In SystemVerilog, what is the difference between: (i) The ternary operator ? and if...then...else statements? [2 marks] (ii) always_ff and always_comb? [2 marks] (iii) Blocking, non-blocking and...

Please do not use chatgpt to answer _______________________________________________ 1. Which statements are correct A) A block cipher according to Shannon should comprise two atomic operations, i.e....

= = (5) Let f be an irreducible polynomial of degree r in Fp[x] where p is an odd prime, and R = {[g]: geFp[x]} be the ring of congruence classes mod f. Let Qp = {G? ER*:G e R*} be the group of...

1456HHSC attend all (a) In the quantum teleportation protocol, Alice and Bob are every in possession of one qubit of a couple in the joint country00i + statei. Explain how the protocol works. In...

Describe, and estimate the costs of, procedures to (a) insert a new item into an existing heap; (b) delete the topmost item from a non-empty heap; (c) starting from an array holding N items in...

For the circuit shown in figure 7.1, choose resistor values that will maximize the power transferred to the 2.7k2 resistor. R1 R3 10V R2 2.7KO Figure 7.1

Sympco Glass manufactures insulated windows. The firms repair and maintenance (R&M) cost is mixed and varies most directly with machine hours worked. The following data have been gathered from recent...

Constructing a financial plan led by client s mission is what approach?

Jimbo wishes to purchase a special whole life insurance policy that pays $10,000 at the end of the year of death but only if death occurs while his age is an even number. Jimbo is currently 41 years...

Peachtree Hospital chief administrator, Chad Matthews, is concerned about the costs in the laboratory, which performs tests for the hospital. Due to increased pressure from insurance companies to...

You can use the t-value as test statistic when the sample size is large sample size is small and population standard deviation is unknown sample size is small or population standard deviation is...

1-Where in 1040 form can I report income from group-term life insurance coverage in excess of $50,000 ($132.48), and is reduced by 7 percent contribution ($6,860) to USCs qualified pension plan, and...

One of your Real Estate Customer wants your help. Your Customer wants to improve his/her Front Lawn by: Having a Water Fountain in a Circular Diameter of 15 Ft and Planting Rose bushes around the...

Cules de los siguientes son ejemplos de activos intangibles reconocidos segn las normas contables? Pregunta 10Respuesta a. Marcas, patentes, derechos legales y bienes materiales. b. Marcas, patentes,...

Proof that: (a) If O d Q, then is Galois over Q. (b) C is Galois over R. (PM)D

Show that the center of S 4 is (e); conclude that S 4 is isomorphic to the group of all inner automorphisms of S 4 .

Let G be an (additive) abelian group with subgroups H and K. Show that G H K if and only if there are homomorphisms such that 1 L 1 = 1 H , 2 L 2 = 1 K , 1 L 2 = 0 and 2 L 1 = 0, where 0 is the...

The Vehicle Inventory and Use Survey (VIUS) has been conducted by the U.S. government to provide information on the number of private and commercial trucks in each state. The stratified random...

In Exercise 6 of Chapter 2, data on numbers of publications were given for an SRS of 50 faculty members. Not all departments were represented, however, in the SRS. The SRS contained several faculty...

The data in the file radon.dat were collected from 1003 homes in Minnesota in 1987 (Tate, 1988) in order to estimate the prevalence and distribution of households with high indoor radon...