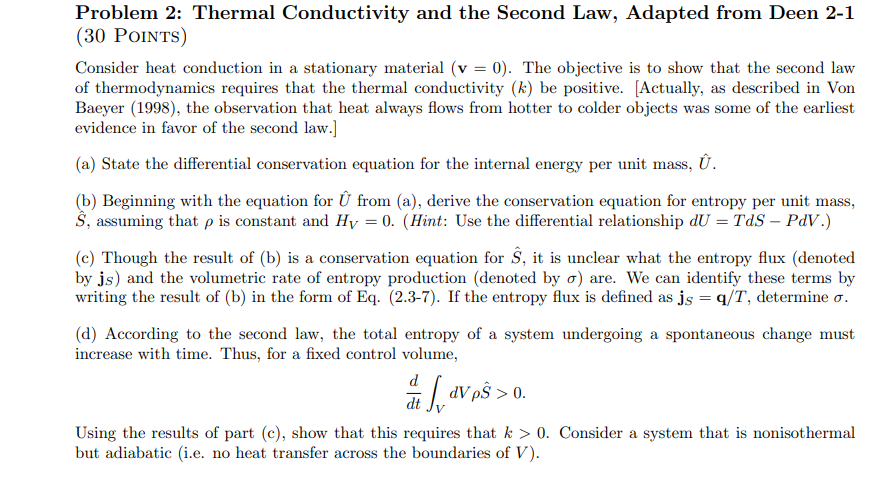
Problem $2$: Thermal Conductivity and the Second Law, Adapted from Deen $2-1$

$(30$ Points$)$

Consider heat conduction in a stationary material $(v=0).$ The objective is to show that the second law

of thermodynamics requires that the thermal conductivity $(k)$ be positive. $[$Actually$,$ as described in Von

Baeyer $(1998),$ the observation that heat always flows from hotter to colder objects was some of the earliest

evidence in favor of the second law.$]$

$($a$)$ State the differential conservation equation for the internal energy per unit mass, hat$(U).$

$($b$)$ Beginning with the equation for hat$(U)$ from $($a$),$ derive the conservation equation for entropy per unit mass,

hat$(S),$ assuming that $$ is constant and $H_V=0.($Hint: Use the differential relationship $dU=TdS-PdV.)$

$($c$)$ Though the result of $($b$)$ is a conservation equation for hat$(S),$ it is unclear what the entropy flux $($denoted

by $j_S)$ and the volumetric rate of entropy production $($denoted by $)$ are. We can identify these terms by

writing the result of $($b$)$ in the form of Eq$.(2\mathrm{.}3-7).$ If the entropy flux is defined as $j_S=\frac{q}{T},$ determine $.$

$($d$)$ According to the second law, the total entropy of a system undergoing a spontaneous change must

increase with time. Thus, for a fixed control volume,

$\frac{d}{dt}{\displaystyle \underset{V}{\overset{\phantom{}}{}}}dVhat(S)>0.$

Using the results of part $($c$),$ show that this requires that $k>0.$ Consider a system that is nonisothermal

but adiabatic $($i$.$e$.$ no heat transfer across the boundaries of $V).$