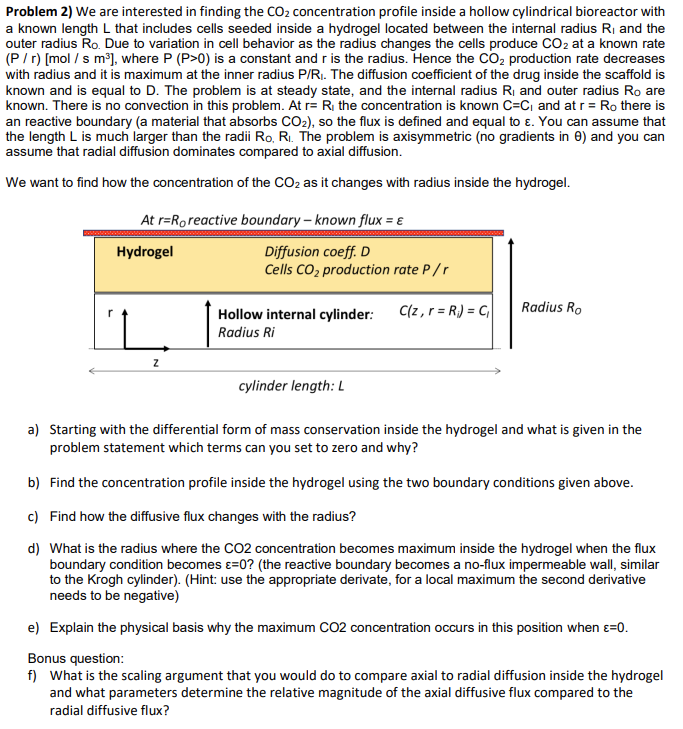
Problem 2) We are interested in finding the CO2 concentration profile inside a hollow cylindrical bioreactor with a known length L that includes cells seeded inside a hydrogel located between the internal radius R1 and the outer radius Ro. . Due to variation in cell behavior as the radius changes the cells produce CO2 at a known rate (P/r)[mol/smm3, where P(P>0) is a constant and r is the radius. Hence the CO2 production rate decreases with radius and it is maximum at the inner radius P/RI. The diffusion coefficient of the drug inside the scaffold is known and is equal to D. The problem is at steady state, and the internal radius R1 and outer radius R0 are known. There is no convection in this problem. At r=RI the concentration is known C=C1 and at r=R0 there is an reactive boundary (a material that absorbs CO2 ), so the flux is defined and equal to . You can assume that the length L is much larger than the radii R0,Rt. The problem is axisymmetric (no gradients in ) and you can assume that radial diffusion dominates compared to axial diffusion. We want to find how the concentration of the CO2 as it changes with radius inside the hydrogel. At r=Rarentivehnundaruknnwn flux = a) Starting with the differential form of mass conservation inside the hydrogel and what is given in the problem statement which terms can you set to zero and why? b) Find the concentration profile inside the hydrogel using the two boundary conditions given above. c) Find how the diffusive flux changes with the radius? d) What is the radius where the CO2 concentration becomes maximum inside the hydrogel when the flux boundary condition becomes =0 ? (the reactive boundary becomes a no-flux impermeable wall, similar to the Krogh cylinder). (Hint: use the appropriate derivate, for a local maximum the second derivative needs to be negative) e) Explain the physical basis why the maximum CO2 concentration occurs in this position when =0. Bonus question: f) What is the scaling argument that you would do to compare axial to radial diffusion inside the hydrogel and what parameters determine the relative magnitude of the axial diffusive flux compared to the radial diffusive flux