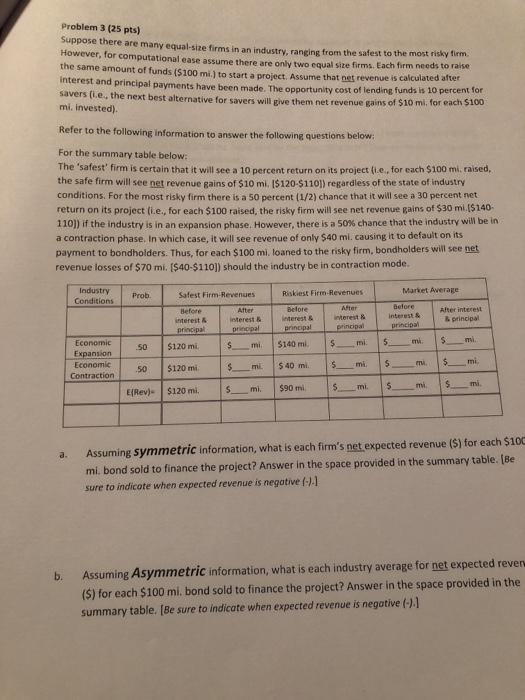
Problem 3 (25 pts) Suppose there are many equal-size firms in an industry ranging from the safest to the most risky firm However, for computational ease assume there are only two equal size firms. Each firm needs to raise the same amount of funds ($100 mil to start a proiect. Assume that net revenue is calculated after interest and principal payments have been made. The opportunity cost of lending funds is 10 percent for savers (i.e., the next best alternative for savers will give them net revenue gains of $10 mi. for each $100 mi. Invested). Refer to the following information to answer the following questions below: For the summary table below: The 'safest' firm is certain that it will see a 10 percent return on its project lie., for each $100 mi. raised, the safe firm will see net revenue gains of $10 mi. 15120-$110]) regardless of the state of industry conditions. For the most risky firm there is a 50 percent (1/2) chance that it will see a 30 percent net return on its project i.e., for each $100 raised, the risky firm will see net revenue gains of $30 ml($140- 110)) if the industry is in an expansion phase. However, there is a 50% chance that the industry will be in a contraction phase. In which case, it will see revenue of only $40 ml. causing it to default on its payment to bondholders. Thus, for each $100 mi. loaned to the risky firm, bondholders will see net revenue losses of $70 mi. [$40-$110]) should the industry be in contraction mode. Industry Conditions Prob. Safest Firm-Revenues Riskiest FirmRevenues Market Average After interest & principal interest & Interest & Before interest & principal leyterest Alter interest & principal Economic5 0 $120 mi. $_mi. $140 mi. $ mi $_ mi. $_ mi. Expansion Economic Contraction 50 $120 mi. $_ mi. $_ mi. $ 40 mi. $90 mi. $__mi. mi. $_ $120 mi. mi. E(Rev)- S $ mi $ mi. a. Assuming symmetric information, what is each firm's net expected revenue (S) for each $100 mi. bond sold to finance the project? Answer in the space provided in the summary table. (Be sure to indicate when expected revenue is negotive (-).] b. Assuming Asymmetric information, what is each industry average for net expected reven (s) for each $100 mi. bond sold to finance the project? Answer in the space provided in the summary table. (Be sure to indicate when expected revenue is negative (-).] C. Assuming asymmetric information, what promised payment (E (rev) in $mi.) will savers require for each $100 mi. bond sold by any firm in the industry?! d. Assuming symmetric information, what expected payment in $mi.) from expansion would savers require for each $ 100 mi. bond sold by the most risky firm? (Assume the expected payment during the contraction firm is constant.) e. Under asymmetric information, which firms would initially end up selling bonds based on savers expected net return of 10 percent? What logical conclusions follow? Explain thoroughly? Problem 3 (25 pts) Suppose there are many equal-size firms in an industry ranging from the safest to the most risky firm However, for computational ease assume there are only two equal size firms. Each firm needs to raise the same amount of funds ($100 mil to start a proiect. Assume that net revenue is calculated after interest and principal payments have been made. The opportunity cost of lending funds is 10 percent for savers (i.e., the next best alternative for savers will give them net revenue gains of $10 mi. for each $100 mi. Invested). Refer to the following information to answer the following questions below: For the summary table below: The 'safest' firm is certain that it will see a 10 percent return on its project lie., for each $100 mi. raised, the safe firm will see net revenue gains of $10 mi. 15120-$110]) regardless of the state of industry conditions. For the most risky firm there is a 50 percent (1/2) chance that it will see a 30 percent net return on its project i.e., for each $100 raised, the risky firm will see net revenue gains of $30 ml($140- 110)) if the industry is in an expansion phase. However, there is a 50% chance that the industry will be in a contraction phase. In which case, it will see revenue of only $40 ml. causing it to default on its payment to bondholders. Thus, for each $100 mi. loaned to the risky firm, bondholders will see net revenue losses of $70 mi. [$40-$110]) should the industry be in contraction mode. Industry Conditions Prob. Safest Firm-Revenues Riskiest FirmRevenues Market Average After interest & principal interest & Interest & Before interest & principal leyterest Alter interest & principal Economic5 0 $120 mi. $_mi. $140 mi. $ mi $_ mi. $_ mi. Expansion Economic Contraction 50 $120 mi. $_ mi. $_ mi. $ 40 mi. $90 mi. $__mi. mi. $_ $120 mi. mi. E(Rev)- S $ mi $ mi. a. Assuming symmetric information, what is each firm's net expected revenue (S) for each $100 mi. bond sold to finance the project? Answer in the space provided in the summary table. (Be sure to indicate when expected revenue is negotive (-).] b. Assuming Asymmetric information, what is each industry average for net expected reven (s) for each $100 mi. bond sold to finance the project? Answer in the space provided in the summary table. (Be sure to indicate when expected revenue is negative (-).] C. Assuming asymmetric information, what promised payment (E (rev) in $mi.) will savers require for each $100 mi. bond sold by any firm in the industry?! d. Assuming symmetric information, what expected payment in $mi.) from expansion would savers require for each $ 100 mi. bond sold by the most risky firm? (Assume the expected payment during the contraction firm is constant.) e. Under asymmetric information, which firms would initially end up selling bonds based on savers expected net return of 10 percent? What logical conclusions follow? Explain thoroughly