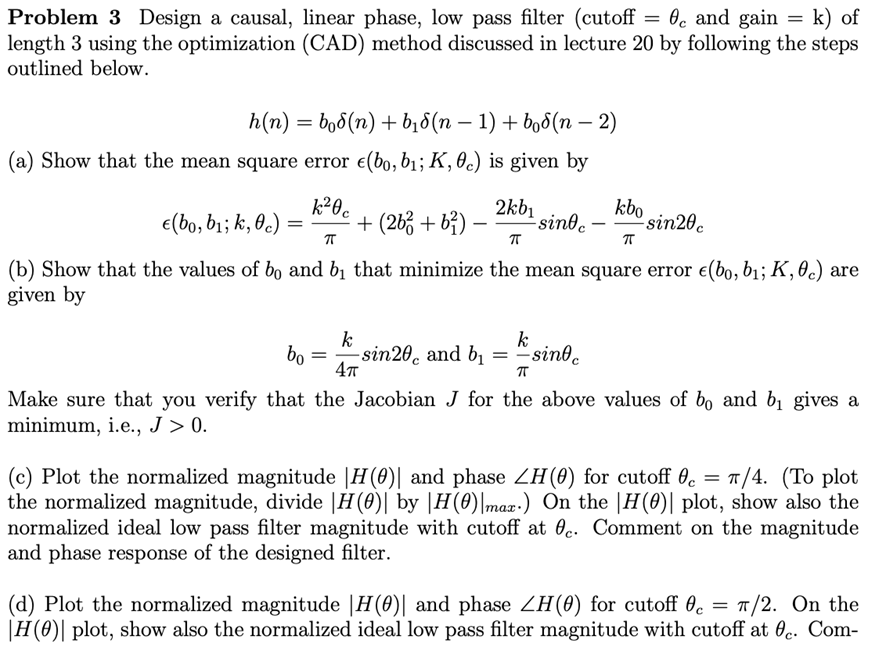
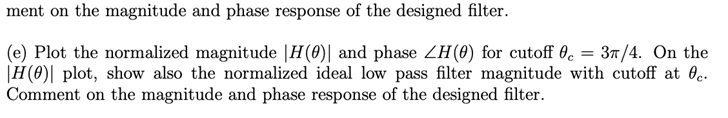
= k) of Problem 3 Design a causal, linear phase, low pass filter (cutoff = 0c and gain length 3 using the optimization (CAD) method discussed in lecture 20 by following the steps outlined below. h(n) = bod(n) + bd(n 1) + bod(n 2) (a) Show that the mean square error (bo, b; K, 0c) is given by k0c 2kb1 + (262 +6) -- sinec -sin20c T (b) Show that the values of bo and b that minimize the mean square error e(bo, b; K, 0c) are given by (bo, b; k, 0c) = k k 4 -sinec Make sure that you verify that the Jacobian J for the above values of bo and b gives a minimum, i.e., J> 0. bo = -sin20 and b kbo = = (c) Plot the normalized magnitude H(0)| and phase ZH(0) for cutoff c /4. (To plot the normalized magnitude, divide |H(0)| by |H(0)|max.) On the |H(0)| plot, show also the normalized ideal low pass filter magnitude with cutoff at c. Comment on the magnitude and phase response of the designed filter. = (d) Plot the normalized magnitude |H(0)| and phase ZH(0) for cutoff Oc /2. On the |H(0)| plot, show also the normalized ideal low pass filter magnitude with cutoff at 0c. Com- ment on the magnitude and phase response of the designed filter. (e) Plot the normalized magnitude | H(0)| and phase ZH(0) for cutoff 0e = 3/4. On the H(0) plot, show also the normalized ideal low pass filter magnitude with cutoff at 0c. Comment on the magnitude and phase response of the designed filter.