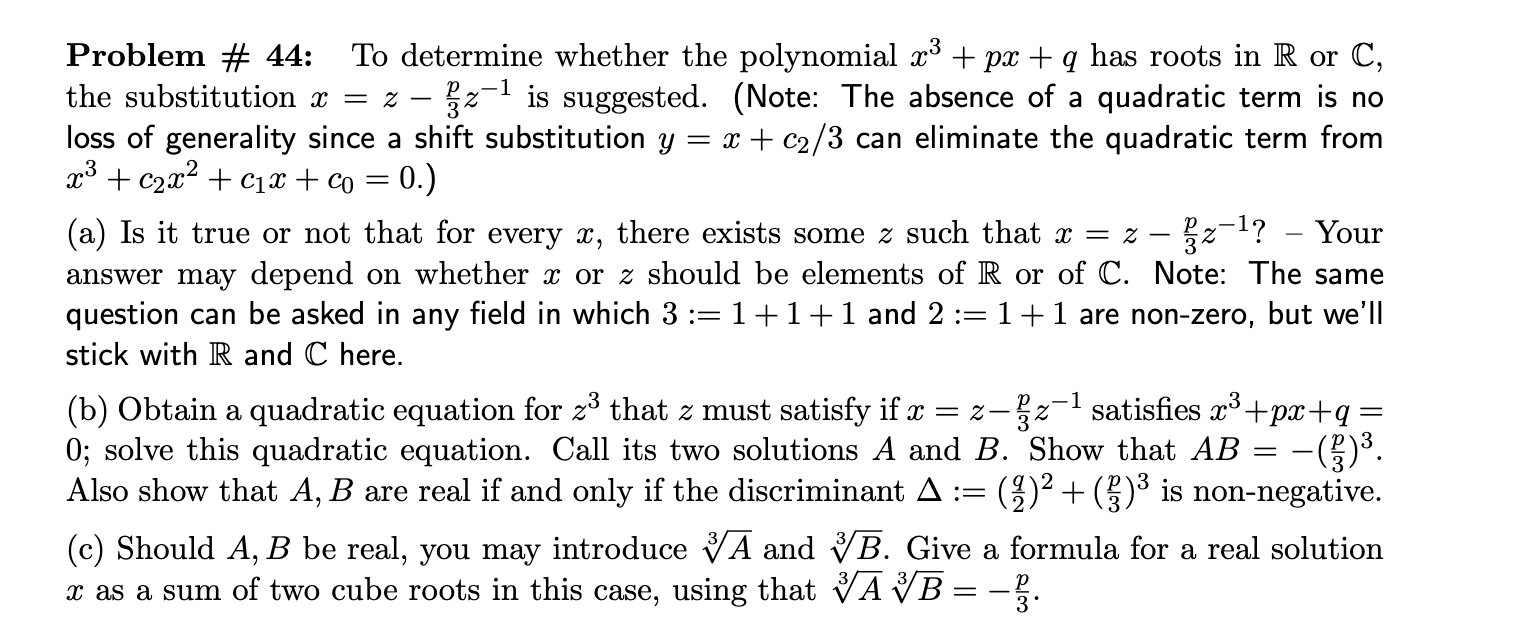
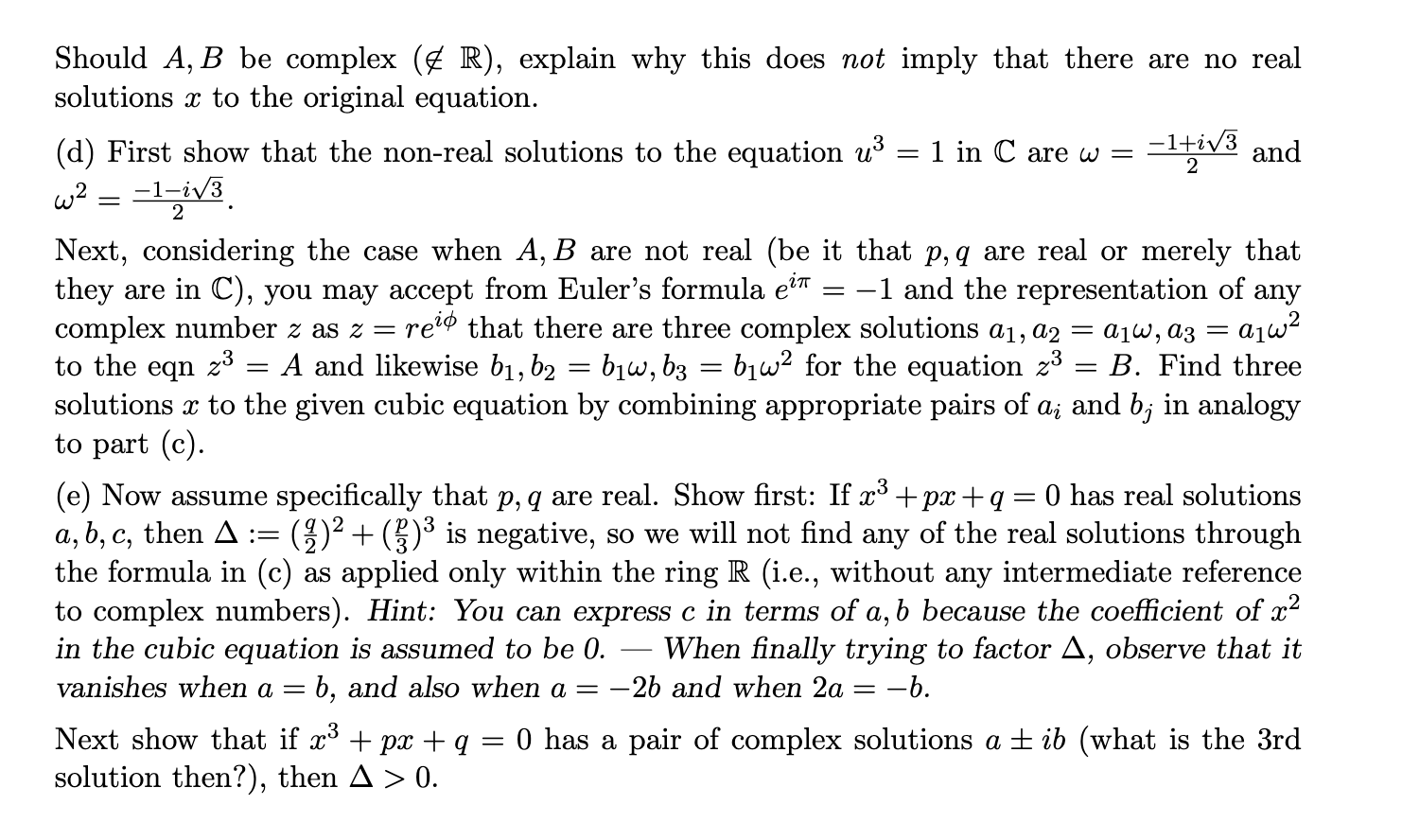
Problem # 44: To determine Whether the polynomial {123 -l- pa: -l- q has roots in IR or C, the substitution at = z 24 is suggested. (Note: The absence of a quadratic term is no loss of generality since a shift substitution y = :1: + 02/3 can eliminate the quadratic term from 3:3 +0212 +c133+co = O.) (a) Is it true or not that for every 3:, there exists some z such that a: = z E's1? Your answer may depend on whether cc or 2 should be elements of R or of (C. Note: The same question can be asked in any field in which 3 := 1 + 1 + 1 and 2 := 1 + 1 are non-zero. but we'll stick with R and (I here. (b) Obtain a quadratic equation for 23 that z must satisfy if as = z z_1 satises 2:3 +p$+q = 0; solve this quadratic equation. Call its two solutions A and B. Show that AB = ('3')3. Also show that A, B are real if and only if the discriminant A := (g)2 + ($3 is non-negative. (0) Should A, B be real, you may introduce \\B/Z and 3/5. Give a formula for a real solution a: as a sum of two cube roots in this case, using that \\3/3 {'7 = '. Should A,B be complex (g? R), explain why this does not imply that there are no real solutions :1: to the original equation. ((1) First show that the non-real solutions to the equation 11,3 = 1 in (C are to = 44'ng and w2 = 4113 2 Next, considering the case when A, B are not real (be it that p, q are real or merely that they are in C), You may accept from Euler's formula 6'\" = 1 and the representation of any complex number 2 as z = re"?5 that there are three complex solutions (11, a2 = (110.), (13 = a1w2 to the eqn 23 = A and likewise b1, b2 = blw, ()3 = (2le for the equation 23 = B. Find three solutions :1: to the given cubic equation by combining appropriate pairs of (12- and bj in analogy to part (c). (e) Now assume specically that p, q are real. Show rst: If 3:3 +133: +q = 0 has real solutions (1,1), c, then A := (%)2 + (faU3 is negative, so we will not nd any of the real solutions through the formula in (c) as applied only within the ring R (i.e., Without any intermediate reference to complex numbers). Hint: You can express c in terms of a, I) because the coefcient of :32 in the cubic equation is assumed to be 0. When finally trying to factor A, observe that it vanishes when a = b, and also when a = 2b and when 2a = b. Next show that if x3 + pm + q = O has a pair of complex solutions a :l: ib (what is the 3rd solution then?), then A > 0