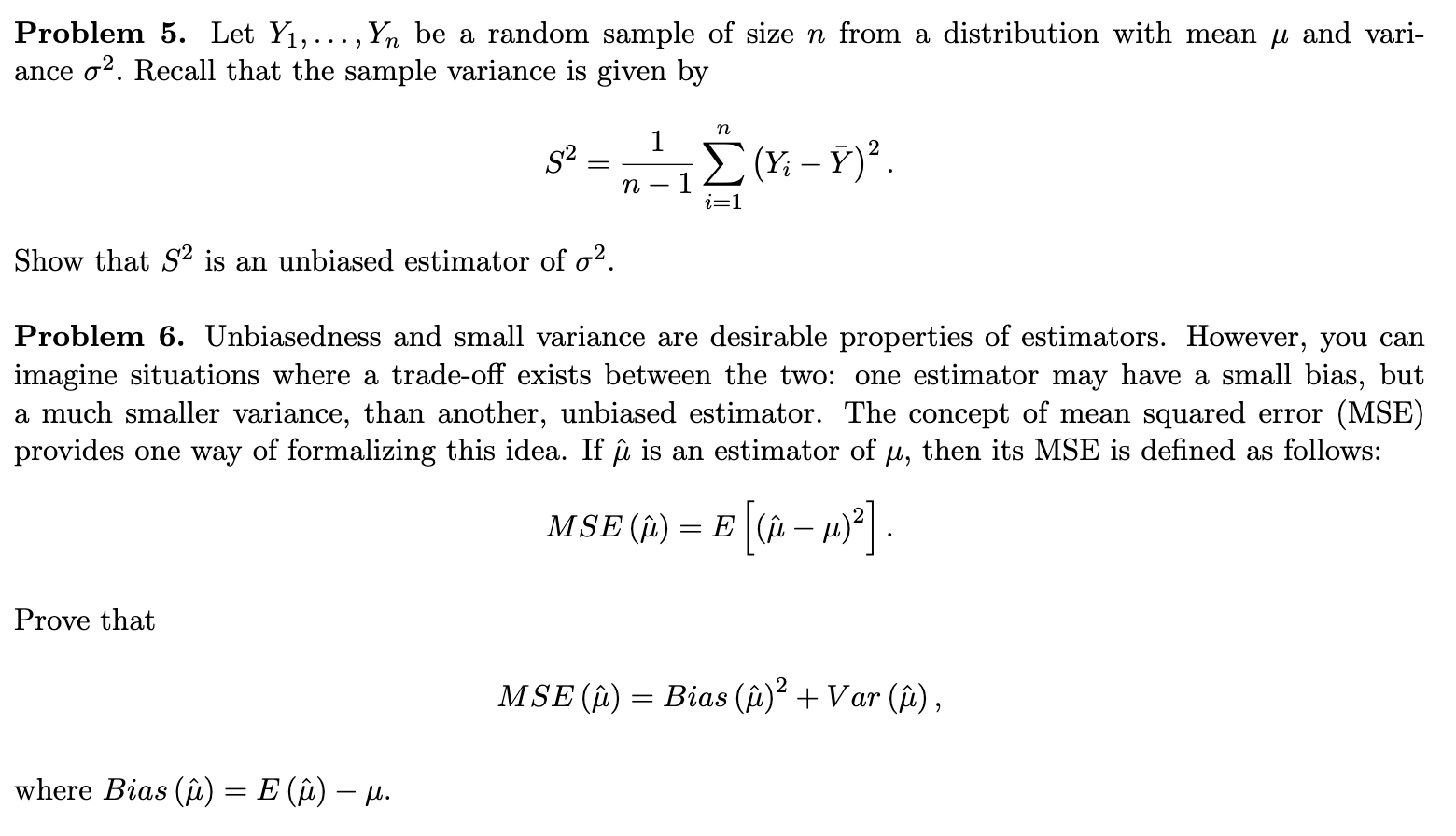
Problem 5. Let Y,..., Yn be a random sample of size n from a distribution with mean and vari- ance 2. Recall that the sample variance is given by S == Show that S2 is an unbiased estimator of . 1 n- 1 n (Yi ). i=1 Problem 6. Unbiasedness and small variance are desirable properties of estimators. However, you can imagine situations where a trade-off exists between the two: one estimator may have a small bias, but a much smaller variance, than another, unbiased estimator. The concept of mean squared error (MSE) provides one way of formalizing this idea. If is an estimator of , then its MSE is defined as follows: MSE (i) = E [( 1)] . Prove that MSE()= = Bias (u) + Var (), where Bias (u) = E () .