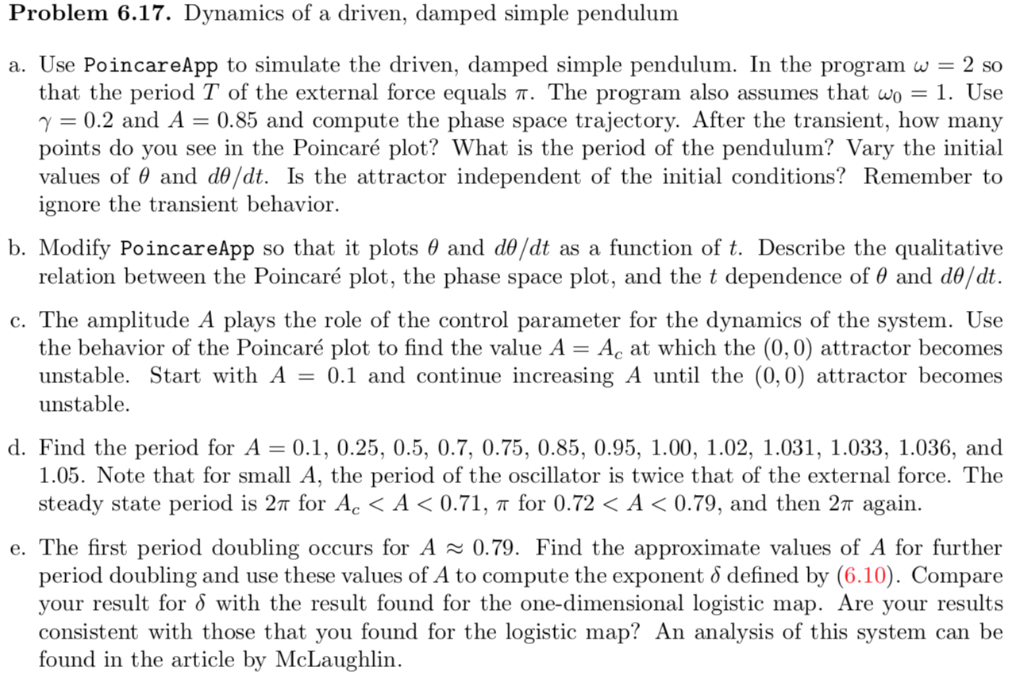
Problem 6.17 from Introduction to Computer Simulation Methods by Gould, Tobochnik, and Christian

(need to solve using Java)

Problem 6.17. Dynamics of a driven, damped simple pendulum a. Use PoincareApp to simulate the driven, damped simple pendulum. In the program w - 2 so that the period T of the external force equals . The program also assumes that wo 1. Use = 0.2 and A 0.85 and compute the phase space trajectory. After the transient, how many points do you see in the Poincar plot? What is the period of the pendulum? Vary the initial values of and d/dt. Is the attractor independent of the initial conditions? Remember to gnore the transient behavior b. Modify Poincar eApp so that it plots and de/dt as a function of t. Describe the qualitative relation between the Poincare plot, the phase space plot, and the t dependence of and dejdt c. The amplitude A plays the role of the control parameter for the dynamics of the system. Use the behavior of the Poincar plot to find the value A -Ac at which the (0, 0) attractor becomes unstable. Start with A- 0.1 and continue increasing A until the (0,0) attractor becomes unstable. d. Find the period for A = 0.1, 0.25, 0.5, 0.7, 0.75, 0.85, 0.95, 1.00, 1.02, 1.031, 1.033, 1.036, and 1.05. Note that for small A, the period of the oscillator is twice that of the external force. The steady state period is 2 for A.