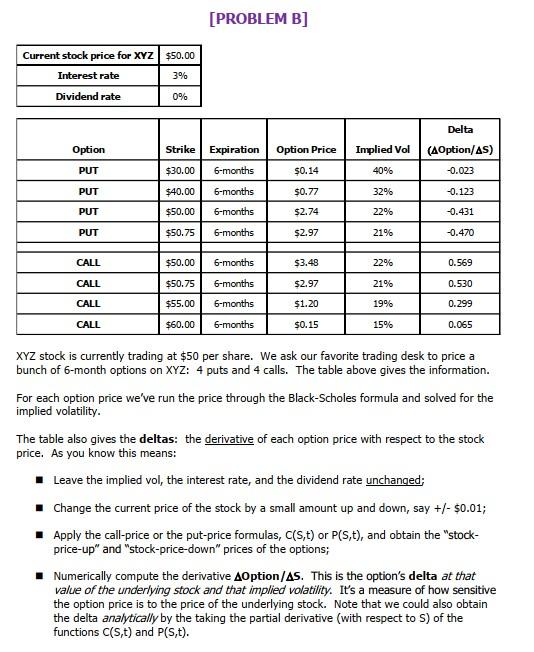
[PROBLEM B] Current stock price for XYZ $50.00 Interest rate 3% Dividend rate 0% Delta (AOption/AS) Option Option Price Implied Vol 40% PUT $0.14 -0.023 Strike Expiration $30.00 6-months $40.00 6-months $50.00 6-months PUT $0.77 32% -0.123 PUT $2.74 22% -0.431 PUT $50.75 6-months $2.97 21% -0.470 CALL $50.00 6-months $3.48 22% 0.569 CALL $50.75 6-months $2.97 21% 0.530 CALL $55.00 6-months $1.20 19% 0.299 CALL $60.00 6-months $0.15 15% 0.065 XYZ stock is currently trading at $50 per share. We ask our favorite trading desk to price a bunch of 6-month options on XYZ: 4 puts and 4 calls. The table above gives the information. For each option price we've run the price through the Black-Scholes formula and solved for the implied volatility. The table also gives the deltas: the derivative of each option price with respect to the stock price. As you know this means: Leave the implied vol, the interest rate, and the dividend rate unchanged Change the current price of the stock by a small amount up and down, say +/- $0.01; Apply the call-price or the put-price formulas, C(s, t) or PCs,t), and obtain the "stock- price-up" and "stock-price-down" prices of the options; Numerically compute the derivative AOption/As. This is the option's delta at that value of the underlying stock and that implied volatility. It's a measure of how sensitive the option price is to the price of the underlying stock. Note that we could also obtain the delta analytically by the taking the partial derivative (with respect to S) of the functions C(s, t) and P(S,t). [1] Suppose you buy the $40-strike put at the offer price of $0.77. You come in the next day and see that the stock price has fallen to $44. Will the put price have gone up or down? [2] Say you bought the $40-strike put (at $0.77); you decide to sell it back after the price of the stock has fallen to $44. Would the trader bid a price corresponding to 32% implied volatility (which is what you "paid" back when the stock was at $50) ... or would they bid at a higher or lower implied volatility? Explain. [3] Why is the delta negative for all the puts and positive for all the calls? [4] The $55-strike call is priced at $1.20. Suppose the price of the stock moved up from $50 to $52. For this $55-strike call... what would be its (approximate) updated price if it got priced at the same implied volatility (19%) as before? [5] Suppose you bought the $40-strike put and the $60-strike call. This would cost you $0.77 + $0.15 = $0.92. Please draw a graph that shows the value of this combined position at the expiration date. The x-axis will be S(T), the value of the stock at expiration; the y-axis will be the value of the position (put and call). [PROBLEM B] Current stock price for XYZ $50.00 Interest rate 3% Dividend rate 0% Delta (AOption/AS) Option Option Price Implied Vol 40% PUT $0.14 -0.023 Strike Expiration $30.00 6-months $40.00 6-months $50.00 6-months PUT $0.77 32% -0.123 PUT $2.74 22% -0.431 PUT $50.75 6-months $2.97 21% -0.470 CALL $50.00 6-months $3.48 22% 0.569 CALL $50.75 6-months $2.97 21% 0.530 CALL $55.00 6-months $1.20 19% 0.299 CALL $60.00 6-months $0.15 15% 0.065 XYZ stock is currently trading at $50 per share. We ask our favorite trading desk to price a bunch of 6-month options on XYZ: 4 puts and 4 calls. The table above gives the information. For each option price we've run the price through the Black-Scholes formula and solved for the implied volatility. The table also gives the deltas: the derivative of each option price with respect to the stock price. As you know this means: Leave the implied vol, the interest rate, and the dividend rate unchanged Change the current price of the stock by a small amount up and down, say +/- $0.01; Apply the call-price or the put-price formulas, C(s, t) or PCs,t), and obtain the "stock- price-up" and "stock-price-down" prices of the options; Numerically compute the derivative AOption/As. This is the option's delta at that value of the underlying stock and that implied volatility. It's a measure of how sensitive the option price is to the price of the underlying stock. Note that we could also obtain the delta analytically by the taking the partial derivative (with respect to S) of the functions C(s, t) and P(S,t). [1] Suppose you buy the $40-strike put at the offer price of $0.77. You come in the next day and see that the stock price has fallen to $44. Will the put price have gone up or down? [2] Say you bought the $40-strike put (at $0.77); you decide to sell it back after the price of the stock has fallen to $44. Would the trader bid a price corresponding to 32% implied volatility (which is what you "paid" back when the stock was at $50) ... or would they bid at a higher or lower implied volatility? Explain. [3] Why is the delta negative for all the puts and positive for all the calls? [4] The $55-strike call is priced at $1.20. Suppose the price of the stock moved up from $50 to $52. For this $55-strike call... what would be its (approximate) updated price if it got priced at the same implied volatility (19%) as before? [5] Suppose you bought the $40-strike put and the $60-strike call. This would cost you $0.77 + $0.15 = $0.92. Please draw a graph that shows the value of this combined position at the expiration date. The x-axis will be S(T), the value of the stock at expiration; the y-axis will be the value of the position (put and call)