Answered step by step
Verified Expert Solution
Question
1 Approved Answer
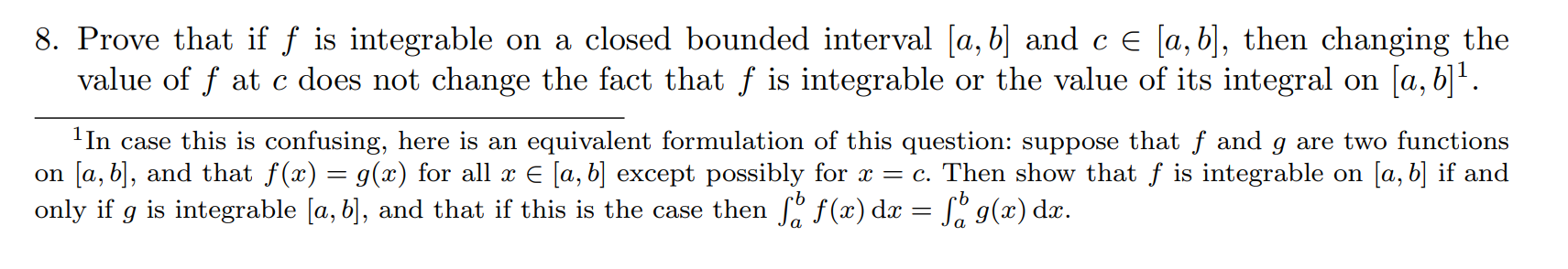
Prove that if f is integrable on a closed bounded interval a,b and cin[a,b] , then changing the value of f at c does not
Prove that if
fis integrable on a closed bounded interval
a,band
cin[a,b], then changing the\ value of
fat
cdoes not change the fact that
fis integrable or the value of its integral on
[a,b]^(1).\
^(1)In case this is confusing, here is an equivalent formulation of this question: suppose that
fand
gare two functions\ on
a,b, and that
f(x)=g(x)for all
xin[a,b]except possibly for
x=c. Then show that
fis integrable on
a,bif and\ only if
gis integrable
a,b, and that if this is the case then
\\\\int_a^b f(x)dx=\\\\int_a^b g(x)dx.
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


