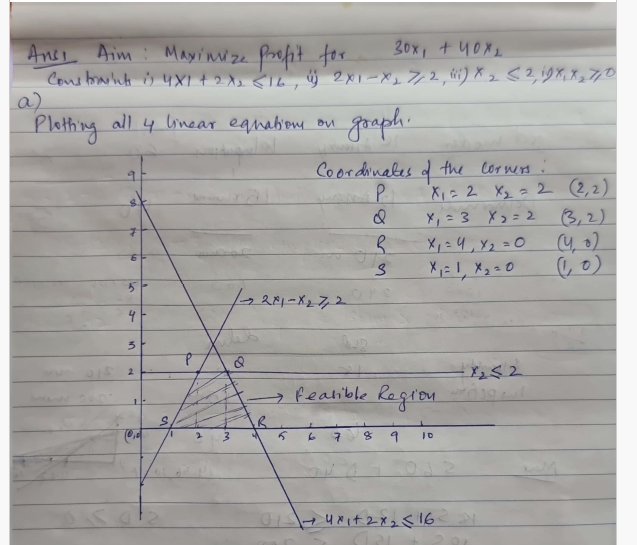
Q1. Solve the following problem, using the Corner Point Method. Maximize profit = 30x1 + 40x2 Subject to 4x1 + 2x2 ? 16 2x1 - x2 ? 2 x2 ? 2 x1, x2 ? 0 a) Draw lines graphically and show the feasible region and corner points on your figure. (10 Points) b) Find the optimal value of decision variables and calculate profit at the optimal point. (10 Points) c) For the optimal solution, how much slack or surplus is there for each? (5 points)

============================================================================

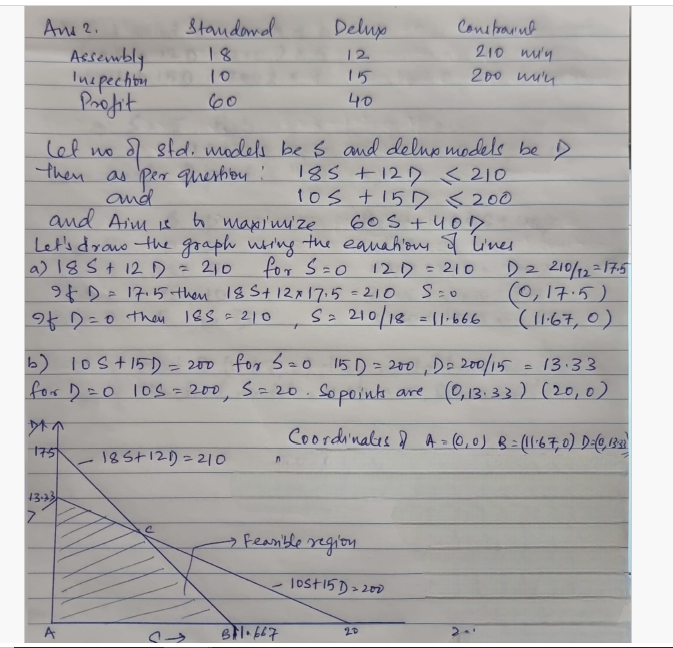
images q1 and q2 for the Q1

======================================================================================= Q2. Billy Penny is trying to determine how many units of two types of lawn mowers to produce each day. One of these is the Standard model, while the other is the Deluxe model. The profit per unit on the Standard model is $60, while the profit per unit on the Deluxe model is $40. The Standard model requires 18 minutes of assembly time, while the Deluxe model requires 12 minutes of assembly time. The Standard model requires 10 minutes of inspection time, while the Deluxe model requires 15 minutes of inspection time. There are 210 minutes of assembly time and 200 minutes of inspection time available each day. a) Formulate the linear programming problem. (10 Points) b) How many units of each product should be manufactured to maximize profits using the Corner Point Method or Isoprofit Line Solution Method? (15 Points)

======================================================================================

\f\fAnd 2. Standard Deluxe Assembly 18 12 210 MM'M Inspection 10 145 200 Wy Profit 60 40 ( of no of stad. models be s and deluxemodels be is them as per question : 185 + 121 5 210 and 105 + 151 5 200 and Aim is to maximize 60 S + 40D Let's draws the graph using the equations of lives a ) 18 5+ 12 1 = 210 for 5= 0 121 = 210 1 2 210/ 12=17:5 9 + D = 17.5 them 18 5+ 12x 17. 5 = 210 5= 0 10 , 17 .5 ) 9* D = 0 Then 185 = 210 ,5= 210/18 = 11-666 ( 11.67 , 0 ) b ) 10 5 + 151 = 200 for 5=0 15 0 = 280, D= 200/15 = 13.33 for D = 0 105 = 200, 5 = 20. Sopoints are ( 0, 13 . 3 3 ) ( 20, 0) Coordinates ? A = 10, 0 ) B= (11 67, 0 ) 1 :(0, 13:3) 175 - 185+ 121= 210 Feasible region - 105+ 15 D) = 250 ATo find point I which is on both lines we are elimination ( 1 8 5 + 12 0 = 21 0 ) 15 = 905+ 601 = 7050 (10 5 + 1450) = 200 ) * 4 = 40 5 + 601 : 800 505 - 250 3 5 : 5, Replacing S in 185+ P120 = 210 9 0 + 12 0 = 210 2 12 D = 120 D= 10 coordinate of point c = (5, 10 ) S D Profit = 605+ 401 Point A O Point B 11:67 0 60* 11. 67+0 = 700.2 - Point e 5 10 60 *5 + 40 * 10 = 700 Point ) 0 13:33 0X 60+ 40x13:33 = 533. 2 Since at point B (11. 67, 0) they would have may profit= 7002 So no of standard model = 11:67 in 12, Deluxmodel = zero