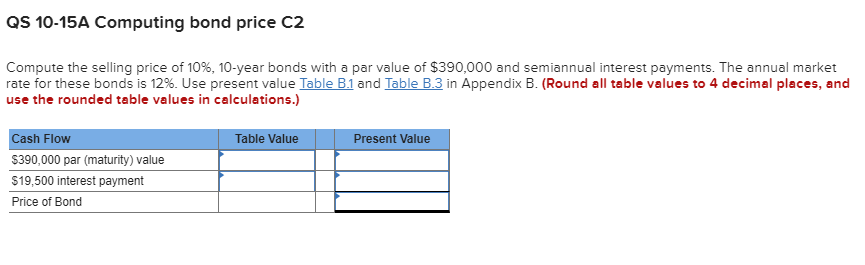
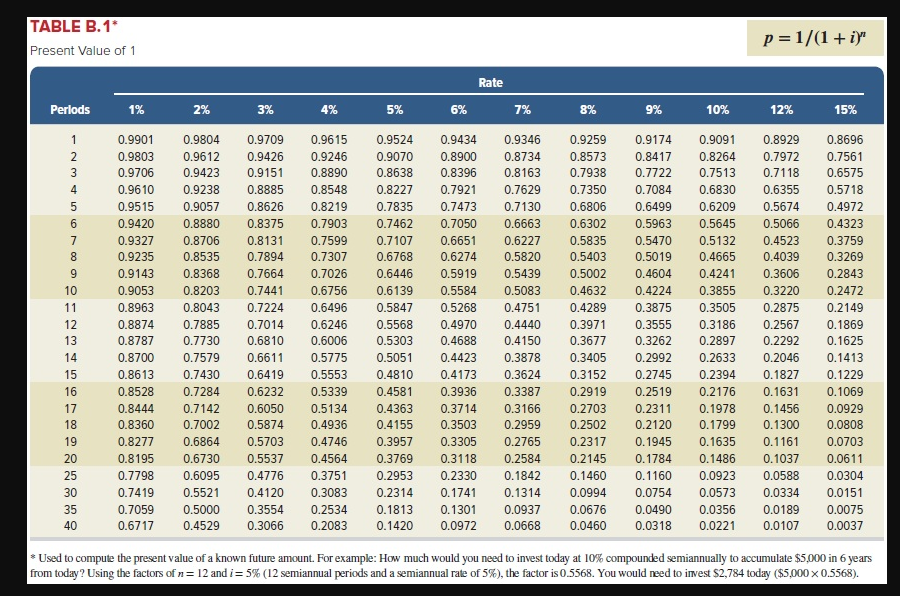
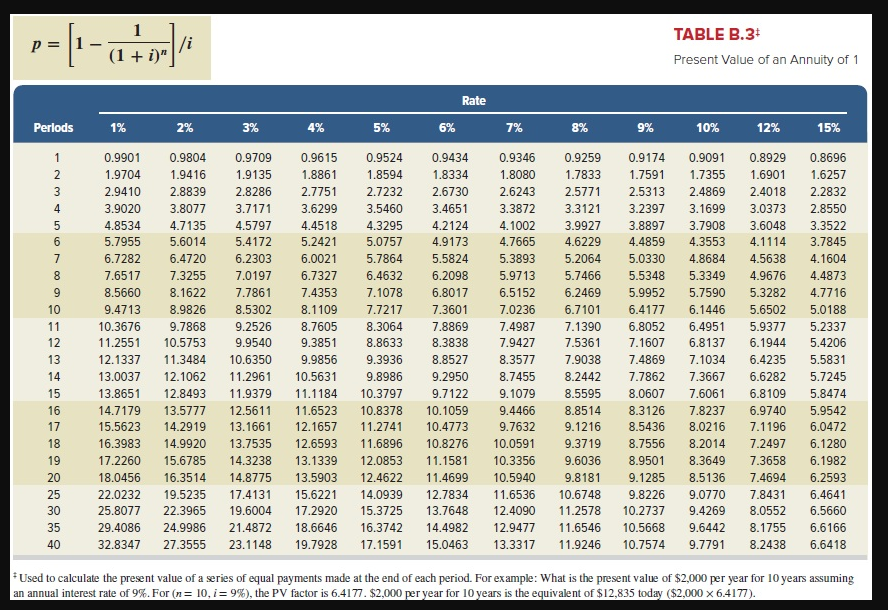
QS 10-15A Computing bond price C2 Compute the selling price of 10%, 10-year bonds with a par value of $390,000 and semiannual interest payments. The annual market rate for these bonds is 12%. Use present value Table B.1 and Table B.3 in Appendix B. (Round all table values to 4 decimal places, and use the rounded table values in calculations.) Table Value Present Value Cash Flow $390,000 par (maturity) value $19,500 interest payment Price of Bond TABLE B.1* Present Value of 1 p=1/(1 + i)" Rate Perlods 1% 2% 3% 4% 5% 6% 7% 8% 9% 10% 12% 15% vouw 0.9524 0.9070 0.8638 0.8227 0.7835 0.7462 0.9901 0.9804 0.97090.9615 0.98030.9612 0.9426 0.9246 0.9706 0.9423 0.9151 0.8890 0.9610 0.9238 0.8885 0.8548 0.9515 0.9057 0.8626 0.8219 0.9420 0 .8880 0.8375 0.7903 0.9327 0.8706 0.8131 0.7599 0.9235 0.8535 0.7894 0.7307 0.9143 0 .8368 0.7664 0.7026 0.9053 0.8203 0.7441 0.6756 0.8963 0.8043 0.7224 0.6496 0.8874 0.7885 0.7014 0.6246 0.8787 0.7730 0.6810 0.6006 0.8700 0.7579 0.6611 0.5775 0.8613 0.7430 0.6419 0.5553 0.8528 0.7284 0.6232 0.5339 0.8444 0.7142 0.6050 0.5134 0.8360 0.7002 0.5874 0.4936 0.8277 0.6864 0.5703 0.4746 0.8195 0.6730 0.5537 0.4564 0.7798 0.6095 0.4776 0.3751 0.7419 0.5521 0.4120 0.3083 0.7059 0 .5000 0.3554 0.2534 0.6717 0.45290.30660.2083 0.6768 0.6446 0.6139 0.5847 0.5568 0.5303 0.5051 0.4810 0.4581 0.4363 0.4155 0.3957 0.3769 0.2953 0.2314 0.1813 0.1420 0.9434 0.8900 0.8396 0.7921 0.7473 0.7050 0.6651 0.6274 0.5919 0.5584 0.5268 0.4970 0.4688 0.4423 0.4173 0.3936 0.3714 0.3503 0.3305 0.3118 0.2330 0.1741 0.1301 0.0972 0.9346 0.8734 0.8163 0.7629 0.7130 0.66630 0.6227 0.5820 0.5439 0.5083 0.4751 0.4440 0.4150 0.3878 0.3624 0.3387 0.3166 0.2959 0.2765 0.2584 0.1842 0.1314 0.0937 0.0668 0.9259 0.9174 0.8573 0.8417 0.7938 0.7722 0.7350 0.7084 0.6806 0.6499 .6302 0.5963 0.5835 0.5470 0.5403 0.5019 0.5002 0.4604 0.4632 0.4224 0.4289 0.3875 0.3971 0.3555 0.3677 0.3262 0.3405 0.2992 0.3152 0.2745 0.2919 0.2519 0.2703 0.2311 0.2502 0.2120 0.2317 0.1945 0.2145 0.1784 0.1460 0.1160 0.0994 0.0754 0.0676 0.0490 0.04600.0318 0.9091 0.8264 0.7513 0.6830 0.6209 0.5645 05132 0.4665 0.4241 0.3855 0.3505 0.3186 0.2897 0.2633 0.2394 0.2176 0.1978 0.1799 0.1635 0.1486 0.0923 0.0573 0.0356 0.0221 0.89290.8696 0.7972 0.7561 0.7118 0.6575 0.6355 0.5718 0.5674 0.4972 0.5066 0.3759 0.4039 0.3269 0.3606 0.2843 0.3220 0.2472 0.2875 0.2149 0.2567 0.1869 0.2292 0.1625 0.2046 0.1413 0.1827 0.1229 0.1631 0.1069 0.1456 0.0929 0.1300 0.0808 0.1161 0.0703 0.1037 0.0611 0.0588 0.0304 0.0334 0.0151 0.0189 0.0075 0.0107 0.0037 * Used to compute the present value of a known future amount. For example: How much would you need to invest today at 10% compounded semiannually to accumulate $5,000 in 6 years from today? Using the factors of n= 12 and i = 5% (12 semiannual periods and a semiannual rate of 5%), the factor is 0.5568. You would need to invest $2,784 today ($5,000 x 0.5568). TABLE B.3 P=[1-1 +] Present Value of an Annuity of 1 Rate Periods 1% 2% 3% 4% 5% 6% 7% 8% 9% 10% 12% 15% WN O O O O 0.9901 1.9704 2.9410 3.9020 4.8534 5.7955 6.7282 7.6517 8.5660 9.4713 10.3676 11.2551 12.1337 13.0037 13.8651 14.7179 15.5623 16.3983 17.2260 18.0456 22.0232 25.8077 29.4086 32.8347 0.9804 0.9709 1.9416 1.9135 2.8839 2.8286 3.8077 3.7171 4.7135 4.5797 5.6014 5.4172 6.4720 6.2303 7.3255 7.0197 8.1622 7.7861 8.9826 8.5302 9.7868 9.2526 10.5753 9.9540 11.3484 10.6350 12.1062 11.2961 12.8493 11.9379 13.5777 12.5611 14.291913.1661 14.9920 13.7535 15.6785 14.3238 16.3514 14.8775 19.5235 17.4131 22.3965 19.6004 24.9986 21.4872 27.3555 23.1148 0.9615 1.8861 2.7751 3.6299 4.4518 5.2421 6.0021 6.7327 7.4353 8.1109 8.7605 9.3851 9.9856 10.5631 11.1184 11.6523 12.1657 12.6593 13.1339 13.5903 15.6221 17.2920 18.6646 19.7928 0.9524 0.9434 1.8594 1.8334 2.7232 2.6730 3.5460 3.4651 4.3295 4.2124 5.0757 4.9173 5.7864 5.5824 6.4632 6.2098 7.1078 6.8017 7.7217 7.3601 8.3064 7.8869 8.8633 8.3838 9.3936 8.8527 9.8986 9.2950 10.3797 9.7122 10.8378 10.1059 11.2741 10.4773 11.6896 10.8276 12.085311.1581 12.4622 11.4699 14.093912.7834 15.3725 13.7648 16.3742 14.4982 17.1591 15.0463 0.9346 1.8080 2.6243 3.3872 4.1002 4.7665 5.3893 5.9713 6.5152 7.0236 7.4987 7.9427 8.3577 8.7455 9.1079 9.4466 9.7632 10.0591 10.3356 10.5940 11.6536 12.4090 12.9477 13.3317 0.9259 0.9174 0.9091 0.8929 0.8696 1.78331.75911.7355 1.6901 1.6257 2.5771 2.53132.48692.4018 2.2832 3.3121 3.2397 3.16993.0373 2.8550 3.9927 3.8897 3.7908 3.6048 3.3522 4.6229 4.48594.3553 4.1114 3.7845 5.2064 5.0330 4.8684 4.5638 4.1604 5.7466 5.5348 5.33494.9676 4.4873 6.2469 5.9952 5.7590 5.3282 4.7716 6.7101 6.4177 6.1446 5.6502 5.0188 7.1390 6.80526.4951 5.9377 5.2337 7.5361 7.1607 6.8137 6.1944 5.4206 7.9038 7.48697.1034 6.4235 5.5831 8.2442 7.7862 7.3667 6.6282 5.7245 8.5595 8.0607 7.6061 6.81095.8474 8.8514 8.3126 7.8237 6.9740 5.9542 9.1216 8.5436 8.0216 7.1196 6.0472 9.3719 8.7556 8.2014 7.2497 6.1280 9.6036 8.9501 8.36497.3658 6.1982 9.8181 9.1285 8.5136 7.4694 6.2593 10.6748 9.82269.0770 7.8431 6.4641 11.2578 10.27379.42698.0552 6.5660 11.6546 10.5668 9.6442 8.1755 6.6166 11.9246 10.7574 9.77918.2438 6.6418 14 *Used to calculate the present value of a series of equal payments made at the end of each period. For example: What is the present value of $2,000 per year for 10 years assuming an annual interest rate of 9%. For (n = 10,i = 9%), the PV factor is 6.4177. $2,000 per year for 10 years is the equivalent of $12,835 today ($2,000 X 6.4177)