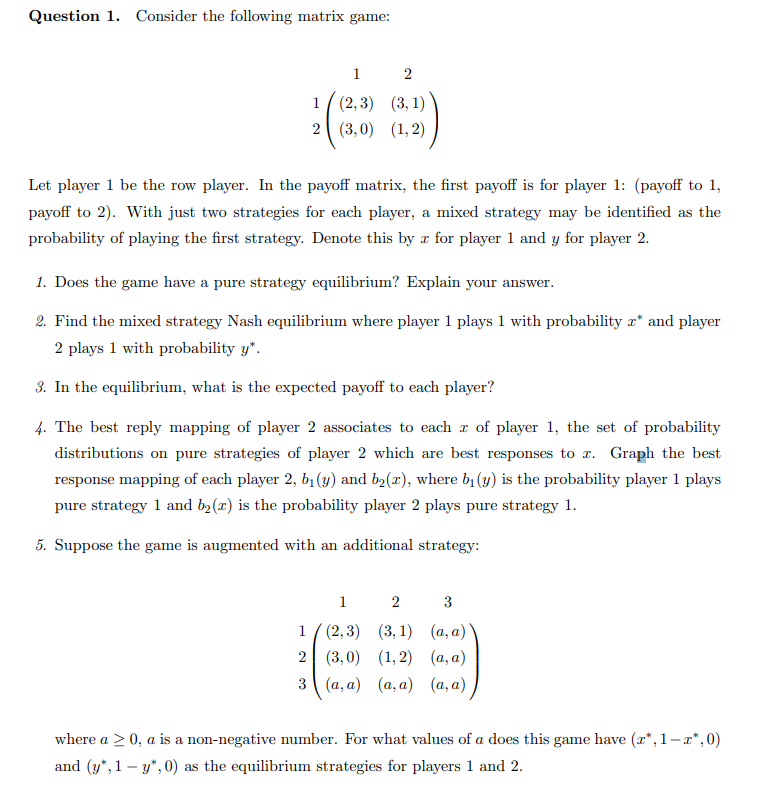
Question 1. Consider the following matrix game: 1 2 1 2 (2,3) (3,1) (3,0) (1,2) Let player 1 be the row player. In the payoff matrix, the first payoff is for player 1: (payoff to 1, payoff to 2). With just two strategies for each player, a mixed strategy may be identified as the probability of playing the first strategy. Denote this by z for player 1 and y for player 2. 1. Does the game have a pure strategy equilibrium? Explain your answer. 2. Find the mixed strategy Nash equilibrium where player 1 plays 1 with probability r* and player 2 plays 1 with probability y*. 3. In the equilibrium, what is the expected payoff to each player? 4. The best reply mapping of player 2 associates to each z of player 1, the set of probability distributions on pure strategies of player 2 which are best responses to x. Graph the best response mapping of each player 2, b (y) and b(x), where b (y) is the probability player 1 plays pure strategy 1 and b(x) is the probability player 2 plays pure strategy 1. 5. Suppose the game is augmented with an additional strategy: 1 2 (2,3) (3, 1) 2 (3,0) (1,2) 3 (a, a) (a, a) 3 (a, a)` (a, a) (a, a) where a > 0, a is a non-negative number. For what values of a does this game have (z*, 1-x*, 0) and (y*, 1-y*, 0) as the equilibrium strategies for players 1 and 2.