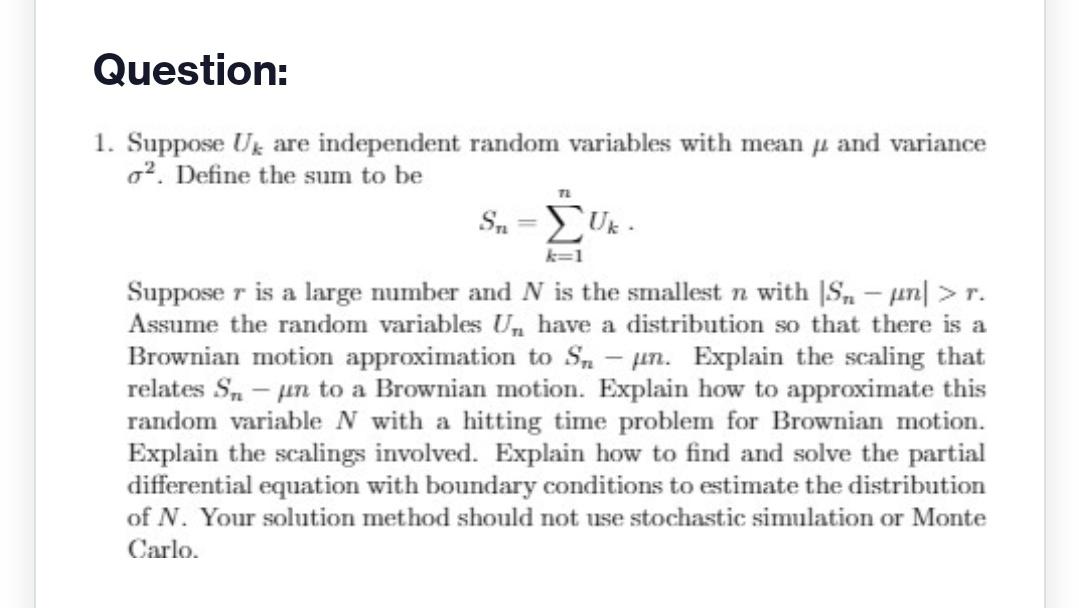
Question: 1. Suppose Uk are independent random variables with mean and variance . Define the sum to be TL Sn Uk. k=1 Suppose r is a large number and N is the smallest n with Sn- un| > r. Assume the random variables Un have a distribution so that there is a Brownian motion approximation to Sn- un. Explain the scaling that relates Sn- un to a Brownian motion. Explain how to approximate this random variable N with a hitting time problem for Brownian motion. Explain the scalings involved. Explain how to find and solve the partial differential equation with boundary conditions to estimate the distribution of N. Your solution method should not use stochastic simulation or Monte Carlo.