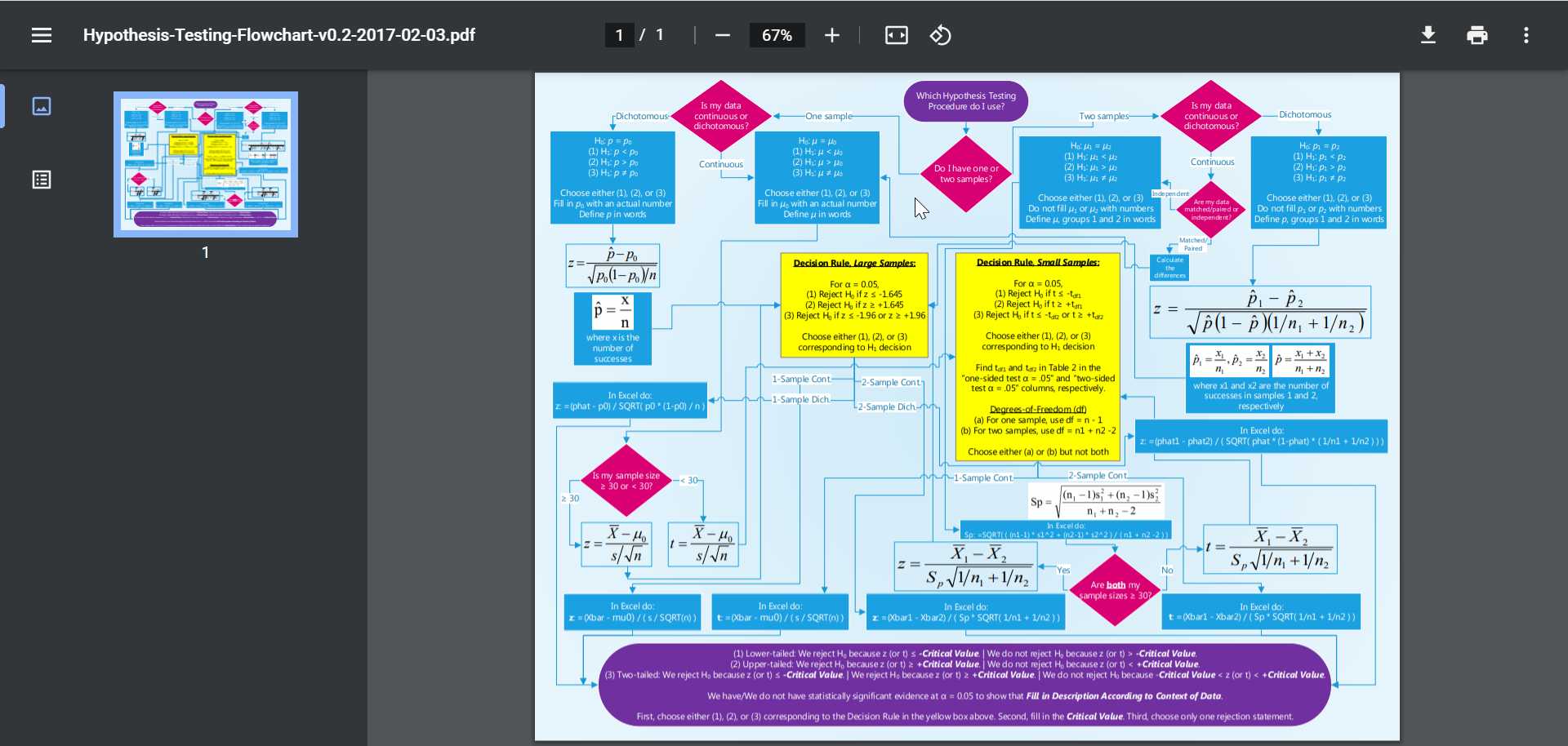
Question 11

Recall in the one sample hypothesis testing scenario that we investigated the following presumptions based on previous research, "no evidence currently exists supporting or refuting the use of electric fans during heat waves" in terms of mortality and illness, as well as Public Health guidelines suggesting not using fans during hot weather, with some research reporting the potential of fans accelerating body heating.

You decide to further your research project by hypothesizing that the true proportion of core body temperature increases amidst higher ambient temperature and humidity levels for the population who do not use electric fans is less than those who do use electric fans, setting the level of significance at 5% for the formal hypothesis test. In other words, you extend your sampling to two samples instead of just one. You randomly sample 20 and 15 participants for your first and second groups, respectively, based on your research funding and for 45 minutes, all study participants sit in a chamber maintained at a temperature of 108 degrees Fahrenheit (i.e., 42 degrees Celsius) and a relative humidity of 70%. After the first 45-minute warming period, you record the participants' core body temperatures. Furthermore, for Group 2 only you place a personal sized electric fan 3 feet away with its airflow directed at a given participant's chest area, and the participants relax in this position for the next 45 minutes, whereas for Group 1 you do not provide electric fans. At the end of this 45-minute fan period, you record the core body temperatures of all participants, documenting any temperature increases as compared to the start of the time period. The following table comprises the data you collect.

Table 1. No Fans

Subject Core Body Temperature Increased?

1 0

2 1

3 0

4 1

5 0

6 0

7 0

8 0

9 0

10 0

11 0

12 0

13 0

14 0

15 1

16 0

17 1

18 0

19 0

20 0

Table 2. Fans

Subject Core Body Temperature Increased?

1 1

2 1

3 0

4 0

5 1

6 1

7 1

8 1

9 1

10 0

11 0

12 0

13 0

14 1

15 0

Per Step 1 of the 5-Steps to Hypothesis Testing, choose the appropriate null and alternative hypotheses, i.e. H0 and H1, respectively, as well as the significance level, ?, pronounced as "alpha".

Please note that 0 and 1 are defined as no and yes, respectively, which is a typical coding scheme in Public Health.

Select one:

a.

H1: p1

b.

? = 10%, H0: p1 = p2, H0: p1 ? p2

c.

H0: p1 = p2, ? = 1%, H1: p1

d.

H1: p1 ? p2, H0: p1 = p2, ? = 2.5%

show work

Question 12

Recall in the one sample hypothesis testing scenario that we investigated the following presumptions based on previous research, "no evidence currently exists supporting or refuting the use of electric fans during heat waves" in terms of mortality and illness, as well as Public Health guidelines suggesting not using fans during hot weather, with some research reporting the potential of fans accelerating body heating.

You decide to further your research project by hypothesizing that the true proportion of core body temperature increases amidst higher ambient temperature and humidity levels for the population who do not use electric fans is less than those who do use electric fans, setting the level of significance at 1% for the formal hypothesis test. In other words, you extend your sampling to two samples instead of just one. You randomly sample 16 and 25 participants for your first and second groups, respectively, based on your research funding and for 45 minutes, all study participants sit in a chamber maintained at a temperature of 108 degrees Fahrenheit (i.e., 42 degrees Celsius) and a relative humidity of 70%. After the first 45-minute warming period, you record the participants' core body temperatures. Furthermore, for Group 2 only you place a personal sized electric fan 3 feet away with its airflow directed at a given participant's chest area, and the participants relax in this position for the next 45 minutes, whereas for Group 1 you do not provide electric fans. At the end of this 45-minute fan period, you record the core body temperatures of all participants, documenting any temperature increases as compared to the start of the time period. The following table comprises the data you collect.

Table 1. No Fans

Subject Core Body Temperature Increased?

1 0

2 0

3 0

4 0

5 0

6 1

7 0

8 1

9 0

10 0

11 0

12 1

13 0

14 0

15 0

16 1

Table 2. Fans

Subject Core Body Temperature Increased?

1 0

2 0

3 0

4 0

5 1

6 0

7 0

8 0

9 1

10 0

11 1

12 1

13 0

14 0

15 0

16 1

17 0

18 0

19 0

20 0

21 1

22 1

23 0

24 1

25 1

Per Step 2 of the 5-Steps to Hypothesis Testing, choose the appropriate test statistic.

Please note that 0 and 1 are defined as no and yes, respectively, which is a typical coding scheme in Public Health.

Select one:

a.

t = (X? - 0) / (s / ?n)

b.

t = (X?1 - X?2) / [ Sp * ?(1 / n1 + 1 / n2) ], where Sp = ?( [(n1 - 1) * s12 + (n2 - 1) * s22] / [n1 + n2 - 2] )

c.

z = (X? - 0) / (s / ?n)

d.

z = (p?1 - p?2) / ?[ p? * (1 - p?) * (1 / n1 + 1 / n2) ], where p?1 = x1 / n1, p?2 = x2 / n2, p? = (x1 + x2) / (n1 + n2)

show work

Question 13

Recall in the one sample hypothesis testing scenario that we investigated the following presumptions based on previous research, "no evidence currently exists supporting or refuting the use of electric fans during heat waves" in terms of mortality and illness, as well as Public Health guidelines suggesting not using fans during hot weather, with some research reporting the potential of fans accelerating body heating.

You decide to further your research project by hypothesizing that the true proportion of core body temperature increases amidst higher ambient temperature and humidity levels for the population who do not use electric fans is less than those who do use electric fans, setting the level of significance at 10% for the formal hypothesis test. In other words, you extend your sampling to two samples instead of just one. You randomly sample 40 and 23 participants for your first and second groups, respectively, based on your research funding and for 45 minutes, all study participants sit in a chamber maintained at a temperature of 108 degrees Fahrenheit (i.e., 42 degrees Celsius) and a relative humidity of 70%. After the first 45-minute warming period, you record the participants' core body temperatures. Furthermore, for Group 2 only you place a personal sized electric fan 3 feet away with its airflow directed at a given participant's chest area, and the participants relax in this position for the next 45 minutes, whereas for Group 1 you do not provide electric fans. At the end of this 45-minute fan period, you record the core body temperatures of all participants, documenting any temperature increases as compared to the start of the time period. The following table comprises the data you collect.

Table 1. No Fans

Subject Core Body Temperature Increased?

1 1

2 0

3 1

4 1

5 0

6 0

7 1

8 0

9 0

10 0

11 0

12 0

13 0

14 0

15 0

16 0

17 0

18 0

19 0

20 0

21 0

22 0

23 0

24 0

25 1

26 0

27 0

28 0

29 0

30 0

31 0

32 0

33 0

34 1

35 0

36 0

37 0

38 0

39 0

40 1

Table 2. Fans

Subject Core Body Temperature Increased?

1 0

2 1

3 0

4 1

5 0

6 1

7 0

8 1

9 1

10 1

11 0

12 0

13 0

14 0

15 1

16 0

17 1

18 1

19 0

20 0

21 0

22 0

23 0

Per Step 3 of the 5-Steps to Hypothesis Testing, choose the appropriate decision rule.

Please note that 0 and 1 are defined as no and yes, respectively, which is a typical coding scheme in Public Health.

Select one:

a.

Reject H0 if z ? -1.282

b.

Accept H1 if t = -1.645

c.

Accept H0 if z ? -1.282

d.

Reject H0 if z ? +2.326

Question 14

Recall in the one sample hypothesis testing scenario that we investigated the following presumptions based on previous research, "no evidence currently exists supporting or refuting the use of electric fans during heat waves" in terms of mortality and illness, as well as Public Health guidelines suggesting not using fans during hot weather, with some research reporting the potential of fans accelerating body heating.

You decide to further your research project by hypothesizing that the true proportion of core body temperature increases amidst higher ambient temperature and humidity levels for the population who do not use electric fans is less than those who do use electric fans, setting the level of significance at 2.5% for the formal hypothesis test. In other words, you extend your sampling to two samples instead of just one. You randomly sample 17 and 42 participants for your first and second groups, respectively, based on your research funding and for 45 minutes, all study participants sit in a chamber maintained at a temperature of 108 degrees Fahrenheit (i.e., 42 degrees Celsius) and a relative humidity of 70%. After the first 45-minute warming period, you record the participants' core body temperatures. Furthermore, for Group 2 only you place a personal sized electric fan 3 feet away with its airflow directed at a given participant's chest area, and the participants relax in this position for the next 45 minutes, whereas for Group 1 you do not provide electric fans. At the end of this 45-minute fan period, you record the core body temperatures of all participants, documenting any temperature increases as compared to the start of the time period. The following table comprises the data you collect.

Table 1. No Fans

Subject Core Body Temperature Increased?

1 0

2 1

3 0

4 0

5 0

6 1

7 0

8 0

9 1

10 0

11 1

12 1

13 1

14 0

15 1

16 0

17 1

Table 2. Fans

Subject Core Body Temperature Increased?

1 0

2 1

3 1

4 0

5 1

6 0

7 1

8 0

9 0

10 1

11 1

12 0

13 0

14 0

15 1

16 0

17 0

18 0

19 0

20 0

21 0

22 0

23 1

24 1

25 1

26 0

27 0

28 0

29 0

30 0

31 1

32 0

33 0

34 1

35 1

36 0

37 1

38 1

39 1

40 0

41 0

42 1

Per Step 4 of the 5-Steps to Hypothesis Testing, compute the test statistic using the appropriate test statistic formula.

Please note the following: 1) 0 and 1 are defined as no and yes, respectively, which is a typical coding scheme in Public Health; 2) you may copy and paste the data into Excel to facilitate analysis; and 3) do not round your numerical answer that you submit as the online grading system is designed to mark an answer correct if your response is within a given range. In other words, the system does not take into account rounding. On the other hand, rounding is preferable when formally reporting your statistical results to colleagues.

Answer:

show work

Question 15

Recall in the one sample hypothesis testing scenario that we investigated the following presumptions based on previous research, "no evidence currently exists supporting or refuting the use of electric fans during heat waves" in terms of mortality and illness, as well as Public Health guidelines suggesting not using fans during hot weather, with some research reporting the potential of fans accelerating body heating.

You decide to further your research project by hypothesizing that the true proportion of core body temperature increases amidst higher ambient temperature and humidity levels for the population who do not use electric fans is less than those who do use electric fans, setting the level of significance at 5% for the formal hypothesis test. In other words, you extend your sampling to two samples instead of just one. You randomly sample 37 and 31 participants for your first and second groups, respectively, based on your research funding and for 45 minutes, all study participants sit in a chamber maintained at a temperature of 108 degrees Fahrenheit (i.e., 42 degrees Celsius) and a relative humidity of 70%. After the first 45-minute warming period, you record the participants' core body temperatures. Furthermore, for Group 2 only you place a personal sized electric fan 3 feet away with its airflow directed at a given participant's chest area, and the participants relax in this position for the next 45 minutes, whereas for Group 1 you do not provide electric fans. At the end of this 45-minute fan period, you record the core body temperatures of all participants, documenting any temperature increases as compared to the start of the time period. The following table comprises the data you collect.

Table 1. No Fans

Subject Core Body Temperature Increased?

1 1

2 0

3 0

4 1

5 0

6 0

7 1

8 0

9 1

10 0

11 1

12 0

13 0

14 1

15 0

16 0

17 0

18 1

19 0

20 0

21 0

22 0

23 1

24 0

25 0

26 0

27 0

28 0

29 0

30 0

31 0

32 0

33 0

34 0

35 0

36 1

37 1

Table 2. Fans

Subject Core Body Temperature Increased?

1 0

2 0

3 0

4 0

5 0

6 0

7 1

8 0

9 0

10 0

11 0

12 0

13 0

14 0

15 0

16 0

17 0

18 1

19 1

20 0

21 0

22 0

23 0

24 0

25 0

26 1

27 0

28 0

29 0

30 0

31 1

Per Step 5 of the 5-Steps to Hypothesis Testing, choose the appropriate formal and informal conclusions.

Please note the following: 1) you may copy and paste the data into Excel to facilitate analysis; and 2) in the prior question you already calculated a test statistic but on a different dataset - calculate the test statistic again using the dataset directly above in selecting the corresponding formal and informal conclusions.

Select one:

a.

We do not reject H0 because z > +2.326, where z = 1.079. We have statistically significant evidence at ? = 1% to show that the true proportion of core body temperature increases amidst higher ambient temperature and humidity levels for the population who do not use electric fans is less than those who do use electric fans.

b.

We accept H0 because z = -2.326, where z = 1.079. We have statistically significant evidence at ? = 5% to show that the true proportion of core body temperature increases amidst higher ambient temperature and humidity levels for the population who do not use electric fans is less than those who do use electric fans.

c.

We do not accept H0 because z

d.

We do not reject H0 because z > -1.645, where z = 1.079. We do not have statistically significant evidence at ? = 5% to show that the true proportion of core body temperature increases amidst higher ambient temperature and humidity levels for the population who do not use electric fans is less than those who do use electric fans.

Please show work

https://www.youtube.com/watch?v=YLHhOJgdOJs

https://www.youtube.com/watch?v=nMzwcXHcBP4

https://www.youtube.com/watch?v=tzfNGv6V0b0

E Hypothesis-Testing-Flowchart-v0.2-2017-02-03.pdf 1 1 - 67% + . . . Which Hypothesis Testing Is my data Procedure do I use? Is my data Dichotomous continuous or One sample Two samples- continuous or Dichotomous dichotomous? dichotomous? Ho: P = Po Ho: H = Ho (1) Hip po Continuous (2) Hi: M > Ho (1) Hitt Hz Continuous (2) Hi: P1 > pz two samples? (3) Hit Hi # Jz (3) Hi: Pi # pz Choose either (1), (2), or (3) Choose either (1), (2), or (3) ndent Fill in po with an actual number Fill in Ho with an actual number Choose either (1), (2), or (3) Are my data Choose either (1), (2), or (3) Define p in words Define u in words Do not fill #1 or #, with numbers Do not fill p, or pz with numbers Define u, groups 1 and 2 in words Define p, groups 1 and 2 in words Matched/ P-Po Z= Decision Rule. Large Samples Decision Rule. Small Samples: Calculate the VPo(1-Po) differences For a = 0.05, For a = 0.05, (1) Reject Ho if z s -1.645 (1) Reject Ho ift s -tan P = (2) Reject Ho if z 2 +1.645 2) Reject Ho if t 2 +tan P1 - P2 n (3) Reject H, if z s -1.96 or z 2 +1.96 (3) Reject Ho if t s -tan or t 2 +taz Vp(1 - P)(1, + 12) where x is the Choose either (1), (2), or (3) Choose either (1), (2), or (3) number of corresponding to Hi decision corresponding to Hi decision successes p, = 31 , P, = 2 p=M+x Find tan and tanz in Table 2 in the n2 m +n2 1-Sample Cont. 2-Sample Cont one-sided test a = .05" and "two-sided where x1 and x2 are the number of In Excel do: test a = .05" columns, respectively. successes in samples 1 and 2, = (phat - p0) / SQRT( p0 * (1-p0) / n) -1-Sample Dich- 2-Sample Dich- Degrees-of-Freedom (df) respectively (a) For one sample, use df = n - 1 b) For two samples, use df = n1 + n2 -2 In Excel do: 2: =(phatl - phat2) / ( SQRT( phat * (1-phat) * (11 + 12) ) ) Choose either (a) or (b) but not both Is my sample size 2 30 or 30 In Excel do: In Excel do: In Excel do: In Excel do: Z. = (Xbar - mu0) / (s / SQRT(n) := (Xbar - mu0) / (s / SQRT(n) = (Xbar1 - Xbar2) / ( Sp * SQRT( 11 + 12 ) : = (Xbarl - Xbar2) / ( Sp * SQRT( 11 + 12 ) (1) Lower-tailed: We reject Ho because z (or t) s -Critical Value. | We do not reject H, because z (or t) > -Critical Value (2) Upper-tailed: We reject H, because z (or t) 2 + Critical Value. | We do not reject H, because z (or t)