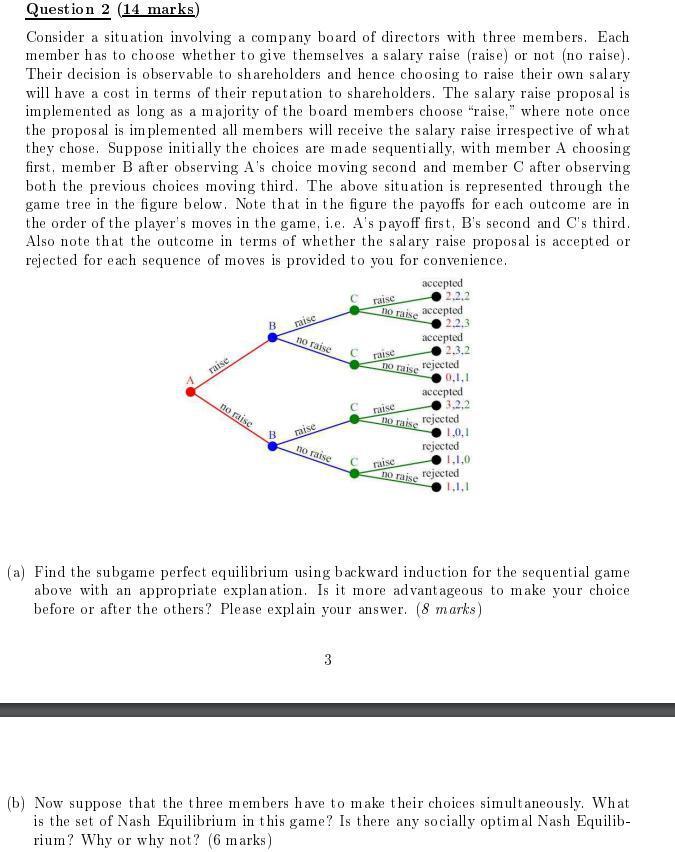
Question 2 (14 marks) Consider a situation involving a company board of directors with three members. Each member has to choose whether to give themselves a salary raise (raise) or not (no raise). Their decision is observable to shareholders and hence choosing to raise their own salary will have a cost in terms of their reputation to shareholders. The salary raise proposal is implemented as long as a majority of the board members choose "raise," where note once the proposal is implemented all members will receive the salary raise irrespective of what they chose. Suppose initially the choices are made sequentially, with member A choosing first, member B after observing A's choice moving second and member Cafter observing both the previous choices moving third. The above situation is represented through the game tree in the figure below. Note that in the figure the payoffs for each outcome are in the order of the player's moves in the game, i.e. A's payoff first, B's second and C's third. Also note that the outcome in terms of whether the salary raise proposal is accepted or rejected for each sequence of moves is provided to you for convenience. accepted raise B mise no raise raise raise no raise Do raise accepted 2.2.3 accepted 2.3.2 ho raise rejected 0,1,1 accepted 3.2.2 raise rejected 1.0.1 rejected 1,1,0 Do raise rejected 1.1.1 raise B raise no raise mise (a) Find the subgame perfect equilibrium using backward induction for the sequential game above with an appropriate explanation. Is it more advantageous to make your choice before or after the others? Please explain your answer. (8 marks) 3 (b) Now suppose that the three members have to make their choices simultaneously. What is the set of Nash Equilibrium in this game? Is there any socially optimal Nash Equilib- rium? Why or why not? (6 marks) Question 2 (14 marks) Consider a situation involving a company board of directors with three members. Each member has to choose whether to give themselves a salary raise (raise) or not (no raise). Their decision is observable to shareholders and hence choosing to raise their own salary will have a cost in terms of their reputation to shareholders. The salary raise proposal is implemented as long as a majority of the board members choose "raise," where note once the proposal is implemented all members will receive the salary raise irrespective of what they chose. Suppose initially the choices are made sequentially, with member A choosing first, member B after observing A's choice moving second and member Cafter observing both the previous choices moving third. The above situation is represented through the game tree in the figure below. Note that in the figure the payoffs for each outcome are in the order of the player's moves in the game, i.e. A's payoff first, B's second and C's third. Also note that the outcome in terms of whether the salary raise proposal is accepted or rejected for each sequence of moves is provided to you for convenience. accepted raise B mise no raise raise raise no raise Do raise accepted 2.2.3 accepted 2.3.2 ho raise rejected 0,1,1 accepted 3.2.2 raise rejected 1.0.1 rejected 1,1,0 Do raise rejected 1.1.1 raise B raise no raise mise (a) Find the subgame perfect equilibrium using backward induction for the sequential game above with an appropriate explanation. Is it more advantageous to make your choice before or after the others? Please explain your answer. (8 marks) 3 (b) Now suppose that the three members have to make their choices simultaneously. What is the set of Nash Equilibrium in this game? Is there any socially optimal Nash Equilib- rium? Why or why not? (6 marks)







