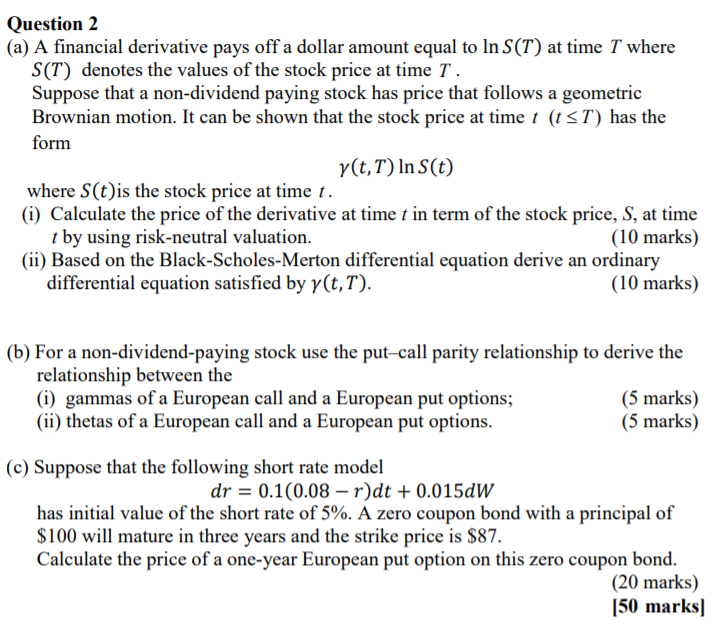
Question 2 (a) A financial derivative pays off a dollar amount equal to In S(T) at time I where S(T) denotes the values of the stock price at time T. Suppose that a non-dividend paying stock has price that follows a geometric Brownian motion. It can be shown that the stock price at time t (1ST) has the form y(t, T) In S(t) where S(t)is the stock price at time t. (i) Calculate the price of the derivative at time t in term of the stock price, S, at time t by using risk-neutral valuation. (10 marks) (ii) Based on the Black-Scholes-Merton differential equation derive an ordinary differential equation satisfied by y(t, T). (10 marks) (b) For a non-dividend-paying stock use the put-call parity relationship to derive the relationship between the (i) gammas of a European call and a European put options; (5 marks) (ii) thetas of a European call and a European put options. (5 marks) (C) Suppose that the following short rate model dr = 0.1(0.08 - r)dt + 0.0150W has initial value of the short rate of 5%. A zero coupon bond with a principal of $100 will mature in three years and the strike price is $87. Calculate the price of a one-year European put option on this zero coupon bond. (20 marks) [50 marks] Question 2 (a) A financial derivative pays off a dollar amount equal to In S(T) at time I where S(T) denotes the values of the stock price at time T. Suppose that a non-dividend paying stock has price that follows a geometric Brownian motion. It can be shown that the stock price at time t (1ST) has the form y(t, T) In S(t) where S(t)is the stock price at time t. (i) Calculate the price of the derivative at time t in term of the stock price, S, at time t by using risk-neutral valuation. (10 marks) (ii) Based on the Black-Scholes-Merton differential equation derive an ordinary differential equation satisfied by y(t, T). (10 marks) (b) For a non-dividend-paying stock use the put-call parity relationship to derive the relationship between the (i) gammas of a European call and a European put options; (5 marks) (ii) thetas of a European call and a European put options. (5 marks) (C) Suppose that the following short rate model dr = 0.1(0.08 - r)dt + 0.0150W has initial value of the short rate of 5%. A zero coupon bond with a principal of $100 will mature in three years and the strike price is $87. Calculate the price of a one-year European put option on this zero coupon bond. (20 marks) [50 marks]







