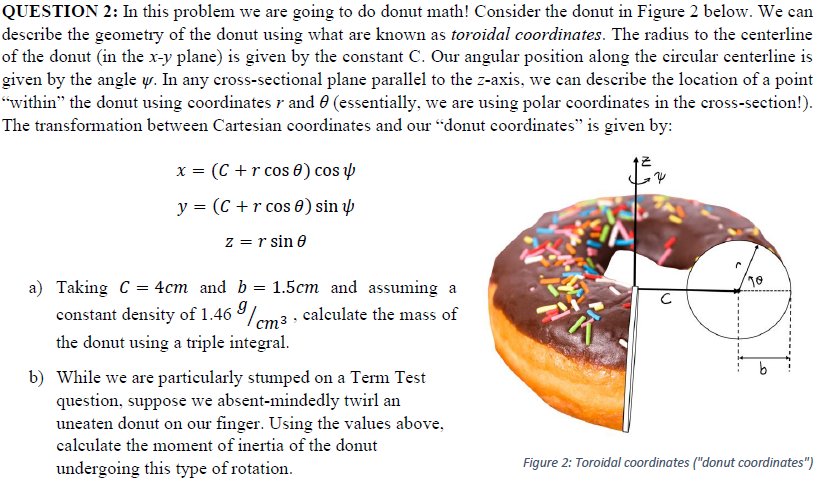
QUESTION 2: In this problem we are going to do donut math! Consider the donut in Figure 2 below. We can describe the geometry of the donut using what are known as toroidal coordinates. The radius to the centerline of the donut (in the x-y plane) is given by the constant C. Our angular position along the circular centerline is given by the angle y. In any cross-sectional plane parallel to the z-axis, we can describe the location of a point within the donut using coordinates r and 8 (essentially, we are using polar coordinates in the cross-section!). The transformation between Cartesian coordinates and our "donut coordinates" is given by: x = (C+r cos ) cos y y = (C + r cos e) sin y z = r sin e 10 a) Taking C = 4cm and b 1.5cm and assuming a constant density of 1.46 9/cm3 , calculate the mass of the donut using a triple integral. b) While we are particularly stumped on a Term Test question, suppose we absent-mindedly twirl an uneaten donut on our finger. Using the values above, calculate the moment of inertia of the donut undergoing this type of rotation. Figure 2: Toroidal coordinates ("donut coordinates") QUESTION 2: In this problem we are going to do donut math! Consider the donut in Figure 2 below. We can describe the geometry of the donut using what are known as toroidal coordinates. The radius to the centerline of the donut (in the x-y plane) is given by the constant C. Our angular position along the circular centerline is given by the angle y. In any cross-sectional plane parallel to the z-axis, we can describe the location of a point within the donut using coordinates r and 8 (essentially, we are using polar coordinates in the cross-section!). The transformation between Cartesian coordinates and our "donut coordinates" is given by: x = (C+r cos ) cos y y = (C + r cos e) sin y z = r sin e 10 a) Taking C = 4cm and b 1.5cm and assuming a constant density of 1.46 9/cm3 , calculate the mass of the donut using a triple integral. b) While we are particularly stumped on a Term Test question, suppose we absent-mindedly twirl an uneaten donut on our finger. Using the values above, calculate the moment of inertia of the donut undergoing this type of rotation. Figure 2: Toroidal coordinates ("donut coordinates")