1.  

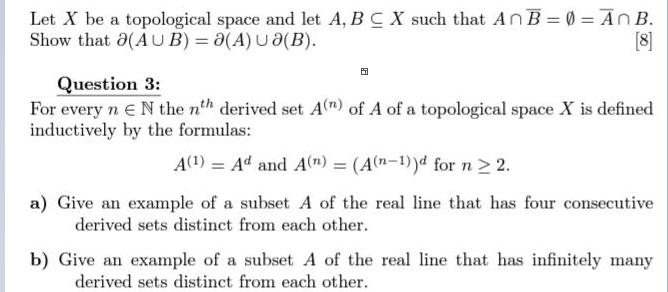
Let X be a topological space and let A, B C X such that AnB=0 = An B. Show that a(AU B) = a(A) Ua(B). [8] Question 3: For every n eN the nth derived set A(m) of A of a topological space X is defined inductively by the formulas: A(1) = Ad and A(m) = (A(n-1))d for n > 2. a) Give an example of a subset A of the real line that has four consecutive derived sets distinct from each other. b) Give an example of a subset A of the real line that has infinitely many derived sets distinct from each other.