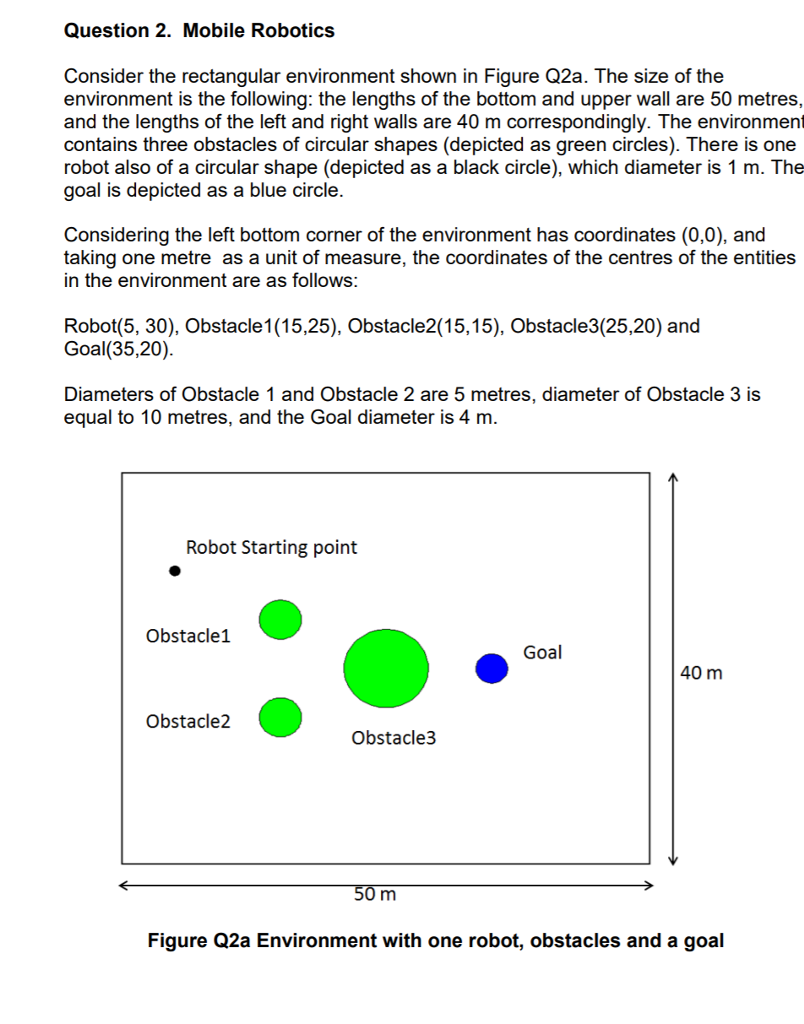
Question 2. Mobile Robotics Consider the rectangular environment shown in Figure Q2a. The size of the environment is the following: the lengths of the bottom and upper wall are 50 metres, and the lengths of the left and right walls are 40 m correspondingly. The environment contains three obstacles of circular shapes (depicted as green circles). There is one robot also of a circular shape (depicted as a black circle), which diameter is 1 m. The goal is depicted as a blue circle. Considering the left bottom corner of the environment has coordinates (0,0), and taking one metre as a unit of measure, the coordinates of the centres of the entities in the environment are as follows: Robot(5, 30), Obstacle1 (15,25), Obstacle2(15,15), Obstacle3(25,20) and Goal(35,20) equal to 10 metres, and the Goal diameter is 4 m. Robot Starting point Obstacle1 Goal 40 m Obstacle2 Obstacle3 Figure Q2a Environment with one robot, obstacles and a goal Design a global artificial potential function for this environment. You have to address the following cases: Design an artificial potential function for each obstacle and the goal, obstacles and the goal at any point in the environment. Please, write your formulas and provide justification for your choice of (a) artificial potential functions. Consider now the case that the robot still has perfect sensing, but star reacting to obstacles only if the distance between the robot and the boun of the obstacle is less than or equal to 4 metres. (b) (c) The same as above but design a new artificial potential function for the goal, so that the robot would move to the goal with constant speed (in the absence of the obstacles) until the distance between the robot and the boundary of the goal is equal to or less than 4 metres. Then the speed of the robot will decrease and becomes equal to zero when the robot reaches the centre of the goal. (d) This case is similar to the case 3, but now the robot stops if it reaches the boundary of the goal. Update artificial potential functions for the obstacles, so that their values will be constant if the distances to the boundaries of the obstacles is equal to or less than 2 metres. (e) Question 2. Mobile Robotics Consider the rectangular environment shown in Figure Q2a. The size of the environment is the following: the lengths of the bottom and upper wall are 50 metres, and the lengths of the left and right walls are 40 m correspondingly. The environment contains three obstacles of circular shapes (depicted as green circles). There is one robot also of a circular shape (depicted as a black circle), which diameter is 1 m. The goal is depicted as a blue circle. Considering the left bottom corner of the environment has coordinates (0,0), and taking one metre as a unit of measure, the coordinates of the centres of the entities in the environment are as follows: Robot(5, 30), Obstacle1 (15,25), Obstacle2(15,15), Obstacle3(25,20) and Goal(35,20) equal to 10 metres, and the Goal diameter is 4 m. Robot Starting point Obstacle1 Goal 40 m Obstacle2 Obstacle3 Figure Q2a Environment with one robot, obstacles and a goal Design a global artificial potential function for this environment. You have to address the following cases: Design an artificial potential function for each obstacle and the goal, obstacles and the goal at any point in the environment. Please, write your formulas and provide justification for your choice of (a) artificial potential functions. Consider now the case that the robot still has perfect sensing, but star reacting to obstacles only if the distance between the robot and the boun of the obstacle is less than or equal to 4 metres. (b) (c) The same as above but design a new artificial potential function for the goal, so that the robot would move to the goal with constant speed (in the absence of the obstacles) until the distance between the robot and the boundary of the goal is equal to or less than 4 metres. Then the speed of the robot will decrease and becomes equal to zero when the robot reaches the centre of the goal. (d) This case is similar to the case 3, but now the robot stops if it reaches the boundary of the goal. Update artificial potential functions for the obstacles, so that their values will be constant if the distances to the boundaries of the obstacles is equal to or less than 2 metres. (e)