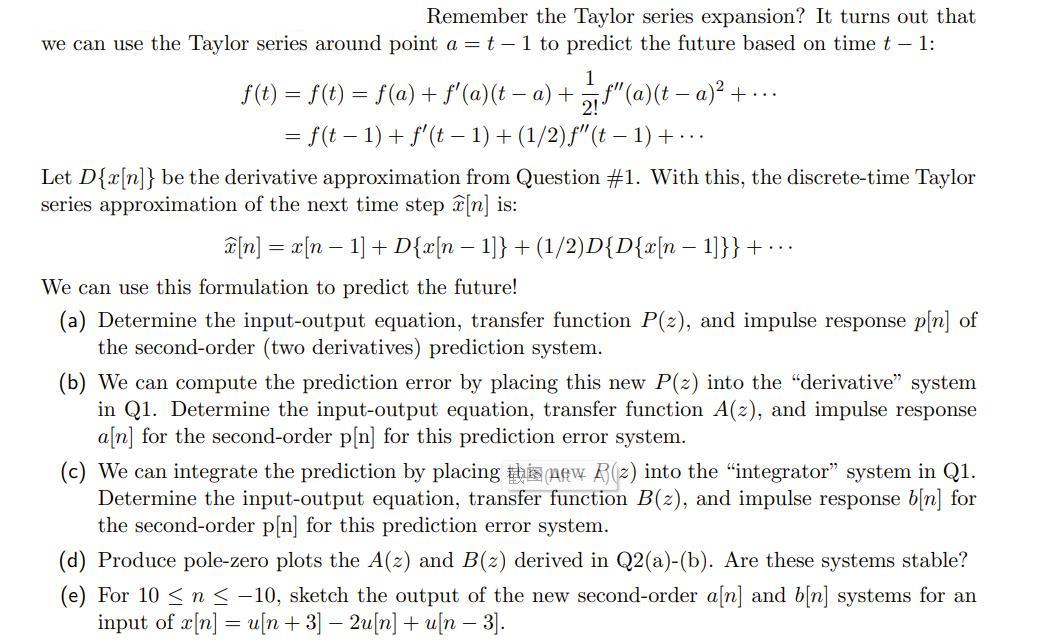
Remember the Taylor series expansion? It turns out that we can use the Taylor series around point a = t- 1 to predict the future based on time t - 1: 1 (t) = f(t) = f(a) + '(a)(t a) + " (a)(t a) + ... - 2! = f(t-1) + f'(t-1) + (1/2) "(t 1) + Let D{x[n]} be the derivative approximation from Question #1. With this, the discrete-time Taylor series approximation of the next time step [n] is: x[n] = x[n 1] + D{x[n 1]} + (1/2)D{D{x[n 1]}} + ... - We can use this formulation to predict the future! (a) Determine the input-output equation, transfer function P(z), and impulse response p[n] of the second-order (two derivatives) prediction system. (b) We can compute the prediction error by placing this new P(z) into the "derivative" system in Q1. Determine the input-output equation, transfer function A(z), and impulse response a[n] for the second-order p[n] for this prediction error system. (c) We can integrate the prediction by placing this new (2) into the "integrator" system in Q1. Determine the input-output equation, transfer function B(z), and impulse response b[n] for the second-order p[n] for this prediction error system. (d) Produce pole-zero plots the A(z) and B(z) derived in Q2(a)-(b). Are these systems stable? (e) For 10 n < -10, sketch the output of the new second-order a[n] and b[n] systems for an input of x[n] =u[n+3] - 2u[n] + u[n - 3]. Remember the Taylor series expansion? It turns out that we can use the Taylor series around point a = t - 1 to predict the future based on time t - 1: 1 (t) = f(t) = f(a) + '(a)(t a) + (a)(t a) + ... - 2! = f(t-1) + f'(t-1) + (1/2) "(t 1) + Let D{x[n]} be the derivative approximation from Question #1. With this, the discrete-time Taylor series approximation of the next time step [n] is: x[n] = x[n 1] + D{x[n 1]} + (1/2)D{D{x[n 1]}} + ... - We can use this formulation to predict the future! (a) Determine the input-output equation, transfer function P(z), and impulse response p[n] of the second-order (two derivatives) prediction system. (b) We can compute the prediction error by placing this new P(z) into the "derivative" system in Q1. Determine the input-output equation, transfer function A(z), and impulse response a[n] for the second-order p[n] for this prediction error system. (c) We can integrate the prediction by placing this new (2) into the "integrator" system in Q1. Determine the input-output equation, transfer function B(z), and impulse response b[n] for the second-order p[n] for this prediction error system. (d) Produce pole-zero plots the A(z) and B(z) derived in Q2(a)-(b). Are these systems stable? (e) For 10 n -10, sketch the output of the new second-order a[n] and b[n] systems for an input of x[n] =u[n+3] - 2u[n] + u[n - 3].