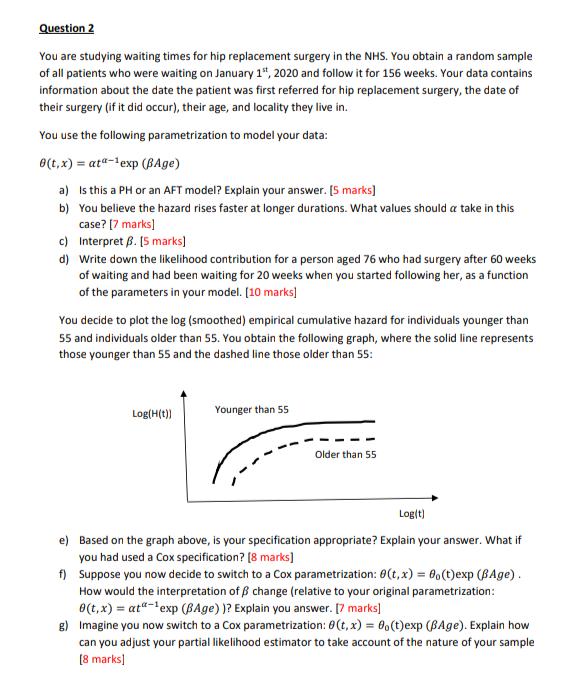
Question 2 You are studying waiting times for hip replacement surgery in the NHS. You obtain a random sample of all patients who were waiting on January 1st, 2020 and follow it for 156 weeks. Your data contains information about the date the patient was first referred for hip replacement surgery, the date of their surgery (if it did occur), their age, and locality they live in. You use the following parametrization to model your data: e(t, x) = at-exp (BAge) a) Is this a PH or an AFT model? Explain your answer. [5 marks] b) You believe the hazard rises faster at longer durations. What values should a take in this case? [7 marks] c) Interpret . [5 marks] d) Write down the likelihood contribution for a person aged 76 who had surgery after 60 weeks of waiting and had been waiting for 20 weeks when you started following her, as a function of the parameters in your model. [10 marks] You decide to plot the log (smoothed) empirical cumulative hazard for individuals younger than 55 and individuals older than 55. You obtain the following graph, where the solid line represents those younger than 55 and the dashed line those older than 55: Log(H(t)) Younger than 55 Older than 55 Log(t) e) Based on the graph above, is your specification appropriate? Explain your answer. What if you had used a Cox specification? [8 marks] f) Suppose you now decide to switch to a Cox parametrization: 0(t, x) = 0o (t)exp (BAge). How would the interpretation of change (relative to your original parametrization: 0(t, x) = ata-exp (BAge))? Explain you answer. [7 marks] g) Imagine you now switch to a Cox parametrization: 0(t, x) = 0, (t)exp (BAge). Explain how can you adjust your partial likelihood estimator to take account of the nature of your sample [8 marks]