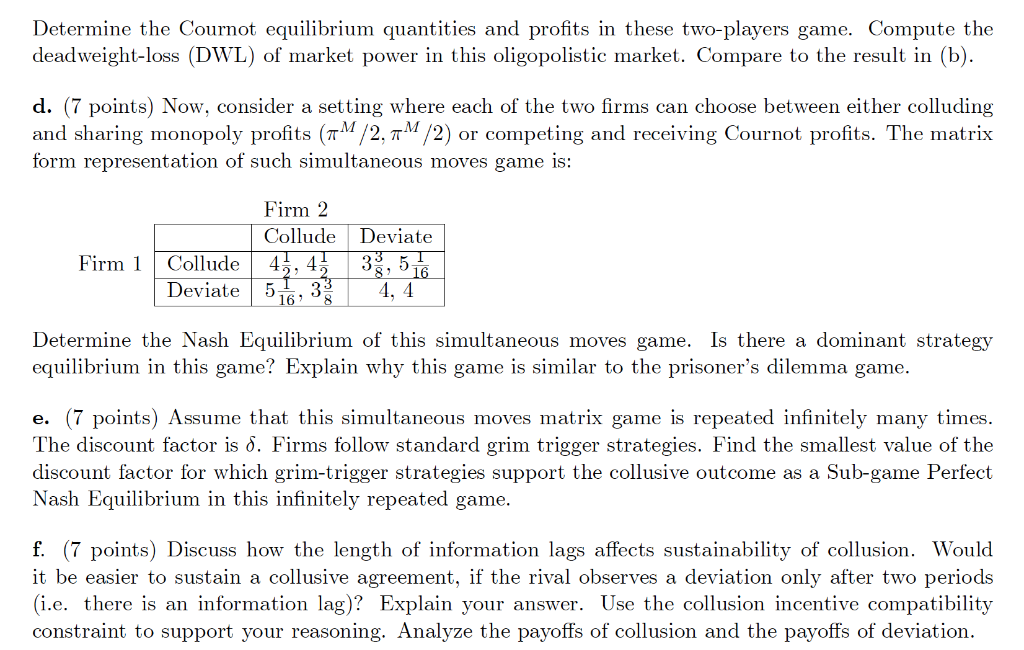
Question 3 (44 points): Market Interventions, Oligopoly and Collusion a. (8 points) Suppose the market for internet access is competitive. Market demand is given by P=9Q(P is in Dollars and Q is in units of millions of megabytes, MB ) and market supply is P=0.5Q. Derive the deadweight-loss (DWL) from a per-unit tax of $3 imposed on sellers. Illustrate your results graphically. b. (7 points) Next, assume that the market for internet access is monopolized. The monopolist's marginal cost of supplying one MB is constant and equal to $3. Market demand is given by P=9Q. Compute the deadweight loss from monopolization of this market. Illustrate the DWL graphically in the (Q,p)-diagram. Determine the Lerner index evaluated at the monopoly price. Discuss the relation between the Lerner Index and market power. c. (8 points) Next, assume that the market for internet access is dominated by 2 symmetric firms that serve a demand given by p=9Q, where Q=q1+q2 denotes the aggregate quantity put in the market by the 2 competitors. Each firm has a marginal cost of production equal to $3 per unit. Determine the Cournot equilibrium quantities and profits in these two-players game. Compute the deadweight-loss (DWL) of market power in this oligopolistic market. Compare to the result in (b). d. (7 points) Now, consider a setting where each of the two firms can choose between either colluding and sharing monopoly profits (M/2,M/2) or competing and receiving Cournot profits. The matrix form representation of such simultaneous moves game is: Determine the Nash Equilibrium of this simultaneous moves game. Is there a dominant strategy equilibrium in this game? Explain why this game is similar to the prisoner's dilemma game. e. (7 points) Assume that this simultaneous moves matrix game is repeated infinitely many times. The discount factor is . Firms follow standard grim trigger strategies. Find the smallest value of the discount factor for which grim-trigger strategies support the collusive outcome as a Sub-game Perfect Nash Equilibrium in this infinitely repeated game. f. (7 points) Discuss how the length of information lags affects sustainability of collusion. Would it be easier to sustain a collusive agreement, if the rival observes a deviation only after two periods (i.e. there is an information lag)? Explain your answer. Use the collusion incentive compatibility constraint to support your reasoning. Analyze the payoffs of collusion and the payoffs of deviation. Question 3 (44 points): Market Interventions, Oligopoly and Collusion a. (8 points) Suppose the market for internet access is competitive. Market demand is given by P=9Q(P is in Dollars and Q is in units of millions of megabytes, MB ) and market supply is P=0.5Q. Derive the deadweight-loss (DWL) from a per-unit tax of $3 imposed on sellers. Illustrate your results graphically. b. (7 points) Next, assume that the market for internet access is monopolized. The monopolist's marginal cost of supplying one MB is constant and equal to $3. Market demand is given by P=9Q. Compute the deadweight loss from monopolization of this market. Illustrate the DWL graphically in the (Q,p)-diagram. Determine the Lerner index evaluated at the monopoly price. Discuss the relation between the Lerner Index and market power. c. (8 points) Next, assume that the market for internet access is dominated by 2 symmetric firms that serve a demand given by p=9Q, where Q=q1+q2 denotes the aggregate quantity put in the market by the 2 competitors. Each firm has a marginal cost of production equal to $3 per unit. Determine the Cournot equilibrium quantities and profits in these two-players game. Compute the deadweight-loss (DWL) of market power in this oligopolistic market. Compare to the result in (b). d. (7 points) Now, consider a setting where each of the two firms can choose between either colluding and sharing monopoly profits (M/2,M/2) or competing and receiving Cournot profits. The matrix form representation of such simultaneous moves game is: Determine the Nash Equilibrium of this simultaneous moves game. Is there a dominant strategy equilibrium in this game? Explain why this game is similar to the prisoner's dilemma game. e. (7 points) Assume that this simultaneous moves matrix game is repeated infinitely many times. The discount factor is . Firms follow standard grim trigger strategies. Find the smallest value of the discount factor for which grim-trigger strategies support the collusive outcome as a Sub-game Perfect Nash Equilibrium in this infinitely repeated game. f. (7 points) Discuss how the length of information lags affects sustainability of collusion. Would it be easier to sustain a collusive agreement, if the rival observes a deviation only after two periods (i.e. there is an information lag)? Explain your answer. Use the collusion incentive compatibility constraint to support your reasoning. Analyze the payoffs of collusion and the payoffs of deviation








