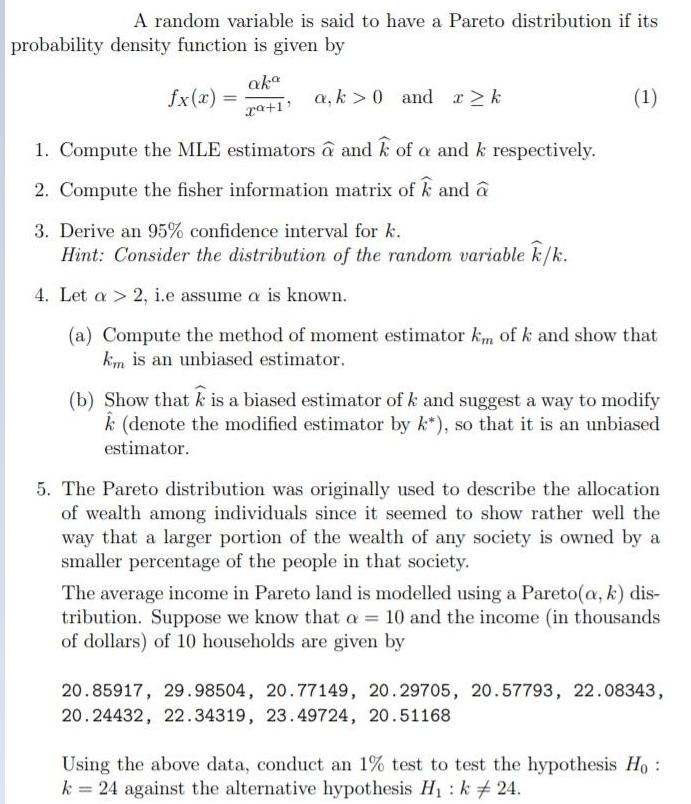
A random variable is said to have a Pareto distribution if its probability density function is given by aka fx(x) = a, k > 0 and r k (1) %3D pa+1 1. Compute the MLE estimators a and k of a and k respectively. 2. Compute the fisher information matrix of k and a 3. Derive an 95% confidence interval for k. Hint: Consider the distribution of the random variable k/k. 4. Let a > 2, i.e assume a is known. (a) Compute the method of moment estimator km of k and show that kim is an unbiased estimator. (b) Show that k is a biased estimator of k and suggest a way to modify k (denote the modified estimator by k*), so that it is an unbiased estimator. 5. The Pareto distribution was originally used to describe the allocation of wealth among individuals since it seemed to show rather well the way that a larger portion of the wealth of any society is owned by a smaller percentage of the people in that society. The average income in Pareto land is modelled using a Pareto(a, k) dis- tribution. Suppose we know that a = 10 and the income (in thousands of dollars) of 10 households are given by 20.85917, 29.98504, 20.77149, 20.29705, 20.57793, 22.08343, 20.24432, 22.34319, 23.49724, 20.51168 Using the above data, conduct an 1% test to test the hypothesis Ho: k = 24 against the alternative hypothesis H1: k # 24.