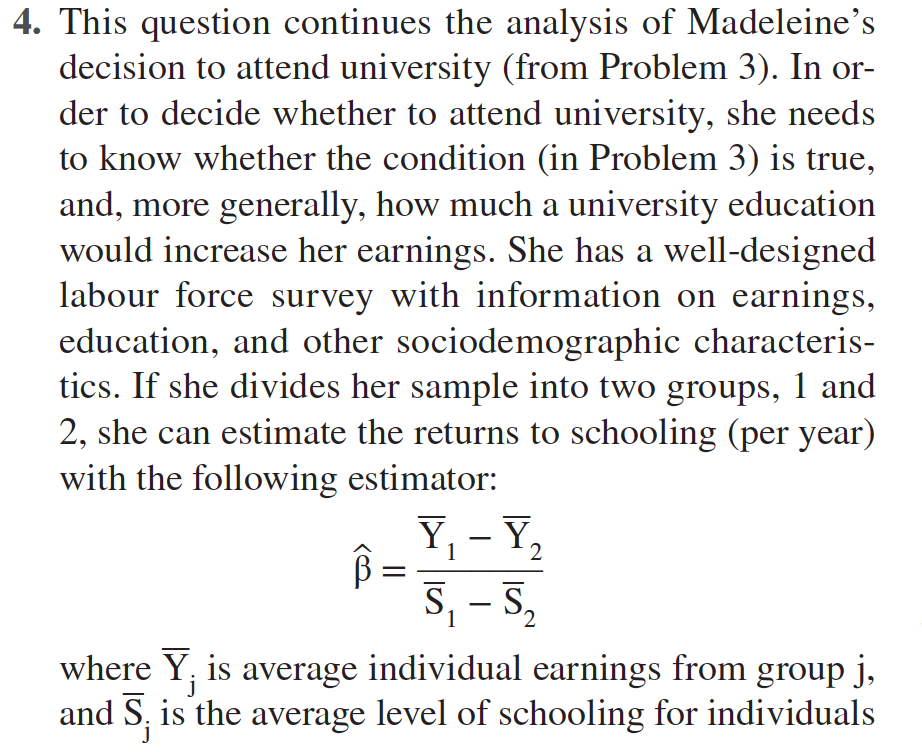
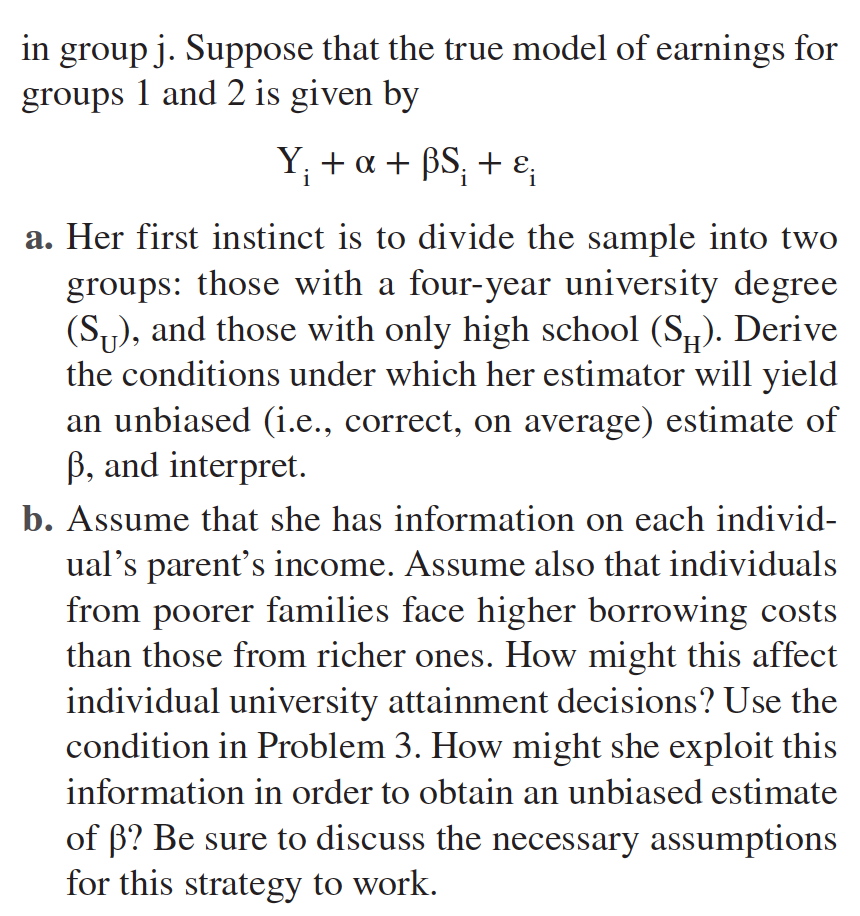
Question 3 for reference:

Please answer all parts. Thanks

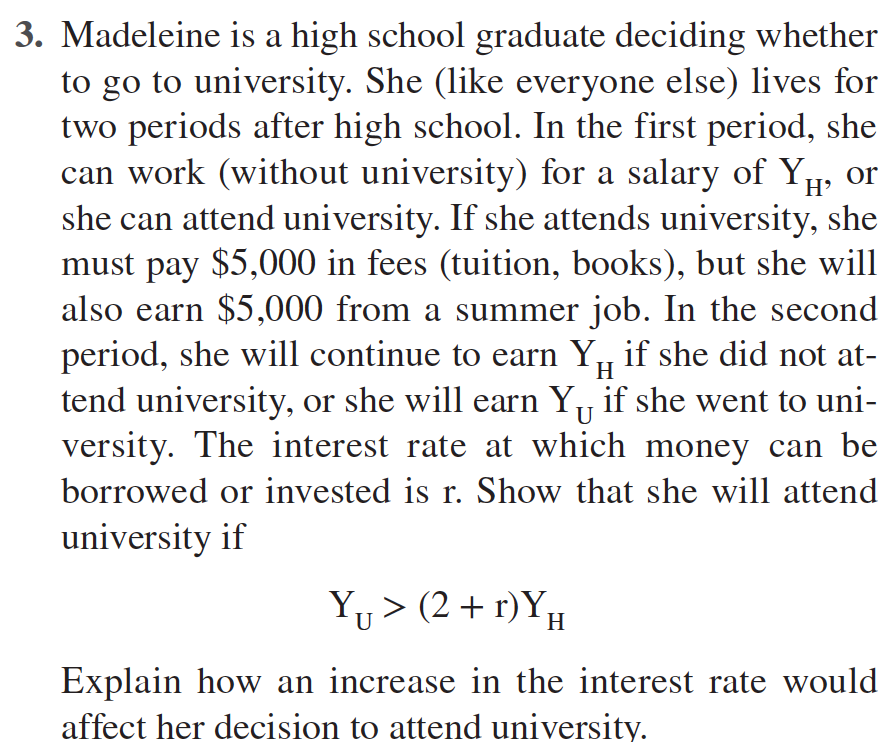
This question continues the analysis of Madeleine's decision to attend university (from Problem 3). In order to decide whether to attend university, she needs to know whether the condition (in Problem 3) is true, and, more generally, how much a university education would increase her earnings. She has a well-designed labour force survey with information on earnings, education, and other sociodemographic characteristics. If she divides her sample into two groups, 1 and 2 , she can estimate the returns to schooling (per year) with the following estimator: =S1S2Y1Y2 where Yj is average individual earnings from group j, and Sj is the average level of schooling for individuals in group j. Suppose that the true model of earnings for groups 1 and 2 is given by Yi++Si+i a. Her first instinct is to divide the sample into two groups: those with a four-year university degree (SU), and those with only high school (SH). Derive the conditions under which her estimator will yield an unbiased (i.e., correct, on average) estimate of , and interpret. b. Assume that she has information on each individual's parent's income. Assume also that individuals from poorer families face higher borrowing costs than those from richer ones. How might this affect individual university attainment decisions? Use the condition in Problem 3. How might she exploit this information in order to obtain an unbiased estimate of ? Be sure to discuss the necessary assumptions for this strategy to work. 3. Madeleine is a high school graduate deciding whether to go to university. She (like everyone else) lives for two periods after high school. In the first period, she can work (without university) for a salary of YH, or she can attend university. If she attends university, she must pay $5,000 in fees (tuition, books), but she will also earn $5,000 from a summer job. In the second period, she will continue to earn YH if she did not attend university, or she will earn YU if she went to university. The interest rate at which money can be borrowed or invested is r. Show that she will attend university if YU>(2+r)YH Explain how an increase in the interest rate would affect her decision to attend university. This question continues the analysis of Madeleine's decision to attend university (from Problem 3). In order to decide whether to attend university, she needs to know whether the condition (in Problem 3) is true, and, more generally, how much a university education would increase her earnings. She has a well-designed labour force survey with information on earnings, education, and other sociodemographic characteristics. If she divides her sample into two groups, 1 and 2 , she can estimate the returns to schooling (per year) with the following estimator: =S1S2Y1Y2 where Yj is average individual earnings from group j, and Sj is the average level of schooling for individuals in group j. Suppose that the true model of earnings for groups 1 and 2 is given by Yi++Si+i a. Her first instinct is to divide the sample into two groups: those with a four-year university degree (SU), and those with only high school (SH). Derive the conditions under which her estimator will yield an unbiased (i.e., correct, on average) estimate of , and interpret. b. Assume that she has information on each individual's parent's income. Assume also that individuals from poorer families face higher borrowing costs than those from richer ones. How might this affect individual university attainment decisions? Use the condition in Problem 3. How might she exploit this information in order to obtain an unbiased estimate of ? Be sure to discuss the necessary assumptions for this strategy to work. 3. Madeleine is a high school graduate deciding whether to go to university. She (like everyone else) lives for two periods after high school. In the first period, she can work (without university) for a salary of YH, or she can attend university. If she attends university, she must pay $5,000 in fees (tuition, books), but she will also earn $5,000 from a summer job. In the second period, she will continue to earn YH if she did not attend university, or she will earn YU if she went to university. The interest rate at which money can be borrowed or invested is r. Show that she will attend university if YU>(2+r)YH Explain how an increase in the interest rate would affect her decision to attend university