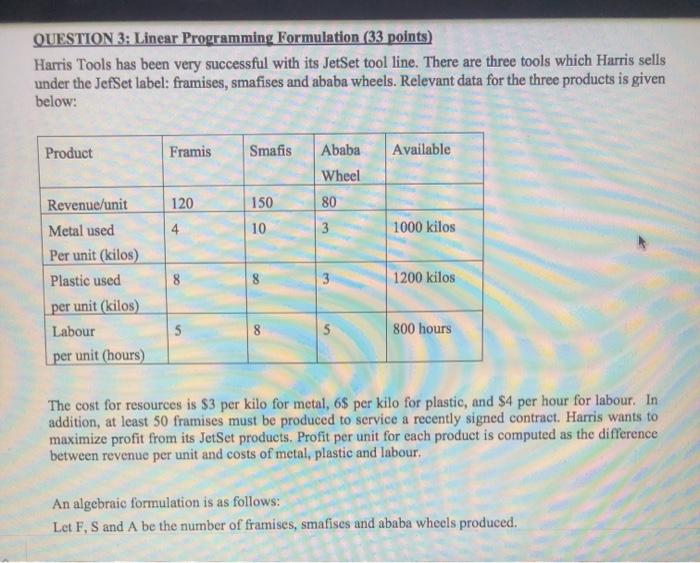
QUESTION 3: Linear Programming Formulation (33 points) Harris Tools has been very successful with its Jet Set tool line. There are three tools which Harris sells under the JefSet label: framises, smafises and ababa wheels. Relevant data for the three products is given below: Product Framis Smafis Available Ababa Wheel 120 150 80 4 10 3 1000 kilos Revenue/unit Metal used Per unit (kilos) Plastic used per unit (kilos) Labour per unit (hours) 8 8 1200 kilos 5 8 800 hours The cost for resources is $3 per kilo for metal, 6$ per kilo for plastic, and $4 per hour for labour. In addition, at least 50 framises must be produced to service a recently signed contract. Harris wants to maximize profit from its Jet Set products, Profit per unit for each product is computed as the difference between revenue per unit and costs of metal, plastic and labour. An algebraic formulation is as follows: Let F, S and A be the number of framises, smafises and ababa wheels produced. between revenue per unit and costs or metal, plastic and labour. An algebraic formulation is as follows: Let F, S and A be the number of framises, smafises and ababa wheels produced. Maximize 40F + 40S +33A subject to 4F + 108 + 3A S 1000 8F + 8S+ 3A S 1200 5F + 8S+SA 800 F 50 F, SA 20 Each formulation question below is independent of the others, this means that when you are answering a part, ignore the previous parts. In (b) to (1) the formulation is altered. You may either write out a complete formulation for the altered problem, or better yet, just clearly state exactly what changes need to be made in the above formulation. (@) Show exactly how the profit per unit of 833 for ababu wheels is computed. (2 points) (b) The revenue per unit for tramiscs, has decreased to $115. How does the formulation change? (2 points) (@) The cost per hour for labour has decreased to $3 per hour. How does the formulation change? (2 points) I (d) At least 30% of the total number of units produced must be Smafis. How does the formulation change? (3 points) (e) The profit derived from abahu wheels must constitute at least 50% of the total profit. How does the formulation change? (3 points) (f) An additional 300 kilos of plastic are available to purchase at a cost of 58 per unit instead of the original cost of $6 per unit. How does the formulation change? (6 points) (g) Formulate the original linear programming problem on a spreadsheet and SOLVE using Excel Solver (Provide the corresponding Excel Spreadsheet" and the "Sensitivity Report"). Include "managerial statements that communicate the results of the analysis. (1.e. describe verbally the results). (10 points) th) What would be the impact on profits, if Harris tools force the production of exactly 10 saalisa (ie. S-1017 Justify your answer from the sensitivity report (3 points). ED Harris tools woald like to increase its profit as such it is considering increasing the capacity of ONE of its resources. What is your best advice? Justify your answer from the sensitivity report 2 points) QUESTION 3: Linear Programming Formulation (33 points) Harris Tools has been very successful with its Jet Set tool line. There are three tools which Harris sells under the JefSet label: framises, smafises and ababa wheels. Relevant data for the three products is given below: Product Framis Smafis Available Ababa Wheel 120 150 80 4 10 3 1000 kilos Revenue/unit Metal used Per unit (kilos) Plastic used per unit (kilos) Labour per unit (hours) 8 8 1200 kilos 5 8 800 hours The cost for resources is $3 per kilo for metal, 6$ per kilo for plastic, and $4 per hour for labour. In addition, at least 50 framises must be produced to service a recently signed contract. Harris wants to maximize profit from its Jet Set products, Profit per unit for each product is computed as the difference between revenue per unit and costs of metal, plastic and labour. An algebraic formulation is as follows: Let F, S and A be the number of framises, smafises and ababa wheels produced. between revenue per unit and costs or metal, plastic and labour. An algebraic formulation is as follows: Let F, S and A be the number of framises, smafises and ababa wheels produced. Maximize 40F + 40S +33A subject to 4F + 108 + 3A S 1000 8F + 8S+ 3A S 1200 5F + 8S+SA 800 F 50 F, SA 20 Each formulation question below is independent of the others, this means that when you are answering a part, ignore the previous parts. In (b) to (1) the formulation is altered. You may either write out a complete formulation for the altered problem, or better yet, just clearly state exactly what changes need to be made in the above formulation. (@) Show exactly how the profit per unit of 833 for ababu wheels is computed. (2 points) (b) The revenue per unit for tramiscs, has decreased to $115. How does the formulation change? (2 points) (@) The cost per hour for labour has decreased to $3 per hour. How does the formulation change? (2 points) I (d) At least 30% of the total number of units produced must be Smafis. How does the formulation change? (3 points) (e) The profit derived from abahu wheels must constitute at least 50% of the total profit. How does the formulation change? (3 points) (f) An additional 300 kilos of plastic are available to purchase at a cost of 58 per unit instead of the original cost of $6 per unit. How does the formulation change? (6 points) (g) Formulate the original linear programming problem on a spreadsheet and SOLVE using Excel Solver (Provide the corresponding Excel Spreadsheet" and the "Sensitivity Report"). Include "managerial statements that communicate the results of the analysis. (1.e. describe verbally the results). (10 points) th) What would be the impact on profits, if Harris tools force the production of exactly 10 saalisa (ie. S-1017 Justify your answer from the sensitivity report (3 points). ED Harris tools woald like to increase its profit as such it is considering increasing the capacity of ONE of its resources. What is your best advice? Justify your answer from the sensitivity report 2 points)