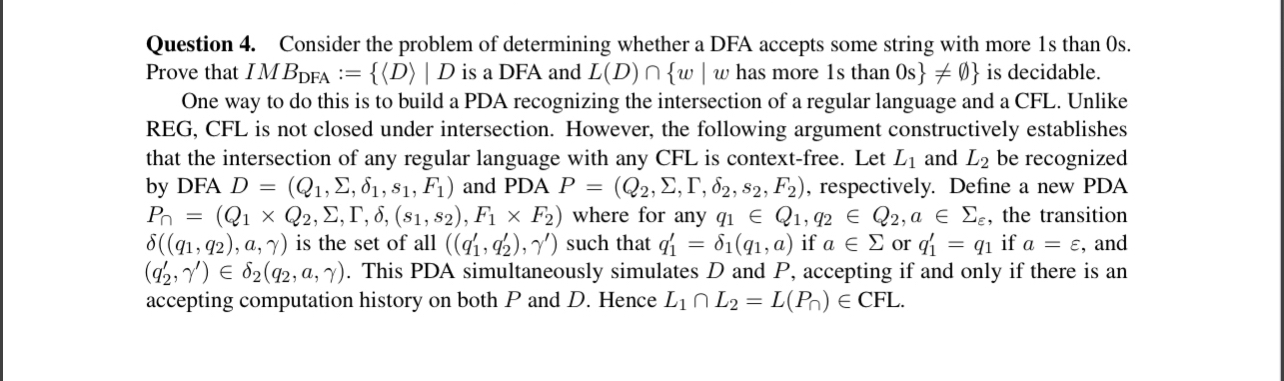
Question $4.$ Consider the problem of determining whether a DFA accepts some string with more $1s$ than $0$ s$.$ Prove that is a DFA and has more $1$ s than $0s$ is decidable.

One way to do this is to build a PDA recognizing the intersection of a regular language and a CFL$.$ Unlike REG, CFL is not closed under intersection. However, the following argument constructively establishes that the intersection of any regular language with any CFL is context$-$free. Let $L_1$ and $L_2$ be recognized by DFA $D=(Q_1,,{}_1,s_1,F_1)$ and PDA $P=(Q_2,,,{}_2,s_2,F_2),$ respectively. Define a new PDA $P_{}=(Q_1{Q}_2,,,,(s_1,s_2),F_1{F}_2)$ where for any $q_{1}in{Q}_1,q_{2}in{Q}_2,$ain${}_{},$ the transition $((q_1,q_2),a,)$ is the set of all $((q_1^{\text{'}},q_2^{\text{'}}),{}^{\text{'}})$ such that $q_1^{\text{'}}={}_1(q_1,a)$ if ain$$ or $q_1^{\text{'}}=q_1$ if $a=,$ and $(q_2^{\text{'}},{}^{\text{'}})in{}_2(q_2,a,).$ This PDA simultaneously simulates $D$ and $P,$ accepting if and only if there is an accepting computation history on both $P$ and $D.$ Hence $L_1{L}_2=L(P_{})inCFL.$