

Question 41..please its complete i need help
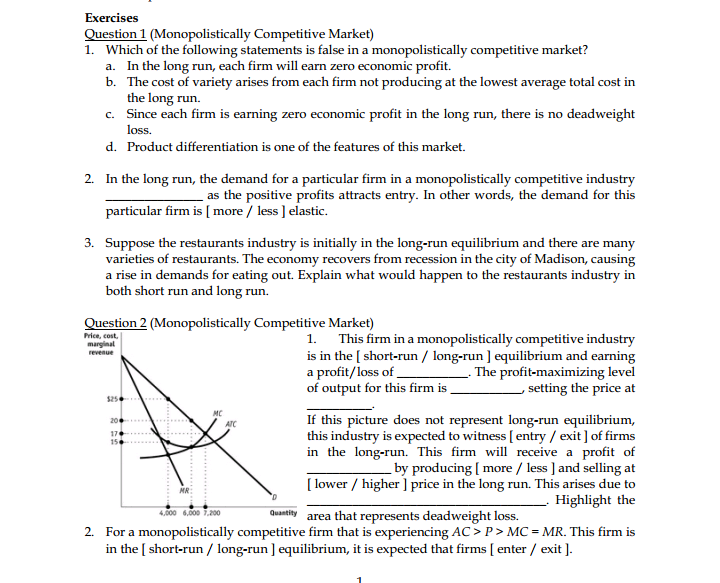
Now discuss the following. a) The inflation rate calculated from GDP deflator using traditional and chain-weighting method are -45.45% and -42.86%, respectively. What can be concluded from the difference in inflation rate. The growth rate of real GDP calculated using traditional and chain-weighting method are 46.67% and 40%, respectively. What can be concluded from the differences? b) Which categories of good would lead to chain-weighting method being preferred? (Hint: Look at the deflation rate.) Question 2 (Optimal Consumption-Labor Choice - Additional Problem in Homework) Consider a Robinson Crusoe simple economy without storage technology. The production function with only labor (() as factor of production is y = f (() = (/2. The utility function from consuming c and exerting labor { is u (e. () = log (c) - f. The market-clearing condition requires that y = c. Crusoe seeks to maximize his utility subject to his production. a) Find the marginal product of labor. b) Find the equation of an indifference curve given a level of utility . ") Find the slope of an indifference curve given a level of utility . Does it hold that, for the same level of labor supply , the higher indifference curve has higher slope? There are two ways to solve for the optimal consumption-labor choice. Method 1: Using the tangency condition (MRS = MPL), note that MRS = - (Ou/80) / (Ou/8c) d) Graphically exhibit the optimal consumption-labor choice. e) Find the marginal rate of substitution between consumption and labor supply. f) Find the optimal consumption, c", and labor supply (*. Show the answers in graph drawn in d). (Hint: Remember that Crusoe eats, c, all he produces, y = (1/2. ) Method 2: Substituting the market-clearing condition - since c = y = (1/2, one can substitute c into utility function to receive a ((1/2, () = log ((1/2) -4. The problem is now a single-variable unconstrained maximiza- tion problem with / as choice variable. g) Find the optimal consumption, c", and labor supply, ". Question 3 (Substitution and Wealth Effect from a Shift in Production Function - Problem 2.8 in Homework) Assume the production function is y = Ave + B. What are the effects on household's work effort, f, output and consumption, c, from: a) An increase in the coefficient A b) An increase in the coefficient B (This is a good question to review 2c) above.) Question 4 (Productivity - Problem 2.11 in Homework) A popular measure of productivity is the ratio of output (e.g., real GDP ) to employment (e.g., worker-hours) that is, y/4. Consider the usual concave production function that takes the form of y = A(", 0 P > MC = MR. This firm is in the [ short-run / long-run ] equilibrium, it is expected that firms [ enter / exit ]












