Answered step by step
Verified Expert Solution
Question
1 Approved Answer
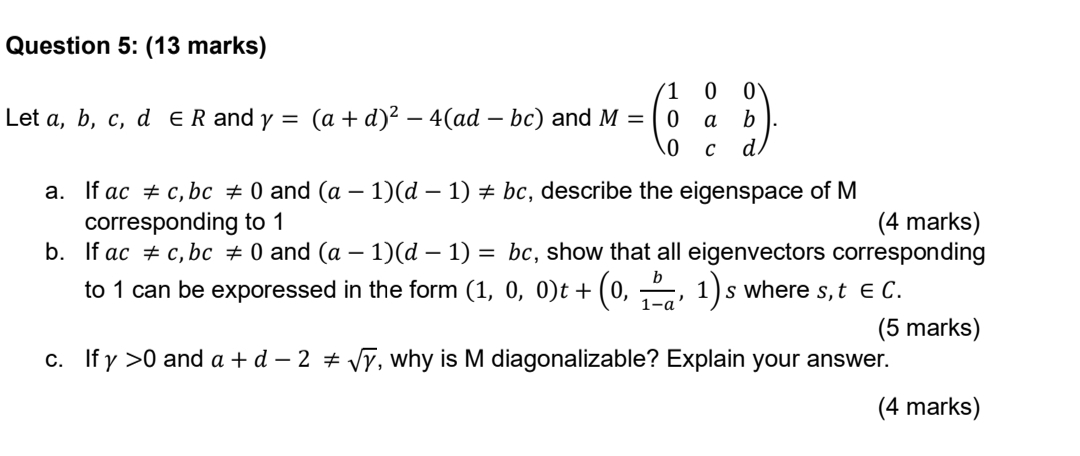
Question 5: (13 marks) Let a,b,c,dinR and gamma =(a+d)^(2)-4(ad-bc) and M=([1,0,0],[0,a,b],[0,c,d]) . a. If ac!=c,bc!=0 and (a-1)(d-1)!=bc , describe the eigenspace of M corresponding
Question 5: (13 marks)\ Let
a,b,c,dinRand
\\\\gamma =(a+d)^(2)-4(ad-bc)and
M=([1,0,0],[0,a,b],[0,c,d]).\ a. If
ac!=c,bc!=0and
(a-1)(d-1)!=bc, describe the eigenspace of
M\ corresponding to 1\ b. If
ac!=c,bc!=0and
(a-1)(d-1)=bc, show that all eigenvectors corresponding\ to 1 can be exporessed in the form
(1,0,0)t+(0,(b)/(1-a),1)swhere
s,tinC.\ (5 marks)\ c. If
\\\\gamma >0and
a+d-2!=\\\\sqrt(\\\\gamma ), why is M diagonalizable? Explain your answer.
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


