Question
Question: Discuss these tough questions and give honest results. 1. Determine if given the that 38 is the ciphertext, the plain text code for {1
Question:
Discuss these tough questions and give honest results.
1. Determine if given the that 38 is the ciphertext, the plain text code for {1 6 8 15 24} which is the Knapsack.
2. Between a jumbled knapsack and a knapsack problem that is super increasing, which problem is easy to solve?
3. Given that you may apply the RSA algorithm where PT message equals 88, determine the value of CT given that p equals 11, q equals 17 and that you are supposed to choose e=7.
4. Calculate for the private key (d, p, q) which is given by the public key denoted by (e=23, n=233 241=56,153).
5. Determine the private key of a user of an RSA system where the public key is given as e = 31, n = 3599.
6. Determine the ciphertext for the message 'WHY' given that the plaintext message consists of mainly single letters that are of 5-bit numerical values in the range (00000)2 to (11001)2. Also, the super-increasing 5- tuple (2, 3, 7, 15, 31), m = 61 and a = 17 is the secret deciphering key.
7. Can we say that the public key in Merkle-Hellman Cryptosystem is used to decrypt messages but cannot be used to encrypt messages. Is it true that the private key on the other hand is used to encrypt messages?
8. Determine another name that is used to refer to Merkle-Hellman Cryptosystem.
9. Determine the value of n given a knapsack that has been formed from the weights of the super-increasing series {1, 2, 4, 9, 20, and 38} whose weight is 23 units.
10. Given a set {1, 2, 3, 9, 10, and 24}, is it true that it is super-increasing?
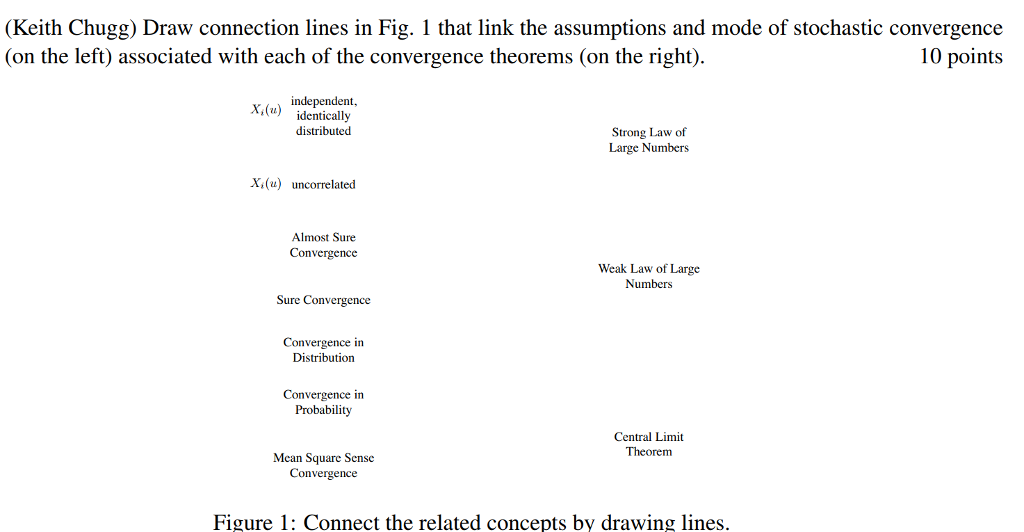
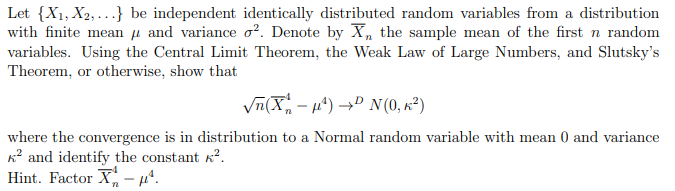
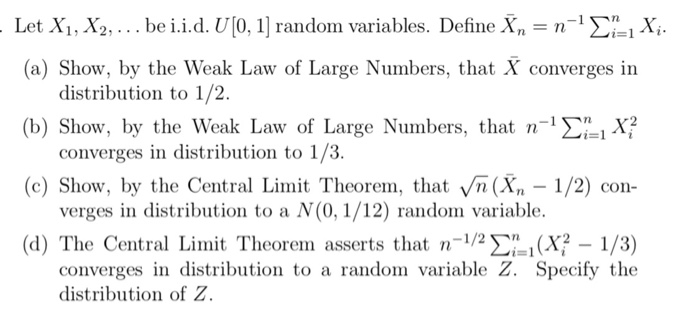
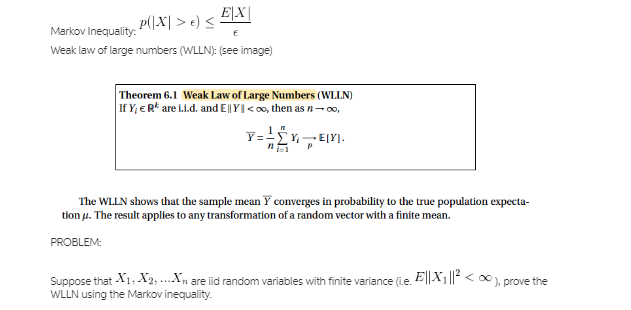
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


