Question:

In the article by Gadd and Phipps (2012), they refer to the challenges faced by psychological and, specifically, neuropsychological assessment. Their study focused on a preliminary standardisation of the Wisconsin Card Sorting Test (a non-verbal measure) for Setswana-speaking university students. The US normative sample is described as participants (N = 899) from both genders who were screened beforehand to exclude individuals with a history of neurological, learning, emotional and attention difficulties. The South African sample consisted of university students (N = 93) from both genders, between the ages of 18 and 29, who were screened in terms of hearing and visual impairments and any history of psychiatriceurological difficulties. The latter was done to prevent contamination of the results by these variables. The students were from the University of Limpopo, Medunsa Campus. Answer Questions 1 to 5.

Question 5

Gadd and Phipps (2012) found that the results of the South African sample on the Wisconsin Card Sorting Test did not resemble a normal distribution and could not be converted to a normal distribution. This implies that the ...

(1) mean performance of this sample cannot be calculated

(2) standard deviation will differ below and above the mean

(3) mean and standard deviation do not provide a predetermined distribution of performance

(4) results cannot be replicated

Would 1 be the correct answer?

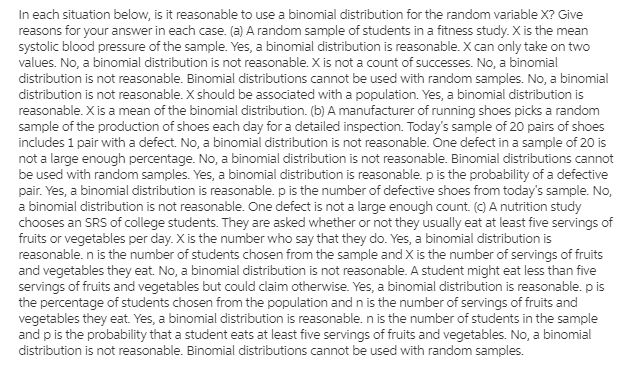
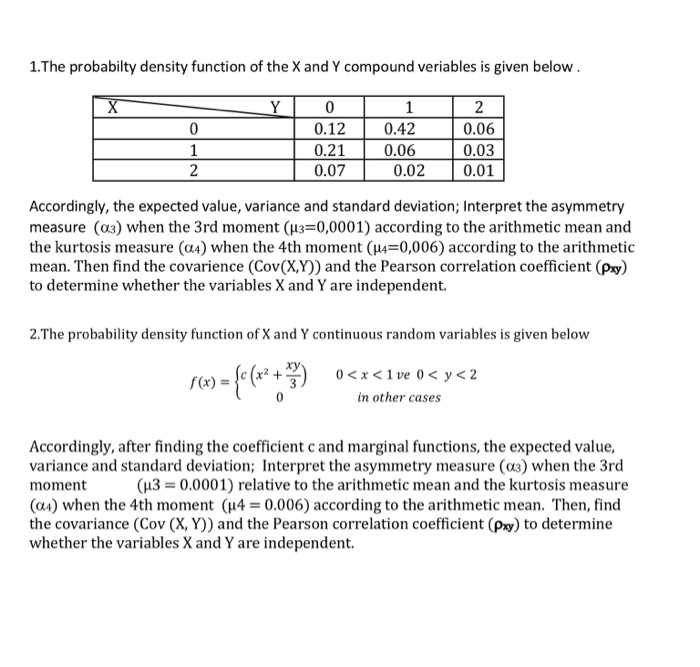
In each situation below, is it reasonable to use a binomial distribution for the random variable X? Give reasons for your answerin each case. {a} A random sample of students in a fitness study. X is the mean systolic blood pressure of the sample. tries, a binomial distnbution is reasonable. X can only take on two values No, a binomial distnbution is not reasonable. X is not a count of successes. No; a binomial tstribution is not reasonable. Binomial distnbutions cannot be used with random samples No, a binomial tstribution is not reasonable. it should be associated with a population \"ms a binomial tstribution is reasonable. X is a mean of the binomial distribution. [bi A manufacturer of running shoes picks a random sample o'fthe production of shoes each day for a detailed inspection. Today's sample of 20 pairs of shoes indudes 1 pairwith a defect. No; a binomial bstribution is not reasonable. Dne defect in a sample of 2B is not a large enough percentage. No, a binomial distribution is not reasonable. Binomial distributions cannot be used with random samples. \"ms a binomial distribution is reasonable. p is the probability of a defective pair. "ms a binomial distribution is reasonable. p is the number of defective shoes from today's sample. No, a binomial distribution is not reasonable. Cine defect is not a large enough count. {c} A nutrition study d'iooses an SR5 of college students Tl'iey are asked whether or not they usually eat at least ve servings of fruits or vegetables per day. X is the nurrberwho saythat they do. \"lies; a binomial distribution is reasonable. n is the number of students chosen from the sample and X is the number of servings of fmits and vegetables they eat. No, a binomial tstribuijon is not reasonable. A student might eat less than ve senaings offnJits and vegetables but could daim othenlvise. Yes, a binomial distnbution is reasonable. p is the percentage of students d'iosen from the population and n is the number of servings of fmits. and vegetables they eat. Yes, a binomial distnbution is reasonable. n is the number of students in the sample and p is the probabilitythat a student eats at least five servings o'ffruits and vegetables No, a binomial tstribution is not reasonable. Binomial distnbutions cannot be used with random samples 1. The probabilty density function of the X and Y compound veriables is given below . X Y 0 1 2 0 0.12 0.42 0.06 0.21 0.06 0.03 0.07 0.02 0.01 Accordingly, the expected value, variance and standard deviation; Interpret the asymmetry measure (03) when the 3rd moment (#3=0,0001) according to the arithmetic mean and the kurtosis measure (04) when the 4th moment (14=0,006) according to the arithmetic mean. Then find the covarience (Cov(X,Y)) and the Pearson correlation coefficient (Pxy) to determine whether the variables X and Y are independent. 2. The probability density function of X and Y continuous random variables is given below (( x) = c(x2 +3 ) O