Question:
Jedi Master Astaal Vibum is interested in studying the midi-clorian count in Jedi younglings and how those levels change as they become padawan and ultimately full Jedi knights. To begin the study, Astaal randomly selected 43 Jedi younglings and measured their midi-clorian counts. Measurements will be taken annually until they leave the Jedi order or reach the level of Jedi Master. What is the population of this study?
Question 1 options:
1)
All Jedi younglings
2)
All Jedi knights
3)
The 43 younglings measured
4)
All children
Question 2 (1 point)
Snoopy Brown is interested in the number of businesses that do not allow dogs in St. Paul, MN. To conduct his study, he randomly selects 100 businesses listed in the St. Paul phone book, calls them and asks their policy. What is the sample of this study?
Question 2 options:
1)
The 100 businesses called
2)
All businesses in St. Paul
3)
All businesses in St. Paul that allow dogs
4)
Any businesses, of the 100 called, that allow dogs
Question 3 (1 point)
Homer Simpson is interested in studying the ratio of the various colors of sprinkles on Lard Lad donuts. To conduct his study, Homer purchases three of each variety of donut they make and counts the sprinkles on them. What method did Homer use to select his sample?
Question 3 options:
1)
Stratified
2)
Simple Random Sample
3)
Systematic
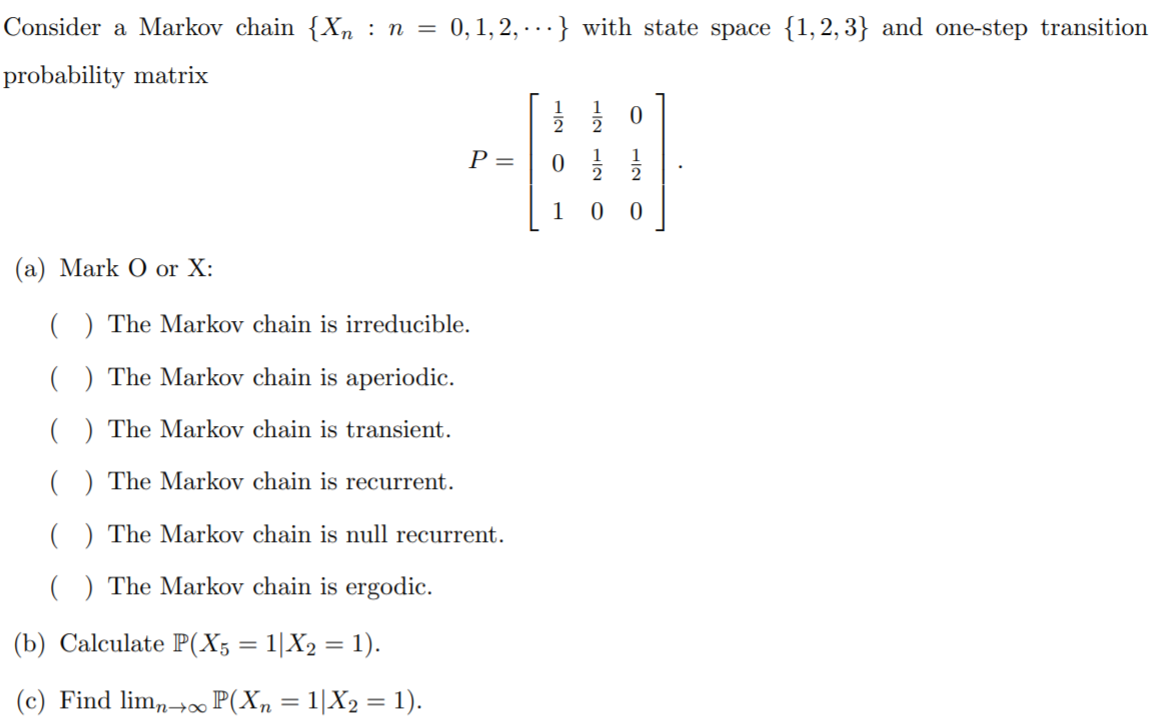
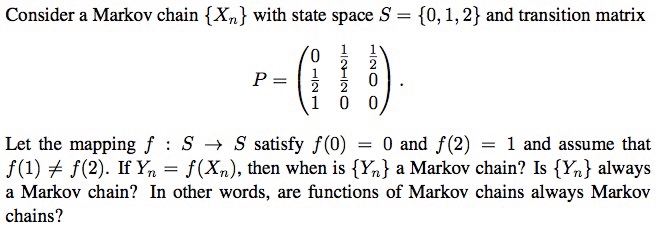
Consider a Markov chain {Xn : n = 0, 1, 2, ...} with state space {1, 2, 3} and one-step transition probability matrix O NIH NIH P = O 0 O (a) Mark O or X: ( ) The Markov chain is irreducible. ( ) The Markov chain is aperiodic. ( ) The Markov chain is transient. ( ) The Markov chain is recurrent. ( ) The Markov chain is null recurrent. ( ) The Markov chain is ergodic. (b) Calculate P(X5 = 1/X2 = 1). (c) Find limn + P(Xn = 1/X2 = 1).Consider a Markov chain { Xn } with state space S' = {0, 1, 2} and transition matrix P = Let the mapping f : S -> S satisfy f(0) = 0 and f(2) = 1 and assume that f(1) # f(2). If Ym = f(X ), then when is { Y } a Markov chain? Is {Ym } always a Markov chain? In other words, are functions of Markov chains always Markov chains?http://keisan.casio.com/exec/system/1180573226 Unlike the normal distribution which is defined over the entire x-axis (that is, under the normal distribution there is no theoretical minimum or maximum the random variable x can take), the beta distribution has a "finite support" (that is, the beta pdf is only defined for x in the interval [0,1]), and it can be use use to define similar "finite support" distributions over any given interval. This means that if you are studying a random variable whose values are bounded above and below, you may be able to fit its histogram using some derivation of the beta distribution. The beta distribution has two "shape" parameters a and b. The following experiments will help you understand how the shape parameters a and b work. FYI, here's an explanation https://www.youtube.com/watch?v=v1uUgTelnQk 1. What is the shape of the beta distribution if a = 0.5 and b = 0.5? 2. What is the shape of the beta distribution if a = 0.5 and b = 1? 3. What is the shape of the beta distribution if a = 1 and b = 1? 4. What is the shape of the beta distribution if a = 3 and b = 3? 5. What is the shape of the beta distribution if a = 30 and b = 30? 6. What is the shape of the beta distribution if a = 300 and b = 300? 7. What is the shape of the beta distribution if a = 3 and b = 20? 8. Find a and b such that the resulting beta distribution is skewed right. 9. On a spreadsheet create a list of forty numbers between 0 and 1 whose histogram follows the shape of the distribution in part 8. (Hint: just as when you generated a perfectly normal distribution a few weeks ago using the normal standard inverse function, here do the same thing; but this time use the beta inverse function "beta.inv(x,a,b)" where x will be the values you get after you divided the interval into 40 equal sized pieces.) 10. Now apply a transformation so that your values are centered around 100 with variance 100; in other words they have mean = 100, and standard deviation =10.Chi Squared and T Distribution[2 points} Let Vn ~ )1; 2 n and Tn ~ tn for all positive integers n. [a] Find numbers an and bn such that aan bn]I converges in distribution to NEIL 1]. [1 point} [b] Show that T 2 n Hn+T 2 n I has a Beta distribution. Specifyr the para meters of the Beta distribution. [1 point] Hirrt: You should be able to do part [b] by using the Beta Gamma connection. 4. Chi Squared and T Distributinn[2 points} Let V" N x: and Tn w in for all positive integers n. {3.} Find numbers an and {In such that an\"; Ian] converges in distribution to N[,1}. [1 point] [b} Show that T3,? [n+ TE} has a. Beta distribution. Specify the parameters of the Beta. distribution. {1 point] Hint: 1tron should be able to do part {b} h}...r using the Beta Gamma connection