Question
Question: Leave X alone an irregular variable with thickness fx(x) = (I/4)x 0 x and let Y = X+ 3. (a) Discover EN, and afterward
Question:
Leave X alone an irregular variable with thickness fx(x) = (I/4)x 0 x and let Y = X+ 3. (a) Discover EN, and afterward utilize the principles for assumption to discover E[Y].
(b) Discover the thickness for Y.
(c) Utilize the thickness for Y to discover Period and contrast your answer with that found to a limited extent (a).
Question 26
Let Xdenote the slack time in a printing line at a specific PC community. That is, X means the distinction between the time that a program is set in the line and the time at which printing starts. Expect that Xis ordinarily appropriated with mean 15 minutes and difference 25.
(a) Discover the articulation for the thickness for X.
(b) Discover the likelihood that a program will arrive at the printer inside 3 minutes of showing up in the line.
(c) Would it be uncommon for a program to remain in the line somewhere in the range of 10 and 20 minutes? Clarify, in view of the rough likelihood of this happening. You don't need to utilize the Z table to respond to this inquiry!
(d) Would you be shocked in the event that it took longer than 30 minutes for the program to arrive at the printer? Clarify, in view of the likelihood of this happening.
Question 27
Let Xdenote the time needed to redesign a PC framework in hours. Expect that the thickness for Xis given by f(x) = k exp (- 2x) 0
(b) Discover the likelihood that it will require all things considered 1 hour to redesign a given framework. (c) Figure out the normal time needed to redesign a framework. (d) Track down the standard deviation in the time needed for the redesign.
Question 28
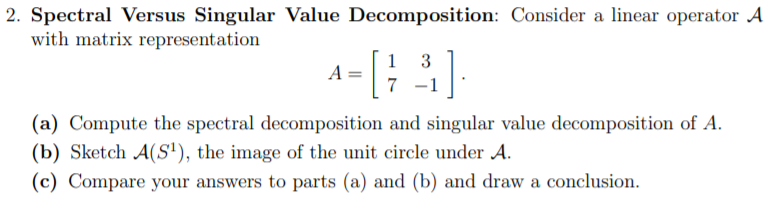
Let Xdenote the opportunity to disappointment in long stretches of a phone modem used to get to a centralized server PC from a far off terminal. Accept that the peril rate work for X is given by P(t) = a$119-1 where a = 2 and 13 = 1/5. (a) Discover the disappointment thickness for X.
(b) Track down the normal estimation of X. (c) Discover the unwavering quality capacity for X.
(d) Discover the likelihood that the modem will keep going for in any event 2 years. (e) What is the risk rate at t = 1 year? (l Depict generally the hypothetical example in the reasons for disappointment in these modems.

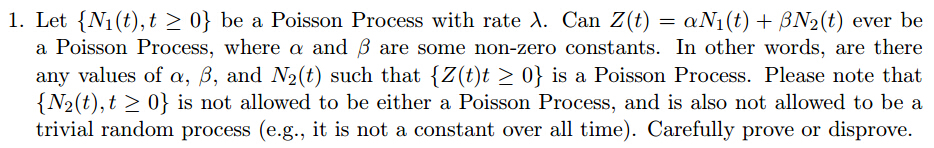

Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


