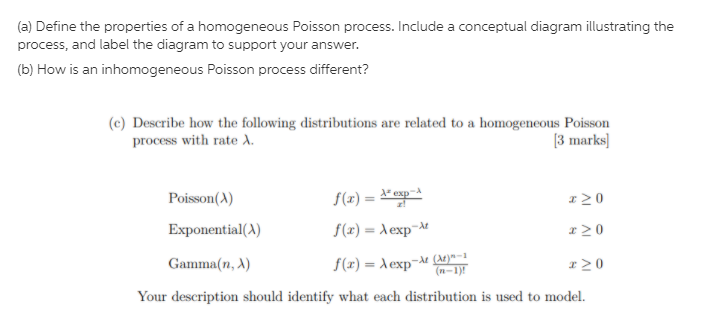
Question:

The management of Madeira Computing is considering the introduction of a wearable electronic device with the functionality of a laptop computer and phone. The fixed cost to launch this new product is $300,000. The variable cost for the product is expected to be between $166 and $246, with a most likely value of $206 per unit. The product will sell for $300 per unit. Demand for the product is expected to range from 0 to approximately 20,000 units, with 4,000 units the most likely.

Model the variable cost as a uniform random variable with a minimum of $166 and a maximum of $246. Model the product demand as 1,000 times the value of a gamma random variable with an alpha parameter of 3 and a beta parameter of 2. Construct a simulation model to estimate the average profit and the probability that the project will result in a loss. (Use at least 1,000 trials.)

a. Develop a what-if spreadsheet model computing profit (in $) for this product in the base-case, worst-case, and best-case scenarios.

b. What is the average profit (in $)? (Round your answer to the nearest thousand.)

c. What is the probability the project will result in a loss? (Round your answer to three decimal places.)

(a) Define the properties of a homogeneous Poisson process. Include a conceptual diagram illustrating the process, and label the diagram to support your answer. (b) How is an inhomogeneous Poisson process different? (c) Describe how the following distributions are related to a homogeneous Poisson process with rate A. [3 marks] Poisson (X) f(x) = exp d Exponential(A) f(x) = Aexp-At 120 Gamma(n, A) f(x) = Aexp-At (At)-1 (n-1)! 120 Your description should identify what each distribution is used to model.4. Distributions associated with Poisson process (14 marks) Prove the following theorem: If W1, W2,..., are the occurrence times in a time-homogeneous Poisson process with. intensity > > 0, then conditioned on N(t) = n the random variables W1, W2. . .., W. have the joint probability density function f (w1. .... Wn) = nit-", for 0 3. The death rates are (1= 3, (2= 4, (3= 1, and Un = 2 for n > 4. A. (5%) Construct the rate diagram for this birth-and-death process. B. (5%) Develop the balance equations. C. (5%) Solve these equations to find the steady-state probability distribution Po, P1, . . . . D. (5%) Use the general formulas for the birth-and-death process to calculate Po, P1, . . . . Also calculate L, La , W , and Wa.Consider a birth-and-death process with just three attainable states (0, 1, and 2), for which the steady-state probabilities are PO, P1, and P2, respectively. The birth-and-death rates are summarized in the following table: state Birth Death rate rate 0 1 1 1 2 2 2 a)Construct the rate diagram for this birth-and-death process. b) Develop the balance equation. c) Solve these equations to find PO, P1, and P2 d) Use the general formulas for the birth-and-death process to calculate PO, P1 and P2.Also calculate L, Lq, W, and W