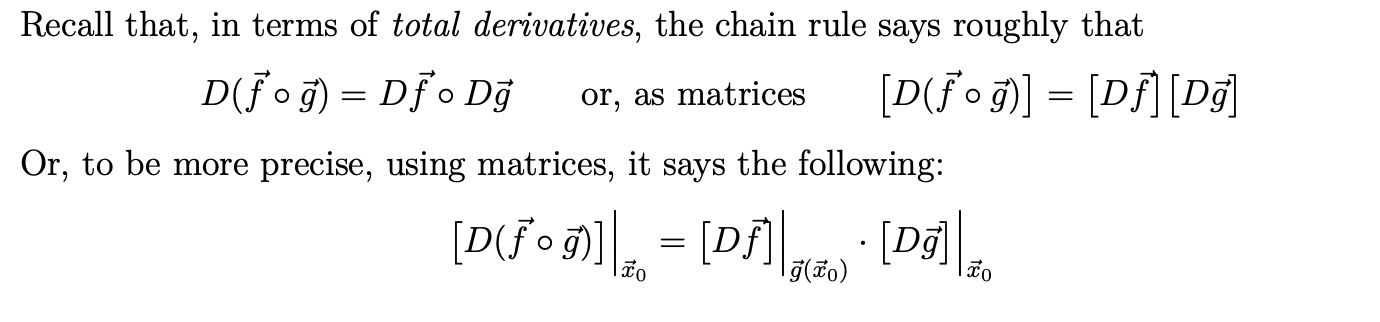
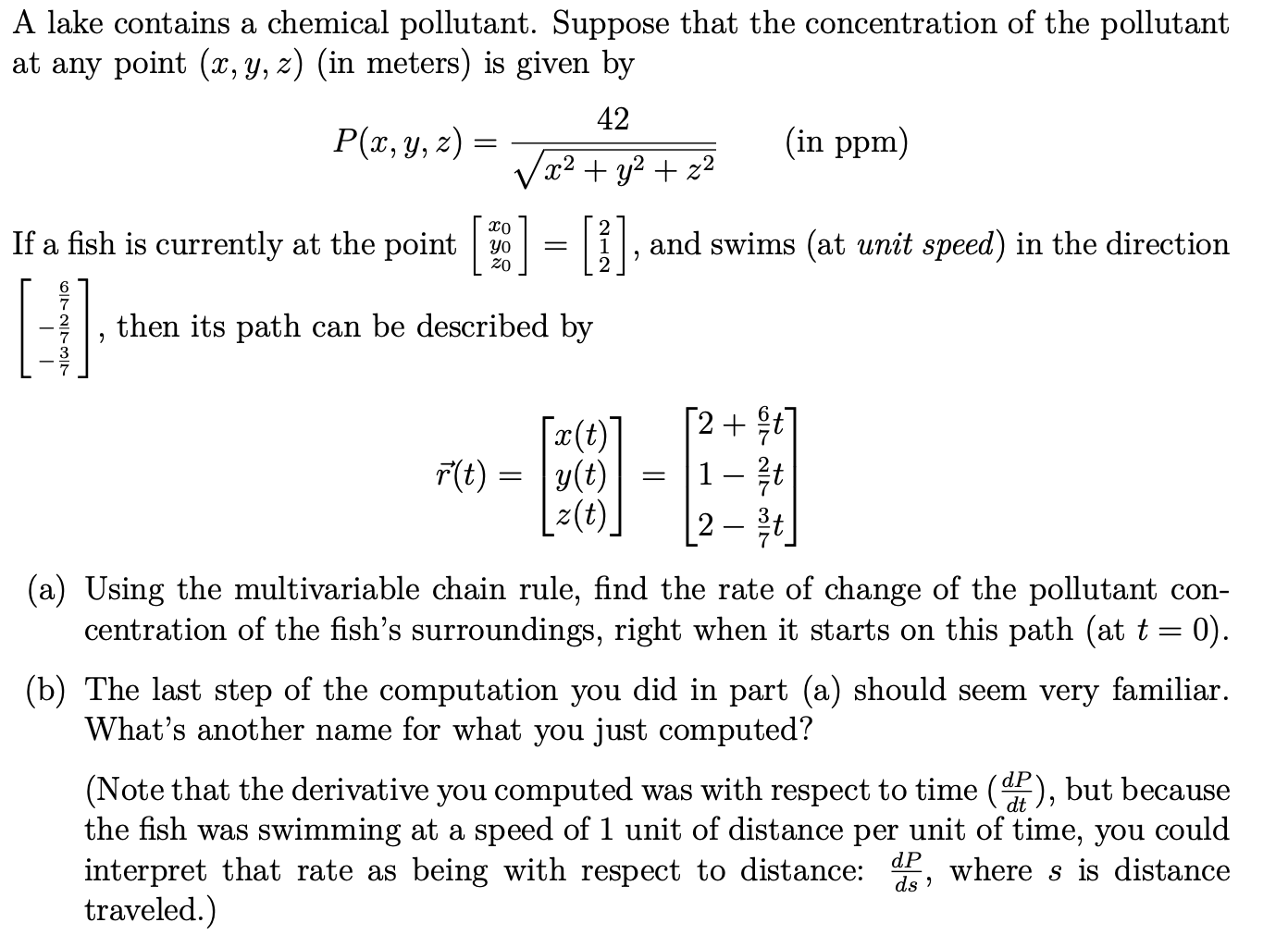
Recall that, in terms of total derivatives, the chain rule says roughly that [D(fog)] = [Df] [Dg] D(fog) = Dfo D or, as matrices Or, to be more precise, using matrices, it says the following: [D(Jag)] = [D] [D] To g(xo) To A lake contains a chemical pollutant. Suppose that the concentration of the pollutant at any point (x, y, z) (in meters) is given by 42 P(x, y, z) = x + y + z (in ppm) If a fish is currently at the point [] = [1], and swims (at unit speed) in the direction (4) then its path can be described by r(t) = [x(t)] y(t) [z(t) = 1 2 + I 67 27 37 4 (a) Using the multivariable chain rule, find the rate of change of the pollutant con- centration of the fish's surroundings, right when it starts on this path (at t = 0). (b) The last step of the computation you did in part (a) should seem very familiar. What's another name for what you just computed? dt (Note that the derivative you computed was with respect to time (d), but because the fish was swimming at a speed of 1 unit of distance per unit of time, you could interpret that rate as being with respect to distance: where s is distance traveled.) dP ds'