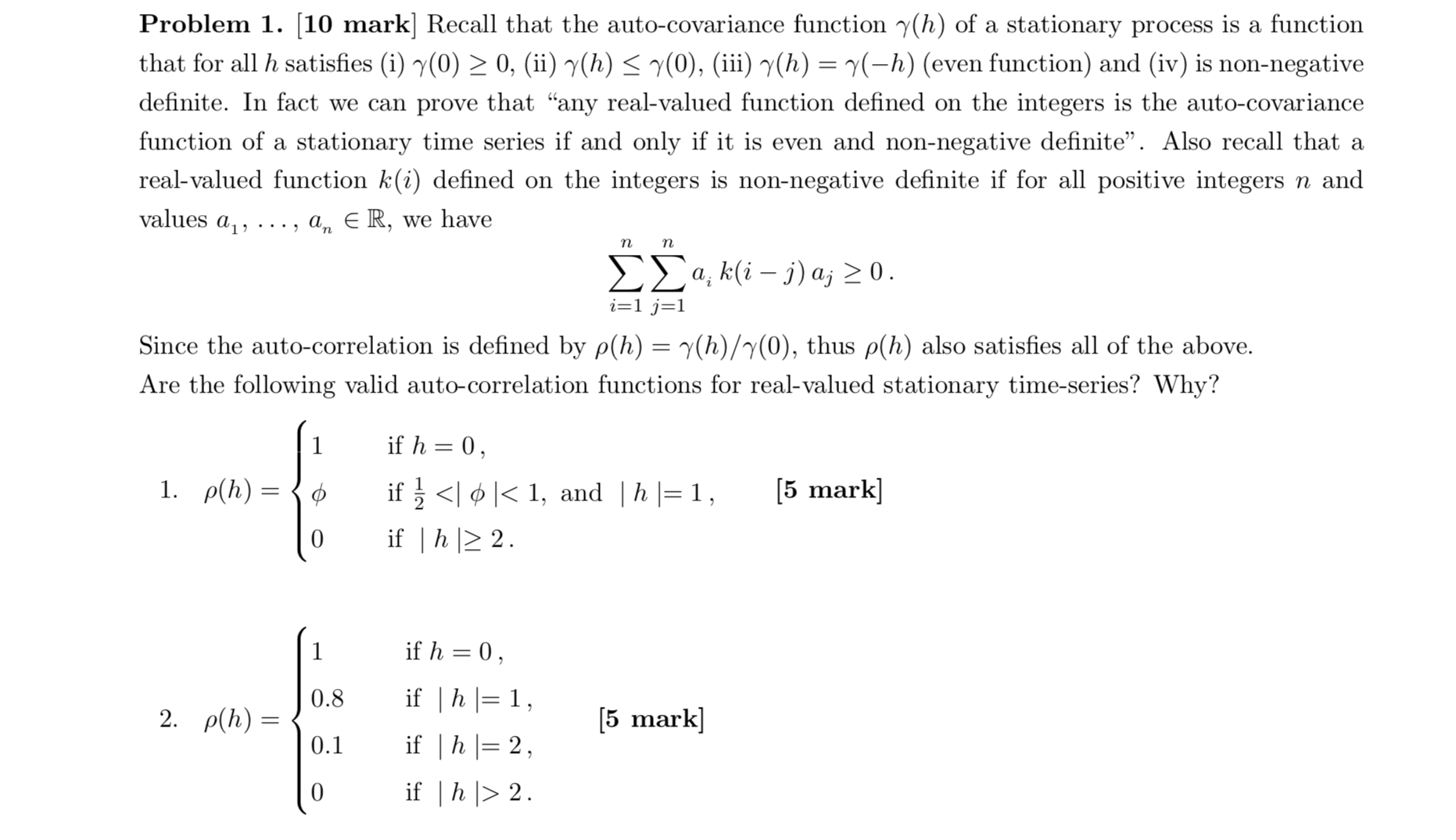
Question
Recall that the auto-covariance function (h) of a stationary process is a function that for all h satisfies (i) (0) 0, (ii) (h) (0), (iii)
Recall that the auto-covariance function (h) of a stationary process is a function that for all h satisfies (i) (0) 0, (ii) (h) (0), (iii) (h) = (h) (even function) and (iv) is non-negative definite. In fact we can prove that "any real-valued function defined on the integers is the auto-covariance function of a stationary time series if and only if it is even and non-negative definite". Also recall that a real-valued function k(i) defined on the integers is non-negative definite if for all positive integers n and values a1, ..., an R, we have
nn
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


