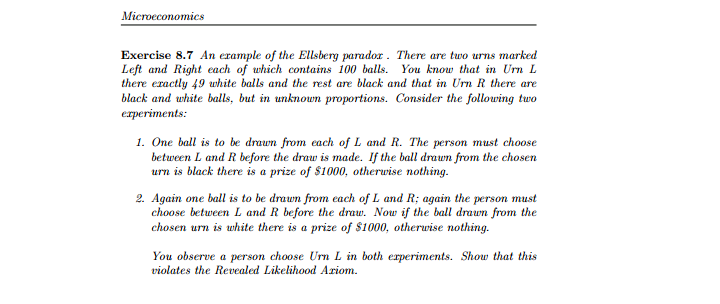
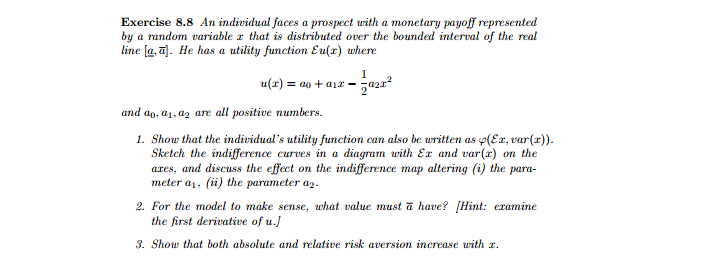
refer to the attachment

Exercise 8.1 Suppose you have to pay $2 for a ticket to enter a competition. The prize is $19 and the probability that you win is . You have an expected utility function with u(x) = logr and your current wealth is $10. 1. What is the certainty equivalent of this competition? 2. What is the risk premium? 3. Should you enter the competition?Microeconomics Exercise 8.3 Consider the following definition of risk aversion. Let P := ((r., 70) : w ( ) be a random prospect, where r. is the payoff in state w and no is the (subjective) probability of state w , and let Er := Even Tory. the mean of the prospect, and let Px := {(Xx, + [1 - AJer, .) : wen} be a "mirture" of the original prospect with the mean. Define an individual as risk averse if he always prefers Px to P for 0 c' > ro. Now consider the following two prospects with probability 0.99 P1: with probability 0 with probability 0.01 with probability 0.99 P. : with probability 0.01 with probability 0 Suppose a person expresses a preference for Pi over Py. Briefly explain why this might be the case in practice. Which of the three arioms State Irrelevance, Independence, Revealed Likelihood, is violated by such preferences?Exercise 8.6 An example to illustrate regret. Let P:= ((IT.) :WEQ} P= ((IT) :WEn} be two prospects available to an individual. Define the expected regret if the person chooses P rather than P' as Ex. max {r' - IN, 0} (8.1) Now consider the choices amongst prospects presented in Exercise 8.4. Show that if a person is concerned to minimise expected regret as measured by (8.1), then it is reasonable that the person select Py when Pi is also available and then also select P. when Pa is available.Microeconomics Exercise 8.7 An example of the Ellsberg paradox . There are two urns marked Left and Right each of which contains 100 balls. You know that in Urn L there exactly 49 white balls and the rest are black and that in Urn R there are black and while balls, but in unknown proportions. Consider the following two experiments: 1. One ball is to be drawn from each of L and R. The person must choose between L and R before the draw is made. If the ball drawn from the chosen urn is black there is a prize of $1090, otherwise nothing. 2. Again one ball is to be drawn from each of L and R; again the person must choose between L and R before the draw. Now if the ball drawn from the chosen urn is while there is a prize of $1090, otherwise nothing. You observe a person choose Urn L in both experiments. Show that this violates the Revealed Likelihood Axiom.Exercise 8.8 An individual faces a prospect with a monetary payoff represented by a random variable r that is distributed over the bounded interval of the real line [a, a]. He has a utility function Eu(x) where 1 u(x) = oo + air - - and ap. a1, a2 are all positive numbers. 1. Show that the individual's utility function can also be written as (Er, var(c)). Sketch the indifference curves in a diagram with Ex and var(x) on the ares, and discuss the effect on the indifference map altering (i) the para- meter an, (u) the parameter a2. 2. For the model to make sense, what value must a have? Hint: examine the first derivative of u.] 3. Show that both absolute and relative risk aversion increase with r