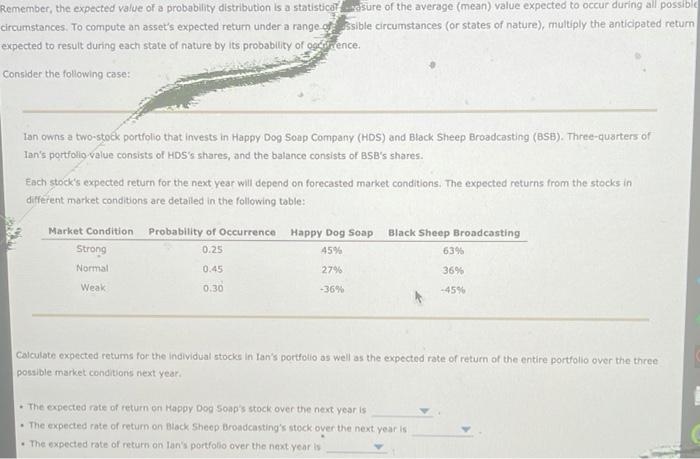
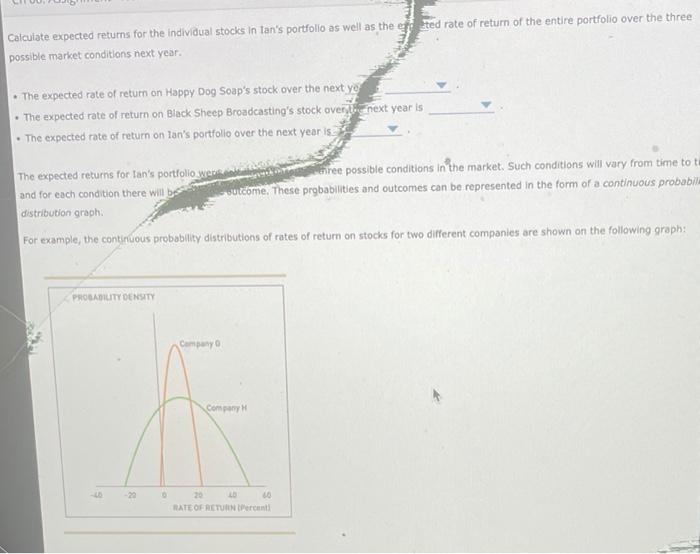
Remember, the expected value of a probability distribution is a statisticatosure of the average (mean) value expected to occur during all possible circumstances. To compute an asset's expected return under a range ossible circumstances (or states of nature), multiply the anticipated return expected to result during each state of nature by its probability of og unence. Consider the following case: tan owns a two-stock portfolio that invests in Happy Dog Soap Company (HDS) and Black Sheep Broadcasting (BSB). Three-quarters of lan's portfolio value consists of HDS's shares, and the balance consists of BSB's shares. Each stoick's expected return for the next year will depend on forecasted market conditions. The expected returns from the stocks in different market conditions are detailed in the following table: Market Condition Probability of Occurrence Strong 0.25 Normal 0.45 Happy Dog Soap 45% Black Sheep Broadcasting 63% 27% 36% Weak 0.30 -36% -459 Calculate expected retums for the individual stocks in Tan's portfolio as well as the expected rate of return of the entire portfolio over the three possible market conditions next year The expected rate of return on Happy Dog Soap's stock over the next year is The expected rate of return on Black Sheep Broadcasting's stock over the next year is The expected rate of return on Tan's portfolio over the next year is Calculate expected returns for the individual stocks in Tan's portfolio as well as the ted rate of return of the entire portfolio over the three possible market conditions next year. The expected rate of return on Happy Dog Soap's stock over the next ye The expected rate of return on Black Sheep Broadcasting's stock over the next year is The expected rate of return on Ian's portfolio over the next year is The expected returns for lan's portfolio were ere possible conditions in the market. Such conditions will vary from time to t and for each condition there will be utcome. These probabilities and outcomes can be represented in the form of a continuous probabil distribution graph For example, the continuous probability distributions of rates of return on stocks for two different companies are shown on the following graph: PROBADILITY DENSITY Campy Company 05 -22 20 LD 0 BATE OF RETURN Percent Based on the graph's information, which company's returns exhibit the greater risk Company H Company G Grad