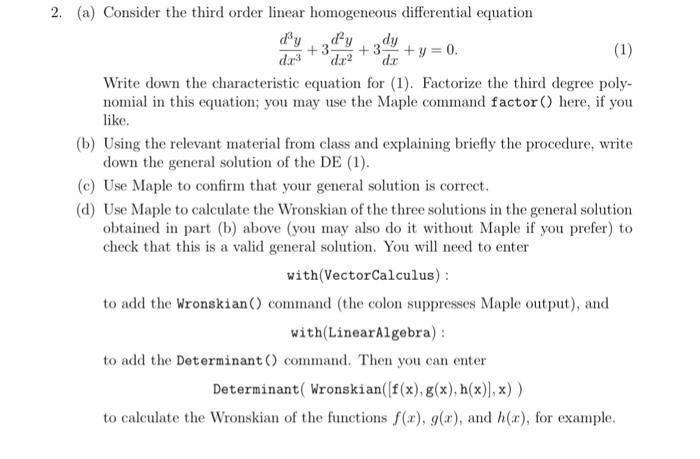
2. (a) Consider the third order linear homogeneous differential equation + y = 0. +3- dx dx da (1) Write down the characteristic equation for (1). Factorize the third degree poly- nomial in this equation; you may use the Maple command factor () here, if you like. (b) Using the relevant material from class and explaining briefly the procedure, write down the general solution of the DE (1). (c) Use Maple to confirm that your general solution is correct. (d) Use Maple to calculate the Wronskian of the three solutions in the general solution obtained in part (b) above (you may also do it without Maple if you prefer) to check that this is a valid general solution. You will need to enter with (Vector Calculus): to add the Wronskian () command (the colon suppresses Maple output), and with (Linear Algebra): to add the Determinant () command. Then you can enter Determinant(Wronskian([f(x), g(x), h(x)], x)) to calculate the Wronskian of the functions f(x), g(x), and h(r), for example. 2. (a) Consider the third order linear homogeneous differential equation + y = 0. +3- dx dx da (1) Write down the characteristic equation for (1). Factorize the third degree poly- nomial in this equation; you may use the Maple command factor () here, if you like. (b) Using the relevant material from class and explaining briefly the procedure, write down the general solution of the DE (1). (c) Use Maple to confirm that your general solution is correct. (d) Use Maple to calculate the Wronskian of the three solutions in the general solution obtained in part (b) above (you may also do it without Maple if you prefer) to check that this is a valid general solution. You will need to enter with (Vector Calculus): to add the Wronskian () command (the colon suppresses Maple output), and with (Linear Algebra): to add the Determinant () command. Then you can enter Determinant(Wronskian([f(x), g(x), h(x)], x)) to calculate the Wronskian of the functions f(x), g(x), and h(r), for example.