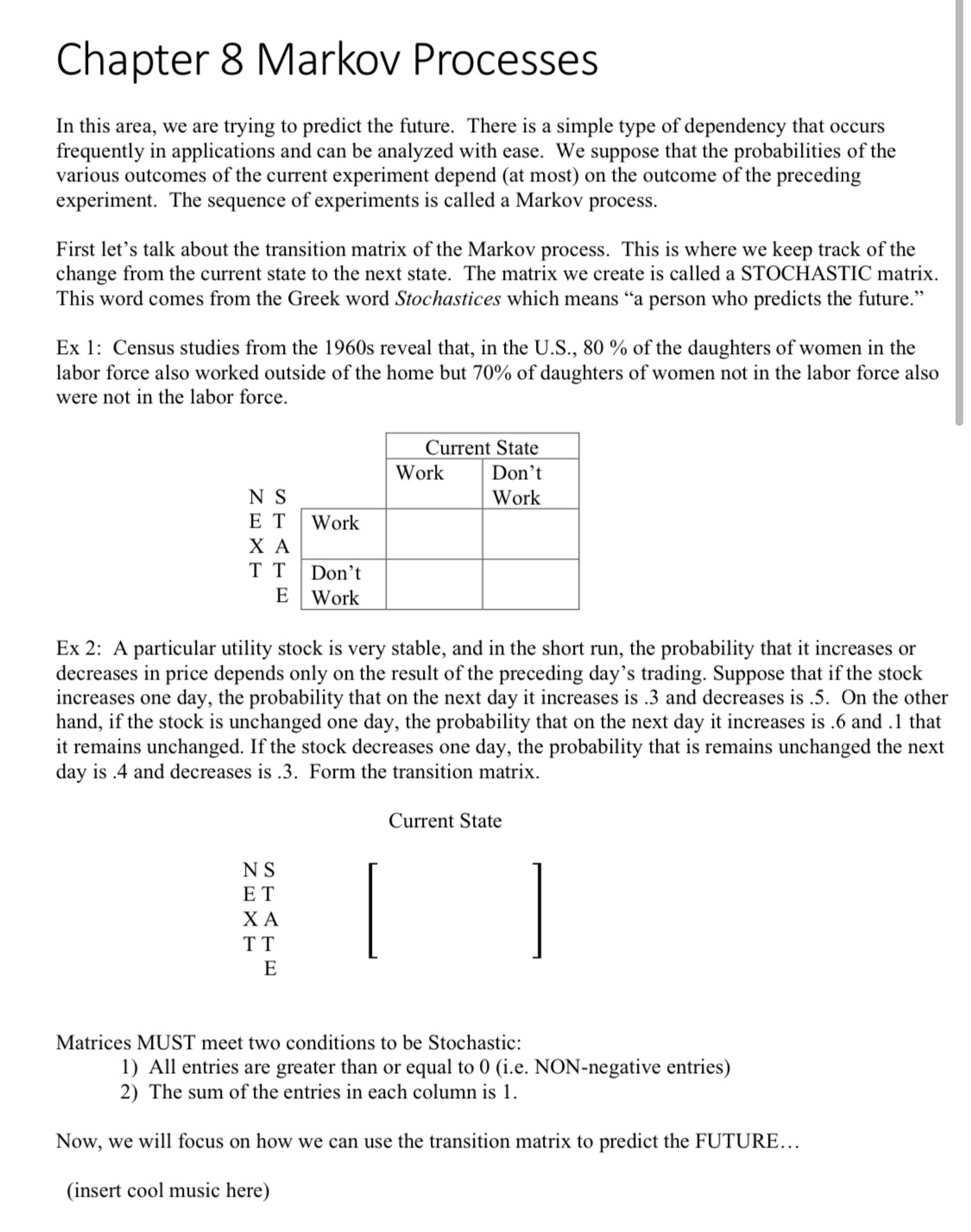
Return to the first example. Assume that the trend remains unchanged from one generation to the next. In 1960, about 40% of US. women worked outside the home. This is the distribution matrix at generation zero. We use a column matrix to describe this information. [' 2] Be sure the order matches the transition; work then no work. To determine how many women will work outside of the home for the next generation, we use Transition times Distribution (Gen 0) which equals Distribution (Gen 1) This can we repeated for each generation. This leads to a formula: A"[ ]o=[ ]n where n is the number of generations alter the initial distribution (the current state). Example 1 Continued: Thus of the women will work after one generation and of the American women will be working aer two generations. Example 2 Continued: Determine the state of the utilities after 2 days, if the initial distribution shows .2 increases, .3 unchanged, and .5 decreases. Chapter 8 Markov Processes In this area, we are trying to predict the future. There is a simple type of dependency that occurs frequently in applications and can be analyzed with ease. We suppose that the probabilities of the various outcomes of the current experiment depend (at most) on the outcome of the preceding experiment. The sequence of experiments is called a Markov process. First let's talk about the transition matrix of the Markov process. This is where we keep track of the change from the current state to the next state. The matrix we create is called a STOCHASTIC matrix. This word comes from the Greek word Stochastices which means \"a person who predicts the future.\" Ex 1: Census studies from the 1960s reveal that, in the U.S., 30 % of the daughters of women in the labor force also worked outside of the home but 70% of daughters of women not in the labor force also were not in the labor force. Current State Ex 2: A particular utility stock is very stable, and in the short run, the probability that it increases or decreases in price depends only on the result of the preceding day's trading. Suppose that if the stock increases one day, the probability that on the next day it increases is .3 and decreases is .5. On the other hand, if the stock is unchanged one day, the probability that on the next day it increases is .6 and .1 that it remains unchanged. If the stock decreases one day, the probability that is remains unchanged the next day is .4 and decreases is .3. Form the transition matrix. Current State NS ET XA TT Matrices MUST meet two conditions to be Stochastic: 1) All entries are greater than or equal to 0 (i.e. NON-negative entries) 2) The sum of the entries in each column is 1. Now, we will focus on how we can use the transition matrix to predict the FUTURE... (insert cool music here)