
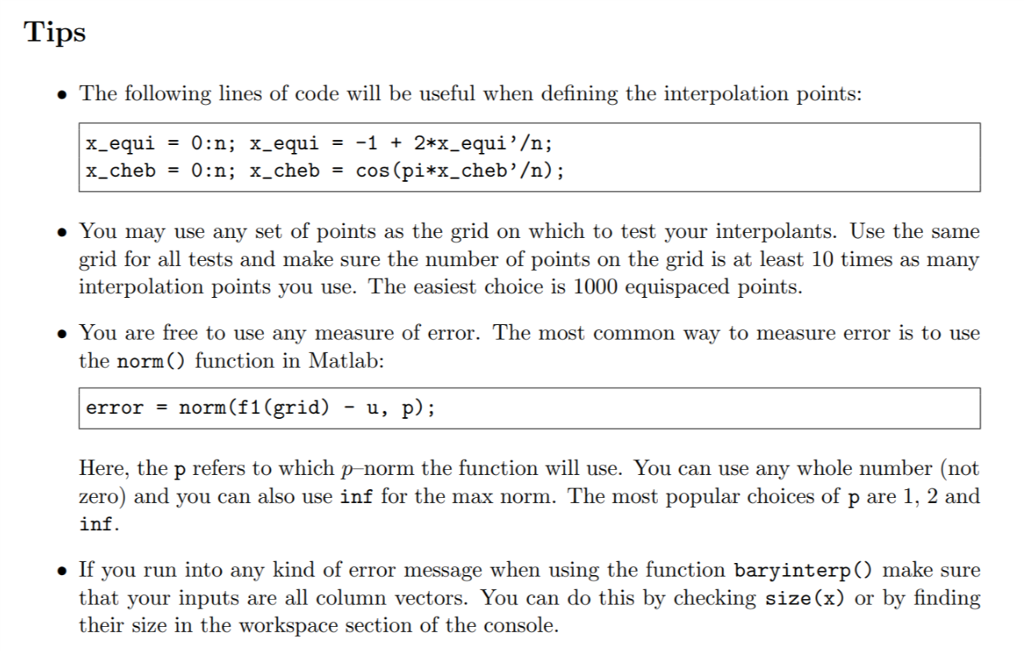

Runge's phenomenon and aliasing Two major concerns in polynomial interpolation are Runge's phenomenon and aliasing. Runge's phenomenon describes oscillations of the interpolant near the boundaries. Aliasing refers to lower degree polynomials pretending to be higher degree polynomials. In this report you will investigate the effects of both issues First, define a set of equispaced points on the interval-1,1: 2i Next, define the set of Chebyshev points on the same interval ri=cos(n), i=0, , n These two sets will act as your interpolation points. In addition, you'll need a set of points to test your interpolants; this set should also span the interval [-1,1] but have several more points than your interpolation points. Download the file baryinterp.m, which uses barycentric Lagrange interpolation to construct the Lagrange interpolating polynomial. All inputs are column vectors. Let x be the interpolation points, f the values of the function to interpolate and grid the points to evaluate the interpolant at. The syntax to use baryinterp) is then u-baryinterp(x,f,grid); Measure the error of interpolation for the function for n between 1 and 100. How does Runge's phenomenon affect the accuracy? Which set of interpolation points deals with it better? Next, measure the error of interpolation for the function /2(x)-cos(10??), f2-0(x) cos (10*pi*x); for n between 1 and 100. How many points do you need to overcome aliasing? Were there any differences between the types of interpolation points? Runge's phenomenon and aliasing Two major concerns in polynomial interpolation are Runge's phenomenon and aliasing. Runge's phenomenon describes oscillations of the interpolant near the boundaries. Aliasing refers to lower degree polynomials pretending to be higher degree polynomials. In this report you will investigate the effects of both issues First, define a set of equispaced points on the interval-1,1: 2i Next, define the set of Chebyshev points on the same interval ri=cos(n), i=0, , n These two sets will act as your interpolation points. In addition, you'll need a set of points to test your interpolants; this set should also span the interval [-1,1] but have several more points than your interpolation points. Download the file baryinterp.m, which uses barycentric Lagrange interpolation to construct the Lagrange interpolating polynomial. All inputs are column vectors. Let x be the interpolation points, f the values of the function to interpolate and grid the points to evaluate the interpolant at. The syntax to use baryinterp) is then u-baryinterp(x,f,grid); Measure the error of interpolation for the function for n between 1 and 100. How does Runge's phenomenon affect the accuracy? Which set of interpolation points deals with it better? Next, measure the error of interpolation for the function /2(x)-cos(10??), f2-0(x) cos (10*pi*x); for n between 1 and 100. How many points do you need to overcome aliasing? Were there any differences between the types of interpolation points









